x-t equation of a particle in SHM is given as x=1.0sin(12πt) in SI units. Potential energy at mean position is zero. Mass of particle is 14 kg. Match the following table (SI units).
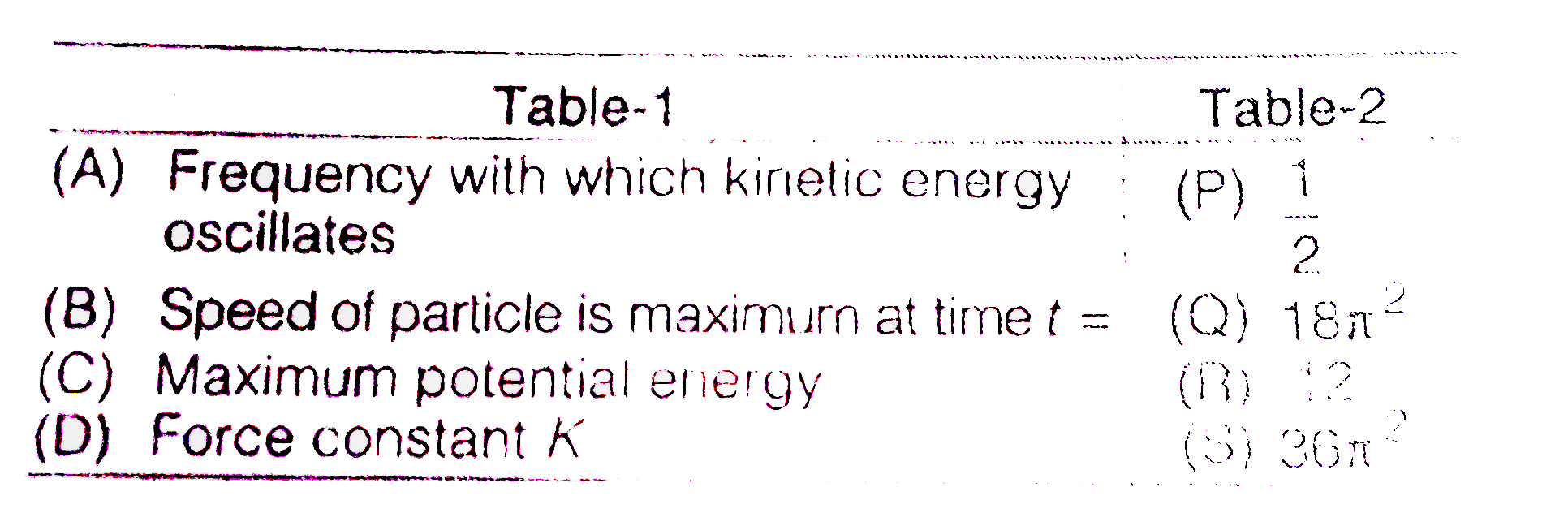
The correct Answer is:A, B, C, D
Step by step video, text & image solution for x-t equation of a particle in SHM is given as x=1.0sin(12pit) in SI units. Potential energy at mean position is zero. Mass of particle is 1/4 kg. Match the following table (SI units). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:Integer type questions
Explore 14 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:Comprehension types
Explore 18 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBook:DC PANDEYChapter:SEMICONDUCTORS AND ELECTRONIC DEVICESExercise:More than One Option is Correct
Explore 3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBook:DC PANDEYChapter:SOLVD PAPERS 2017 NEET, AIIMS & JIPMERExercise:Solved paper 2018(JIPMER)
Explore 38 Videos
Similar Questions
Velocity-time equation of a particle moving in a straight line is, v=(10+2t+3t2) (SI units) Find
(a) displacement of particle from the mean position at time t=1s, if it is given that displacement is 20m at time t=0.
(b) acceleration-time equation.
View SolutionThe displacement of a particle in SHM is x=3sin(20πt)+4cos(20πt)cm. Its amplitude of oscillation is
View Solution
Knowledge Check
Assertion : x-t equation of a particle in SHM is given as :x=Acosωt
Reason : In the given equation the minimum potential energy is zero.AIf both Assertion and Reason are correct and Reason is the correct explanation of Assertion.BIf both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.CIf Assertion is true but Reason is false.DIf Assertion is false but Reason is true.In SHM , potential energy of a particle at mean position is E1 and kinetic enregy is E2 , then
AE1=E2Btotal potential energy at x=√3A2 is E1+3E24Ctotal kinetic energy at x=√3A2 is 3E24Dtotal kinetic energy at x=A√2 is E24A particle of mass 1 kg executing S.H.M. is given by y = 2 cos (10t+π/3) in SI units. Its maximum potential energy is
A2 JB20 JC200 JD100 J
Displacement-time equation of a particle moving along x-axis is x=20+t3−12t (SI units)
(a) Find, position and velocity of particle at time t=0.
(b) State whether the motion is uniformly accelerated or not.
(c) Find position of particle when velocity of particle is zero.
View SolutionThe equation for the displacement of a particle executing SHM is x=5sin(2πt)cm Then the velocity at 3cm from the mean position is( in cm//s)
View SolutionThe displacement of a particle executing SHM is given by
Y=5 sin (4t+π3)
If T is the time period and the mass of the particle is 2g, the kinetic energy of the particle When t=T4 is given by-
View SolutionThe equation of a longitudinal wave is given by y=10sin2π(50t+5022x) (in S.I units). Find the value of x at which change in pressure is maximum at t=0.
View SolutionThe displacement of a particle of mass 3g executing simple harmonic motion is given by x=3sin(0.2t) in SI units. The kinetic energy of the particle at a point which is at a displacement equal to 1/3 of its amplitude from its mean position is
View Solution