You are given a rod of length L. The linear mass density is $λ$ such that $λ = a + b x$ . Here a and b are constants and the mass of the rod increases as x decreases. Find the mass of the rod

Video Solution

Text Solution

Verified by Experts

We are not calculated the mass of the rod simply multiplying linear mass density $λ$ will the length of the rod as $λ$ is not constant.

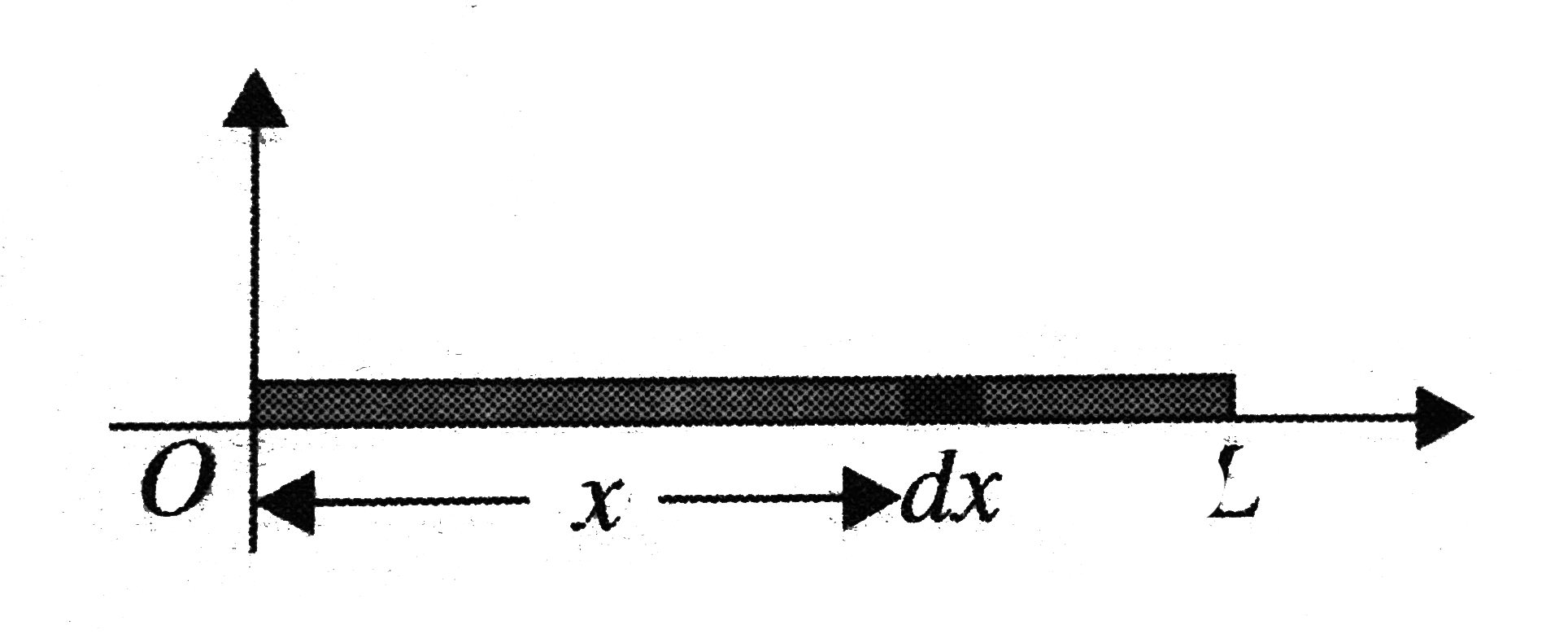
Hence, we have to divide entire rod length into a number of elements and add the mass of the elements.
$M = d m_{1} + d m_{2} + d m_{3} + ...$
This step can be written in terms of integration.
$M = \int d m$
If we take an element $d x$ on the rod at a distance $x$ from the left end of the rod.

Mass of this element $d m = λ . d x$
$d m = (a + b x) d x$
Hence, total mass,
$M = \int d m = L \int 0 (a + b x) d x$
$= L \int 0 a d x + L \int 0 b x d x$
$= a L \int 0 d x + b L \int 0 x d x$
$= a {[x]}_{0}^{L} + b {[\frac{x^{2}}{2}]}_{0}^{L} = a L + \frac{b L^{2}}{2}$

The linear density of a non $-$ uniform rod of length $2 m$ is given by $λ (x) = a (1 + b x^{2})$ where $a$ and $b$ are constants and $0 \leq x \leq 2$ . The centre of mass of the rod will be at. $X =$
A $\frac{3 + 6 b}{6 + 8 b}$
B $\frac{6 + 2 b}{3 + 4 b}$
C $\frac{3 + 6 b}{3 + 4 b}$
D $\frac{1 + 2 b}{3 + 4 b}$
The density of a non-uniform rod of length 1m is given by $ρ (x) = a (1 + b x^{2})$ where, a and b are constant and $0 \leq x \leq 1$ . The centre of mass of the rod will be at
A $\frac{3 (2 + b)}{4 (3 b + b)}$
B $\frac{4 (2 + b)}{3 (3 + b)}$
C $\frac{3 (3 + b)}{4 (2 + b)}$
D $\frac{4 (3 + b)}{3 (2 b + b)}$
The density of a non-uniform rod of length 1 m is given by $ρ (x) = a (1 + b x^{2})$ where a and b are constant and $0 \leq x \leq 1$ .
The centre of mass of the rod will be
A $\frac{3 (2 + b)}{4 (3 + b)}$
B $\frac{4 (2 + b)}{3 (3 + b)}$
C $\frac{3 (3 + b)}{4 (2 + b)}$
D $\frac{4 (3 + b)}{3 (2 + b)}$