यदि दो समरूप त्रिभुजों के क्षेत्रफल बराबर हो तो सिद्ध कीजिए कि वे त्रिभुज सर्वांगसम होते है
Step by step video & image solution for यदि दो समरूप त्रिभुजों के क्षेत्रफल बराबर हो तो सिद्ध कीजिए कि वे त्रिभुज सर्वांगसम होते है by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Similar Questions
The areas of two similar triangles are 324cm2 and 225cm2 . If the altitude of the smaller triangle is 10 cm, then the altitude of the bigger triangle in centimetres, is:
दो समान त्रिभुजों के क्षेत्र 324cm2 और 225cm2 है। यदि छोटे त्रिभुज की ऊंचाई 10 सेमी है, तो सेंटीमीटर में बड़े की ऊंचाई है:View SolutionIf the area of an equilateral triangle is 36√3cm2, then the perimeter of the triangle is:
यदि एक समबाहु त्रिभुज का क्षेत्रफल 36√3 वर्ग सेमी है, तो इस त्रिभुज का परिमाप कितना होगा ?View Solution
Knowledge Check
- Question 1 - Select One
If each angle of a triangle is less than the sum of the other two, then the triangle is
Aअधिक कोणBसमकोणCन्यून कोणDसमभुज
In triangle ABC AD, BE, CF are medians. If the area of the triangle DGC is 20cm2 , then the area of triangle AGF + the area of triangle BGF is equal to:
त्रिभुज ABC में, AD, BE और CF मध्यरेखा हैं और G त्रिभुज का केन्द्रक है। यदि त्रिभुज DGC का क्षेत्रफल 20cm2 है, तो त्रिभुज AGF का क्षेत्रफल+ त्रिभुज BGF का क्षेत्रफल किसके बराबर है:View Solution△ABC is similar to △DEF. The area of △ABC is 100cm2 and the area of △DEF is 49cm2. If the altitude of △ABC=5cm, then the corresponding altitude of △DEF is:
त्रिभुज ABC त्रिभुज DEF के समरूप है | त्रिभुज ABC का क्षेत्रफल 100 वर्ग सेमी है तथा त्रिभुज DEF का क्षेत्रफल 49 वर्ग सेमी है | यदि त्रिभुज ABC की ऊंचाई - 5 सेमी है, तो त्रिभुज DEF की संगत ऊंचाई ज्ञात करें |View SolutionIn the given figure, E and F are the centers, of two identical circles. What is the ratio of area of triangle, AOB to the area of triangle DOC?
दी गई आकृति में, E तथा F दो समरूप वृत्तों के केन्द्र है। त्रिभुज AOB के क्षेत्रफल का त्रिभुज DOC के क्षेत्रफल से क्या अनुपात है?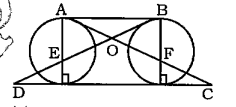
View SolutionIn a △ABC, D and E are two points on the sides AB and BC , respectively such that AD : DB = 2: 3 and DE || AC. If the area of △ADE is equal to 18 square cm, then what is the area ( In square cm) of △ABC ?
किसी त्रिभुज ABC में, D और E क्रमश: भुजा AB और BC पर स्थित बिंदु हैं जो इस प्रकार हैं कि AD : DB = 2: 3 है तथा 9DE || AC है | यदि त्रिभुज ADE का क्षेत्रफल 18 वर्ग सेमी के बराबर है, तो त्रिभुज ABC का क्षेत्रफल ( वर्ग सेमी में ) ज्ञात करेंView SolutionA triangle has sides 25, 39 and 34 units. If the area of a square exceeds the area of this triangle by 21 units, then the side of the square is:
एक त्रिभुज की भुजाएँ 25, 39 और 34 इकाई हैं। यदि एक वर्ग का क्षेत्रफल इस त्रिभुज के क्षेत्रफल से 21 इकाई अधिक है तो वर्ग की भुजा ज्ञात कीजिए।View SolutionThe diagonal of a square is equal to the side of an equilateral triangle. If the area of the square is 18√3 sq.cm. What is the area (in cm2) of the equilateral triangle?
किसी वर्ग का विकर्ण एक समबाहु त्रिभुज की भुजा के बराबर है | यदि वर्ग का क्षेत्रफल 18√3 वर्ग सेमी है, तो समबाहु त्रिभुज का क्षेत्रफल ( वर्ग सेमी में ) ज्ञात करें |View SolutionABC and BDE are two equilateral triangles such that D is the mid-point of BC. If the area of triangle ABC is 136cm2, then the area of triangle BDE is equal to:
ABC और BDE दो समबाहु त्रिभुज हैं जेसे D, BC का मध्य-बिंदु है। यदि त्रिभुज ABC का क्षेत्रफल 136cm2, है, तो त्रिभुज BDE का क्षेत्रफल ज्ञात करेView Solution