△ABC is similar to △DEF. The area of △ABC is 100cm2 and the area of △DEF is 49cm2. If the altitude of △ABC=5cm, then the corresponding altitude of △DEF is:
त्रिभुज ABC त्रिभुज DEF के समरूप है | त्रिभुज ABC का क्षेत्रफल 100 वर्ग सेमी है तथा त्रिभुज DEF का क्षेत्रफल 49 वर्ग सेमी है | यदि त्रिभुज ABC की ऊंचाई - 5 सेमी है, तो त्रिभुज DEF की संगत ऊंचाई ज्ञात करें |
7 cm
4.5 cm
6 cm
3.5 cm
To find the corresponding altitude of triangle DEF, we can use the properties of similar triangles and the relationship between their areas and corresponding altitudes.
1. Understanding Similar Triangles:
Since triangle ABC is similar to triangle DEF, the ratio of their areas is equal to the square of the ratio of their corresponding altitudes.
2. Setting Up the Ratios:
Let the altitude of triangle DEF be denoted as h2. We know:
- Area of triangle ABC =100cm2
- Area of triangle DEF =49cm2
- Altitude of triangle ABC =5cm
The relationship between the areas can be expressed as:
Area of ABCArea of DEF=(h1h2)2
where h1=5cm (altitude of triangle ABC).
3. Substituting the Values:
Substitute the known values into the equation:
10049=(5h2)2
4. Cross-Multiplying:
Cross-multiply to eliminate the fraction:
100⋅h22=49⋅25
Simplifying the right side:
100⋅h22=1225
5. Solving for h22:
Divide both sides by 100:
h22=1225100=12.25
6. Finding h2:
Take the square root of both sides to find h2:
h2=√12.25=3.5cm
Thus, the corresponding altitude of triangle DEF is 3.5 cm.
Since triangle ABC is similar to triangle DEF, the ratio of their areas is equal to the square of the ratio of their corresponding altitudes.
Let the altitude of triangle DEF be denoted as h2. We know:
- Area of triangle ABC =100cm2
- Area of triangle DEF =49cm2
- Altitude of triangle ABC =5cm
Area of ABCArea of DEF=(h1h2)2
where h1=5cm (altitude of triangle ABC).
Substitute the known values into the equation:
10049=(5h2)2
Cross-multiply to eliminate the fraction:
100⋅h22=49⋅25
Simplifying the right side:
100⋅h22=1225
Divide both sides by 100:
h22=1225100=12.25
Take the square root of both sides to find h2:
h2=√12.25=3.5cm
Topper's Solved these Questions
Similar Questions
Knowledge Check
If △ABC≅△DEF then
AAC=DEBBC=DFCFE=CBDAB=DFΔABC is similar to ΔDEF. If the ratio of similar sides is k:1, the ratio of their areas is
Ak2:1B2k:1Ck22:1D2k2:1
△ABC is similar to △DEF. If area of △ABC is 9 sq. cm. and area of △DEF is 16 sq. cm. and BC=2.1 cm. Then the length of EF will be
△ABC,triangle DEF के समरूप है। यदि triangle ABC का क्षेत्रफल 9 वर्ग से.मी. हो और triangle DEF का क्षेत्रफल 16 वर्ग से.मी. हो ओर BC = 2.1 से.मी. हो तो EF की लम्बाई बताइए?
View SolutionIn triangle ABC AD, BE, CF are medians. If the area of the triangle DGC is 20cm2 , then the area of triangle AGF + the area of triangle BGF is equal to:
त्रिभुज ABC में, AD, BE और CF मध्यरेखा हैं और G त्रिभुज का केन्द्रक है। यदि त्रिभुज DGC का क्षेत्रफल 20cm2 है, तो त्रिभुज AGF का क्षेत्रफल+ त्रिभुज BGF का क्षेत्रफल किसके बराबर है:
View SolutionABC and BDE are two equilateral triangles such that D is the mid-point of BC. If the area of triangle ABC is 136cm2, then the area of triangle BDE is equal to:
ABC और BDE दो समबाहु त्रिभुज हैं जेसे D, BC का मध्य-बिंदु है। यदि त्रिभुज ABC का क्षेत्रफल 136cm2, है, तो त्रिभुज BDE का क्षेत्रफल ज्ञात करे
View SolutionIn △ABC, MN||BC, the area of quadrilateral MBCN=130 sqcm. If AN : NC = 4:5, then the area of △MAN is:
त्रिभुज ABC में, MN||BC है तथा चतुर्भुज MBCN का क्षेत्रफल 130 वर्ग सेमी है | यदि AN : NC = 4 : 5 है, तो त्रिभुज MAN का क्षेत्रफल ज्ञात करें |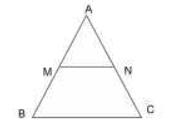
View SolutionIf the area of an equilateral triangle is 36√3cm2, then the perimeter of the triangle is:
यदि एक समबाहु त्रिभुज का क्षेत्रफल 36√3 वर्ग सेमी है, तो इस त्रिभुज का परिमाप कितना होगा ?
View SolutionIf the height of an equilateral triangle is 12 cm, then what is the area of the triangle?
यदि एक समबाह त्रिभुज की ऊंचाई 12 सेमी है, तो त्रिभुज का क्षेत्रफल कितना है?
View Solution