A 25 foot ladder is placed against the side of a building at an angle of 70∘ from the ground. How far away is the base of the ladder from the building?
25cos70∘
8.5sin70∘
25tan70∘
8.5cos70∘
The correct Answer is:A
To solve the problem, we can use trigonometric relationships in a right triangle formed by the ladder, the wall of the building, and the ground. Here are the steps to find how far away the base of the ladder is from the building:
Step 1: Identify the components of the triangle
We have a right triangle where:
- The ladder acts as the hypotenuse (25 feet).
- The angle between the ground and the ladder is 70∘.
- We need to find the base of the ladder from the building, which we will denote as x.
Step 2: Use the cosine function
In a right triangle, the cosine of an angle is defined as the ratio of the length of the adjacent side (base) to the length of the hypotenuse. Thus, we can write:
cos(70∘)=x25
Step 3: Rearrange the equation to solve for x
To find x, we can rearrange the equation:
x=25⋅cos(70∘)
Step 4: Calculate x
Now, we will calculate x using the cosine value:
x=25⋅cos(70∘)
Using a calculator, we find:
cos(70∘)≈0.3420
Thus,
x≈25⋅0.3420≈8.55 feet
Final Answer
The distance from the base of the ladder to the building is approximately 8.55 feet.
---
We have a right triangle where:
- The ladder acts as the hypotenuse (25 feet).
- The angle between the ground and the ladder is 70∘.
- We need to find the base of the ladder from the building, which we will denote as x.
In a right triangle, the cosine of an angle is defined as the ratio of the length of the adjacent side (base) to the length of the hypotenuse. Thus, we can write:
cos(70∘)=x25
To find x, we can rearrange the equation:
x=25⋅cos(70∘)
Now, we will calculate x using the cosine value:
x=25⋅cos(70∘)
Using a calculator, we find:
cos(70∘)≈0.3420
Thus,
x≈25⋅0.3420≈8.55 feet
The distance from the base of the ladder to the building is approximately 8.55 feet.
Topper's Solved these Questions
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #6
3 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #7
3 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #4
3 Videos
- ADVANCED ARITHMETICBOOK - PRINCETONCHAPTER - ADVANCED ARITHMETICEXERCISE - Examples
25 Videos
Similar Questions
Knowledge Check
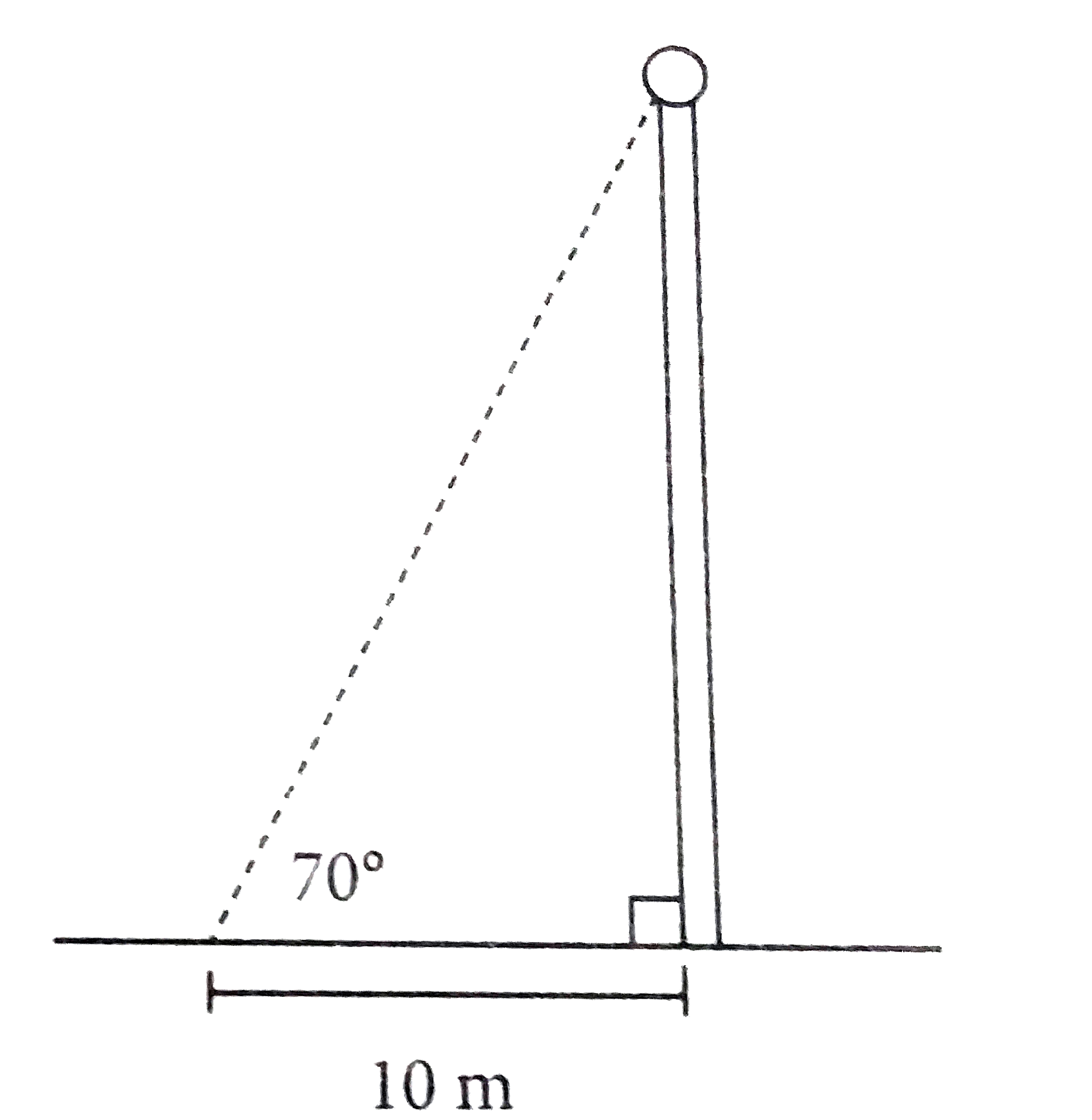
Martin wants to know how tall a certain flagpole is. Martin walks 10 meters from the flagpole, lies on the ground, and measures an angle of 70∘ from the ground to the base of the ball at the top of the flagpole. Approximately how tall is the flagpole from the ground to the base of the ball at the top of the flagpole?A3mB9mC27mD29m
A ladder 25 m long reaches a window of a building 20 m above the ground. Determine the distance of the foot of the ladder from the building.
View SolutionA ladder 17m long reaches a window of a building 15m above the ground. Find the distance of the foot of the ladder from the building.
View SolutionA ladder is placed against a vertical tower. If the ladder makes an angle of 30∘ with the ground and reached upto a height of 15 m of the tower, find the length of the ladder.
View SolutionA ladder is placed along a wall such that its upper end is resting against a vertical wall. The foot of the ladder is 2.4 m from the wall and the ladder is making an angle of 68∘ with the ground . Find the height, upto which the ladder reaches.
View SolutionA 6.5 m long ladder is placed against a wall such that its foot is at a distance of 2.5 m from the wall. Find the height of the wall where the top of the ladder touches it
View SolutionA ladder 50 dm long when set against the wall of a house just reaches a window at a height of 48 dm. How far is the lower end of the ladder from the base of the wall?
View SolutionA ladder 65 feet long is placed against a building and reaches a point that is 56 feet above the ground on one side of a street. Keeping the foot of the ladder at the same point, the ladder is turned to a building on the other side of the street. It now touches this building at a point 63 feet above the ground. find the width of the street.
View Solution
