A ladder, 25 m long reaches a window of building 20 m, above the ground. The distance of the foot of the ladder from the building.
45 m
5 m
10 m
15 m
The correct Answer is:D
To solve the problem of finding the distance of the foot of the ladder from the building, we can use the Pythagorean theorem. Here’s the step-by-step solution:
Step 1: Understand the problem
We have a right triangle formed by the ladder, the wall of the building, and the ground. The ladder acts as the hypotenuse, the height of the window is one leg, and the distance from the foot of the ladder to the building is the other leg.
Step 2: Identify the lengths
- Length of the ladder (hypotenuse, AB) = 25 m
- Height of the window (one leg, AC) = 20 m
- Distance from the foot of the ladder to the building (the other leg, BC) = ?
Step 3: Apply the Pythagorean theorem
According to the Pythagorean theorem:
AB2=AC2+BC2
Where:
- AB is the length of the ladder (25 m)
- AC is the height of the window (20 m)
- BC is the distance we need to find
Step 4: Substitute the known values
Substituting the known values into the equation:
252=202+BC2
Step 5: Calculate the squares
Calculating the squares:
625=400+BC2
Step 6: Rearrange the equation to solve for BC
Now, rearranging the equation to isolate BC2:
BC2=625−400
BC2=225
Step 7: Take the square root
Now, take the square root of both sides to find BC:
BC=√225
BC=15
Step 8: Conclusion
The distance of the foot of the ladder from the building is:
Distance=15 m
Final Answer
The distance of the foot of the ladder from the building is 15 meters.
---
We have a right triangle formed by the ladder, the wall of the building, and the ground. The ladder acts as the hypotenuse, the height of the window is one leg, and the distance from the foot of the ladder to the building is the other leg.
- Length of the ladder (hypotenuse, AB) = 25 m
- Height of the window (one leg, AC) = 20 m
- Distance from the foot of the ladder to the building (the other leg, BC) = ?
According to the Pythagorean theorem:
AB2=AC2+BC2
Where:
- AB is the length of the ladder (25 m)
- AC is the height of the window (20 m)
- BC is the distance we need to find
Substituting the known values into the equation:
252=202+BC2
Calculating the squares:
625=400+BC2
Now, rearranging the equation to isolate BC2:
BC2=625−400
BC2=225
Now, take the square root of both sides to find BC:
BC=√225
BC=15
The distance of the foot of the ladder from the building is:
Distance=15 m
The distance of the foot of the ladder from the building is 15 meters.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar14videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Exercise 6a24videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
A ladder 25 m long reaches a window of a building 20 m above the ground. Determine the distance of the foot of the ladder from the building.
View SolutionA ladder 25m long reaches a window of a building 20m above the ground. Determine the distance of the foot of the ladder from the building.
View Solution
Knowledge Check
- Question 1 - Select One
A painter needs to reach the top of a tail sign in the middle of a flat and level field .He uses a ladder of length x to reach a point on the sign 15 feet above the ground . The angle formed where the ladder meets the ground is noted in the figure below as θ , which of the following relation - ships must be true ?
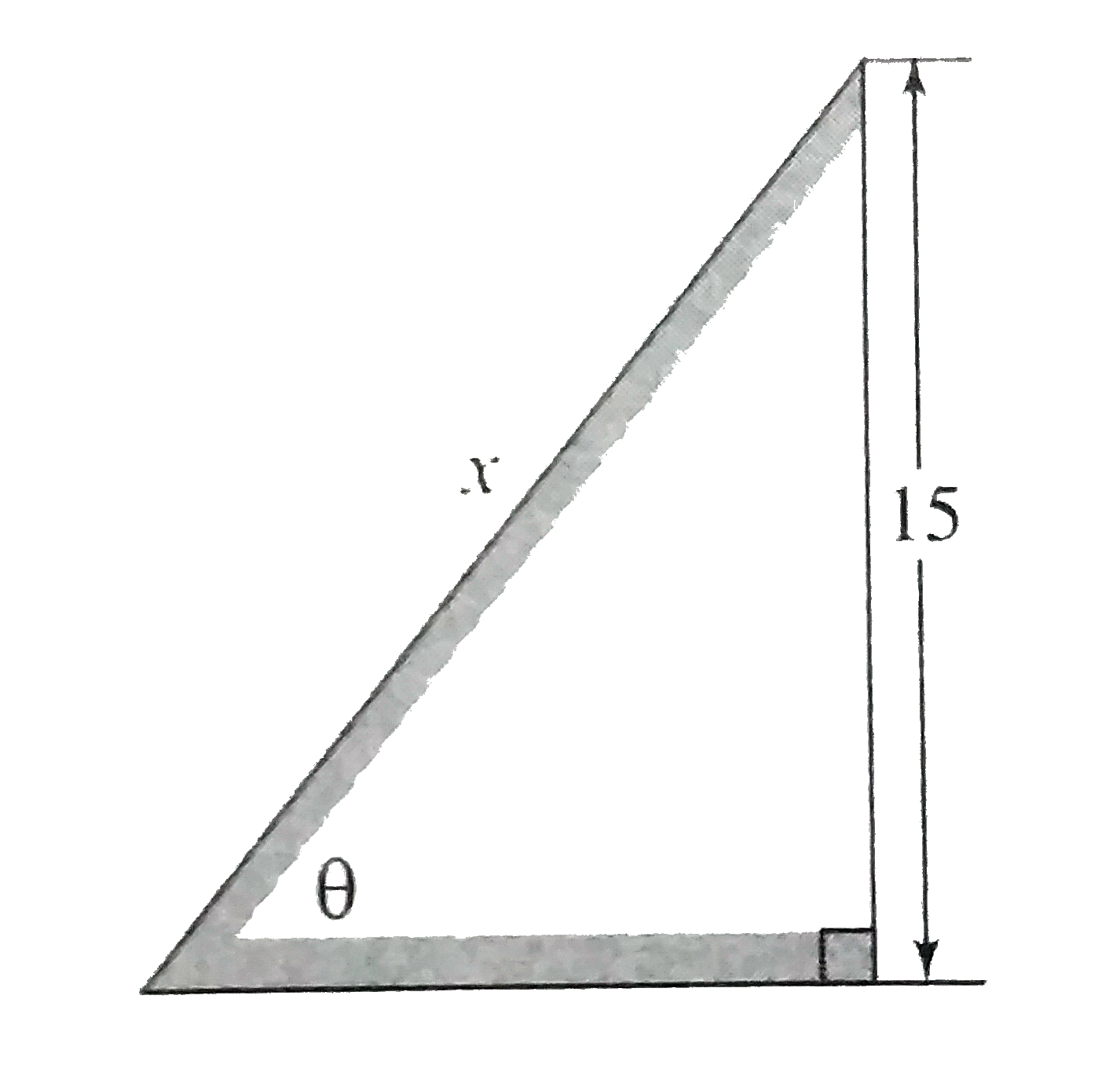 Asinθ=15xBcosθ=15xCtanθ=15xDθ=15x
Asinθ=15xBcosθ=15xCtanθ=15xDθ=15x - Question 2 - Select One
A 25 foot ladder is placed against the side of a building at an angle of 70∘ from the ground. How far away is the base of the ladder from the building?
A25cos70∘B8.5sin70∘C25tan70∘D8.5cos70∘
A ladder 17m long reaches a window of a building 15m above the ground. Find the distance of the foot of the ladder from the building.
View SolutionA ladder 10m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from base of the wall.
View SolutionA ladder 15 m long reaches a window which is 9 m above the ground on one side of a street. Keeping its foot at the some point, the ladder is turned to other side of the street to reach a window 12 m high. Find the width of the street.
View SolutionA ladder 15 m long reaches a window which is 9 m above the ground on one side of a street. Keeping its foot at the some point, the ladder is turned to other side of the street to reach a window 12 m high. Find the width of the street.
View SolutionA ladder 17 m long reaches a window which is 8 m above the ground, on one side of the street. Keeping its foot at the same point, the ladder is turned to the other side of the street to reach a window at a height of 15 m. Find the width of the street.
View SolutionA ladder reaches a window which is 15 metres above the ground on one side of the street. Keeping its food at the same point, the ladder is turned to the other side of the street to reach a window 8 metre high. Find the width of the street, if the length of the ladder is 17 metres.
View Solution

