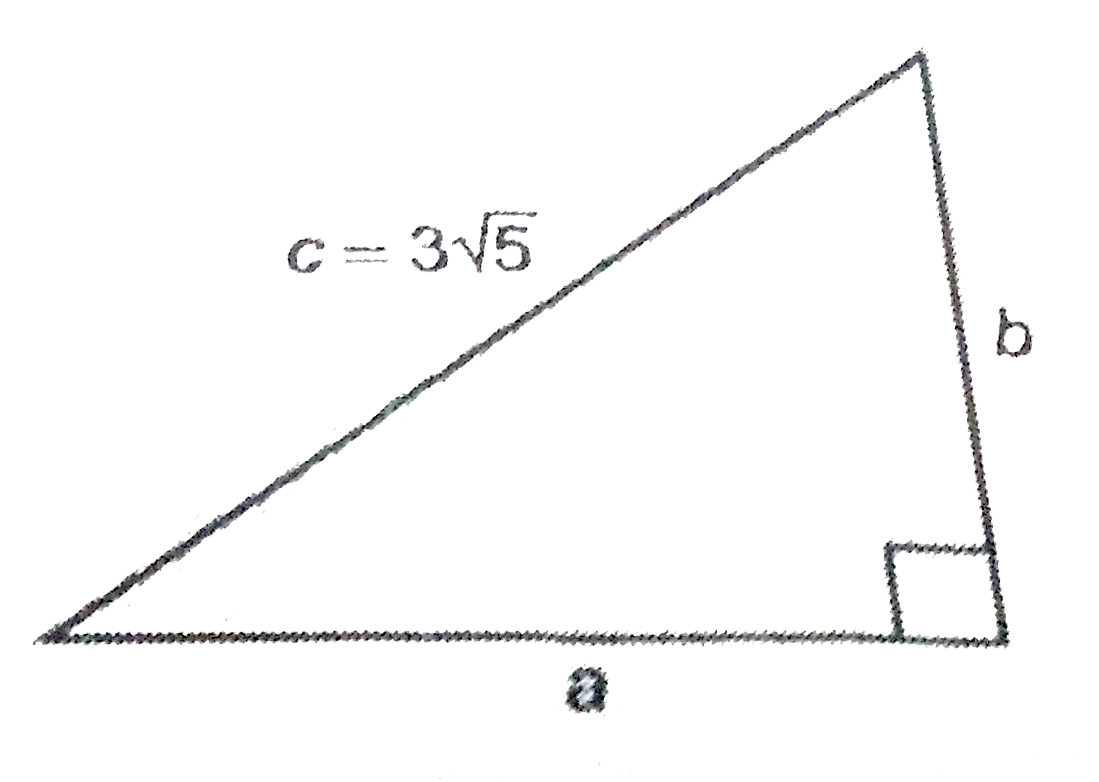
a, b and c are the integral sides of a right angled triangle in which c is the hypotenuse measuring 3√5 metres. If side 'a' is increased by 4003% and side 'b' is increased by
503%, then their total becomes 14 metres. Find the area of triangle.
Video Solution
Text Solution
By Pythagoras theorem,
a2+b2=(3√5)2
⇒a2+b2=45 ......(1)
Now length of base =a+a×4003×1100=7a3
and perpendicular =b+b×503×100=7a6
According to the problem, 7a3+7a6=14
⇒14a+7b=84⇒b=12−2a
Substituting this value in equation (1), we get
a2+(12−2a)2=45
⇒a2+144+4a2−48a=45
⇒5a2−48a+99=0
⇒5a2−15a−33a+99=0
⇒5a(a−3)−33(a−3)=0
⇒(a−3)(5a−33)=0
∵a=3ora=335
But a=335 is not an integer.
So, we reject a=335
therefore a=3
Hence, a=3m,b=12−2×3=6
∵AreaofΔ=12ab=12×3×6=9m2
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - QUADRATIC EQUATIONSEXERCISE - Problems From NCERT /exemplar17videos
QUADRATIC EQUATIONS
- BOOK - NAGEEN PRAKASHANCHAPTER - QUADRATIC EQUATIONSEXERCISE - Exercise 4a37videos
QUADRATIC EQUATIONS
- BOOK - NAGEEN PRAKASHANCHAPTER - PROBABILITYEXERCISE - Revision Exercise Very Short Answer/short Answer Questions16videos
PROBABILITY
- BOOK - NAGEEN PRAKASHANCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions5videos
REAL NUMBERS
Similar Questions
Knowledge Check
- Question 1 - Select One
The base of a right angled triangle is 5 metres and hypotenuse is 13 metres. Its area will be -----
A25m2B28m2C30m2DNone of these - Question 2 - Select One
In a right-angled triangle with sides a and b, hypotenuse c, the altitude drawn on the hypotenuse is x. Then
Aab=x2B1a+1b=1xCa2+b2=2x2D1a2+1b2=1x2 - Question 3 - Select One
The sides of a right triangle ABC are a, b and c, where c is the hypotenuse. What will be the radius of the in circle of this triangle?
A(a+b+c)2B(a+b−c)2C(b+c−a)2D(a+c−b)2
The base of a right-angled triangle measures 48 cm and its hypotenuse measures 50 cm. Find the area of the triangle.
View SolutionThe base of a right - angled triangle measures 48 cm and its hypotenuse measures 50 cm . Find the area of the triangle .
View SolutionA field is in the form of a right triangle with hypotenuse 50 m and one side 30 m. Find the area of the field.
View SolutionIf each side of an equilateral triangle is tripled then what is the percentage increase in the area of the triangle?
View SolutionIf a,b and c are the lengths of the sides of a right triangle ABC with c=2a and b2−3a2=0, then ∠ABC=……………………
View Solution

