AB is a diameter of a circle. CD is a.chord parallel to AB and 2CD=AB. The tangent at B meets the line AC produced at E and AE=K.AB, then K is equal to
The correct Answer is:2
Topper's Solved these Questions
Similar Questions
AB is a diameter of a circle. CD is a chord parallel to AB and 2CD=AB. The tangent at B meets the line AC produced at E then AE is equal to -
View SolutionAB is a diameter of a circle. CD is a chord parallel to AB and 2CD = AB. The tangent at B meets the line AC produced at E then AE is equal to
View Solution
Knowledge Check
If A={a,b,c,d}andB={b,c,e}, then A∪B is equal to
A{a,b,c,d,e}BϕC{b,c,d}DNone of theseIf A={a,b,c,d}andB={b,c,e}, then A∪B is equal to
A{a,b,c,d,e}BϕC{b,c,d}DNone of these
AB is a diameter of a circle. CD is a chord parallel to AB and 2CD=AB. The tangent at B meets the line AC produced at E then AE is equal to -
View SolutionAB is a diameter of a circle C(O, r). Chord CD is equal to radius OC. If AC and BD when produced intersect at P, prove that ∠APB is constant.
View SolutionABCD is a parallelogram. AB is produced to E so that BE=AB . Prove that ED bisects BC.
View SolutionIn Figure, A is the centre of the circle. ABCD is a parallelogram and CDE is a straight line. Find ∠BCD : ∠ABE.
View SolutionIf the diagram, DC is a diameter of the large circle centered at A, and AC is a diameter of the smaller circle centered at B. If DE is tangent to the smaller circle at F and DC=12 then the length of DE is
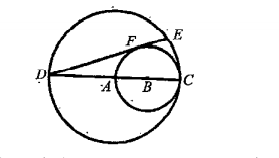 .
.
View SolutionABCD is a parallelogram and E is the mid-point of BC, DE and AB when produced meet at F. Then AF=
View Solution
