A boy goes to a school from his home at a speed of x km/hr and comes back at a speed of y km/hr, then the average speed is given by
AM
GM
HM
none of these
The correct Answer is:C
To find the average speed of a boy who travels to school at a speed of x km/hr and returns at a speed of y km/hr, we can follow these steps:
1. Define the Distance:
Let the distance between the boy's home and the school be s kilometers.
2. Calculate Time Taken to Reach School:
The time taken to go from home to school at a speed of x km/hr is given by the formula:
Time=DistanceSpeed=sx hours
3. Calculate Time Taken to Return Home:
The time taken to return from school to home at a speed of y km/hr is:
Time=sy hours
4. Total Distance:
The total distance traveled (to school and back) is:
Total Distance=s+s=2s kilometers
5. Total Time:
The total time taken for the round trip is:
Total Time=sx+sy
6. Finding the Average Speed:
The average speed is calculated using the formula:
Average Speed=Total DistanceTotal Time
Substituting the values we have:
Average Speed=2ssx+sy
7. Simplifying the Expression:
We can factor out s from the denominator:
Average Speed=2ss(1x+1y)=21x+1y
This simplifies to:
Average Speed=2xyx+y km/hr
8. Identifying the Type of Mean:
The expression 2xyx+y is recognized as the harmonic mean of x and y.
Final Answer:
The average speed of the boy is given by:
Average Speed=2xyx+y km/hr
This corresponds to the harmonic mean of the speeds x and y.
---
Let the distance between the boy's home and the school be s kilometers.
The time taken to go from home to school at a speed of x km/hr is given by the formula:
Time=DistanceSpeed=sx hours
The time taken to return from school to home at a speed of y km/hr is:
Time=sy hours
The total distance traveled (to school and back) is:
Total Distance=s+s=2s kilometers
The total time taken for the round trip is:
Total Time=sx+sy
The average speed is calculated using the formula:
Average Speed=Total DistanceTotal Time
Substituting the values we have:
Average Speed=2ssx+sy
We can factor out s from the denominator:
Average Speed=2ss(1x+1y)=21x+1y
This simplifies to:
Average Speed=2xyx+y km/hr
The expression 2xyx+y is recognized as the harmonic mean of x and y.
The average speed of the boy is given by:
Average Speed=2xyx+y km/hr
This corresponds to the harmonic mean of the speeds x and y.
Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Exercise
40 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test
29 Videos
- MISCELLANEOUS EQUATIONS AND INEQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test
29 Videos
Similar Questions
Knowledge Check
A boy goes from his house to school by bus at a speed of 20kmh−1 and returns back through the same route at a speed of 30kmh−1 . The average speed of his journey is :
A24kmh−1B25kmh−1C30kmh−1D20kmh−1
A particle goes from A to B with a speed of 40km/h and B to C with a speed of 60Km/h. If AB=6BC, the average speed in km/h between A and C is-
[Hint : Average speed =total distance travelledtime taken]
View SolutionA motorcyclist drives from A to B with a uniform speed of 30kmh−1 and returns back with a speed of 20kmh−1. Find its average speed.
View SolutionA man walks on a straight road from his home to a market 2.5 km away with a speed of 5 km/h. Finding the market closed, he instantly turns and walks back home with a speed of 7.5 km/h. The average speed of the man over the interval of time 0 to 40 min, is equal to
View SolutionA bo walks to his school at a distance of 6 km with constant speed of 2.5kmh−1 and walks back with a constant speed of 4kmh−1. His average speed for round trip expressed in kmh−1, is
View SolutionA man starting from point P cross a 4km wide lagoon and reaches point Q in the shortest possible time by the path shown in the figure. If the person swims at a speed of 3km//hr and walks at a speed of 4km//hr, then find his time fo journey (in minutes).
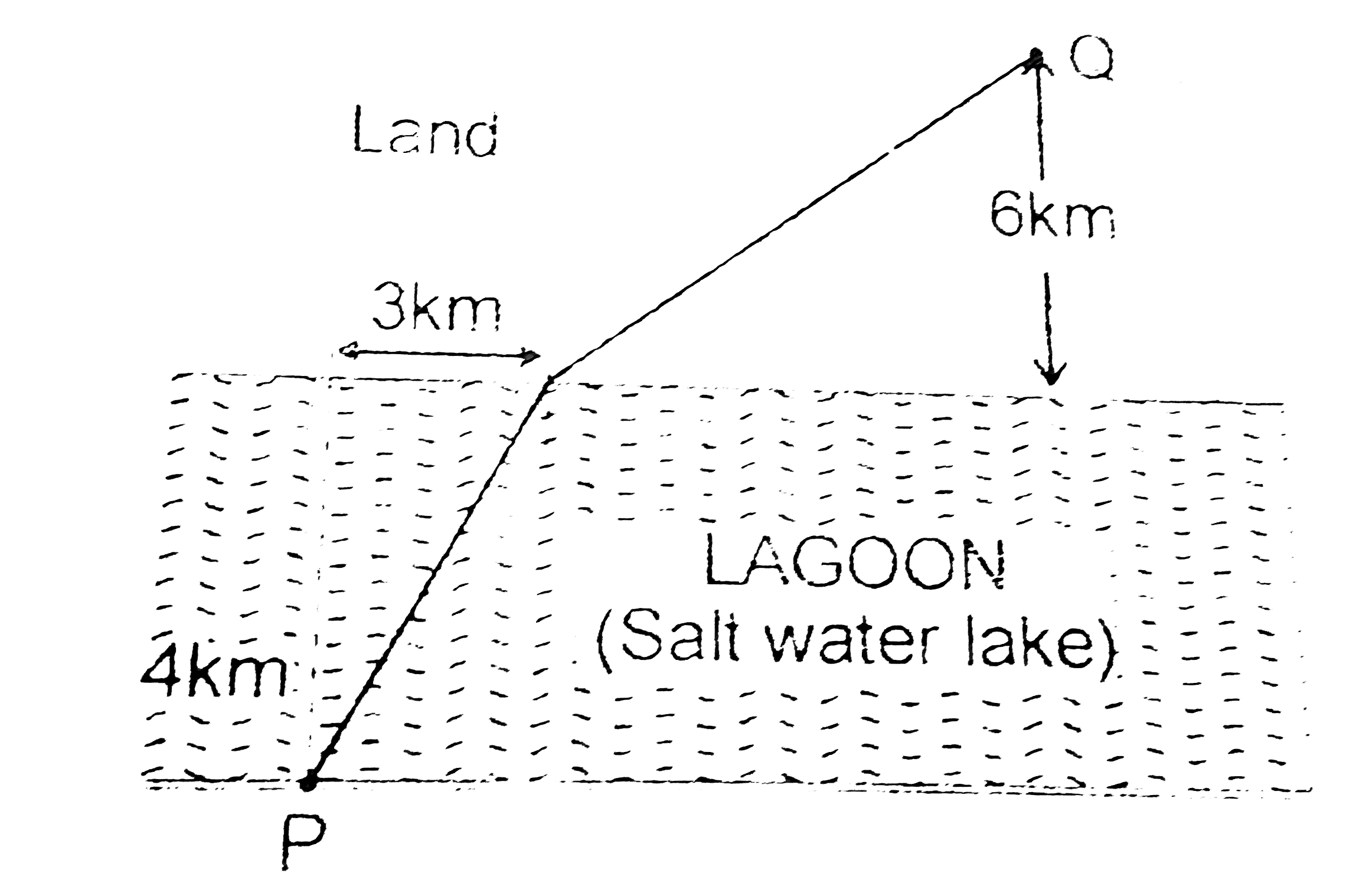 .
.
View SolutionA man at a speed of 6 km/hr for 1 km and 8 km/hr for the next 1 km. What is t his average speed for the walk of 2 km?
View SolutionA person walks along a straight road from his house to a market 2.5kms away with a speed of 5 km/hr and instantly turns back and reaches his house with a speed of 7.5 kms/hr. The average speed of the person during the time interval 0 to 50 minutes is (in m/sec)
View Solution