A circle can have .............parallel tangents at the most.
The correct Answer is:Two
To solve the question "A circle can have .............parallel tangents at the most," we need to analyze the properties of tangents to a circle.
1. Understanding Tangents: A tangent to a circle is a line that touches the circle at exactly one point. For a line to be a tangent, it must not intersect the circle at any other point.
2. Parallel Lines: When we talk about parallel tangents, we are referring to lines that do not intersect each other and maintain a constant distance apart.
3. Drawing the Circle: Imagine a circle drawn on a plane.
4. Identifying Tangents: From any point outside the circle, you can draw two tangents to the circle. These tangents will touch the circle at two distinct points.
5. Parallel Tangents: If we want to find parallel tangents, we can draw two lines that are parallel to each other and tangent to the circle.
6. Maximum Number of Parallel Tangents: A circle can have at most two parallel tangents. This is because if you try to draw a third tangent that is parallel to the first two, it will intersect the circle at two points, which disqualifies it from being a tangent.
7. Conclusion: Therefore, the maximum number of parallel tangents that can be drawn to a circle is two.
Final Answer:
A circle can have two parallel tangents at the most.
---
A circle can have two parallel tangents at the most.
Topper's Solved these Questions
- BOOK - MBD -HARYANA BOARDCHAPTER - MBD NEW STYLE MODEL TEST PAPER -1 EXERCISE - SET - C (SECTION - B)2videos
MBD NEW STYLE MODEL TEST PAPER -1
- BOOK - MBD -HARYANA BOARDCHAPTER - MBD NEW STYLE MODEL TEST PAPER -1 EXERCISE - SET - C (SECTION - C)5videos
MBD NEW STYLE MODEL TEST PAPER -1
- BOOK - MBD -HARYANA BOARDCHAPTER - MBD NEW STYLE MODEL TEST PAPER -1 EXERCISE - SET - B (SECTION - D)4videos
MBD NEW STYLE MODEL TEST PAPER -1
- BOOK - MBD -HARYANA BOARDCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - LONG SHORT ANSWER TYPE QUESTIONS8videos
INTRODUCTION TO TRIGONOMETRY
- BOOK - MBD -HARYANA BOARDCHAPTER - MBD NEW STYPE MODEL TEST PAPER - 2EXERCISE - SET - D (SECTION - D)6videos
MBD NEW STYPE MODEL TEST PAPER - 2
Similar Questions
Fill in the blanks: The common point of a tangent and the circle is called...... A circle may have ..... parallel tangents. A tangent to a circle intersects it in ..... point(s). A line intersecting a circle in two points is called a ........ (v) The angle between tangent at a point on a circle and the radius through the point is .........
View SolutionPoint O is the centre of a circle . Line a and line b are parallel tangents to the circle at P and Q. Prove that segment PQ is a diameter of the circle.
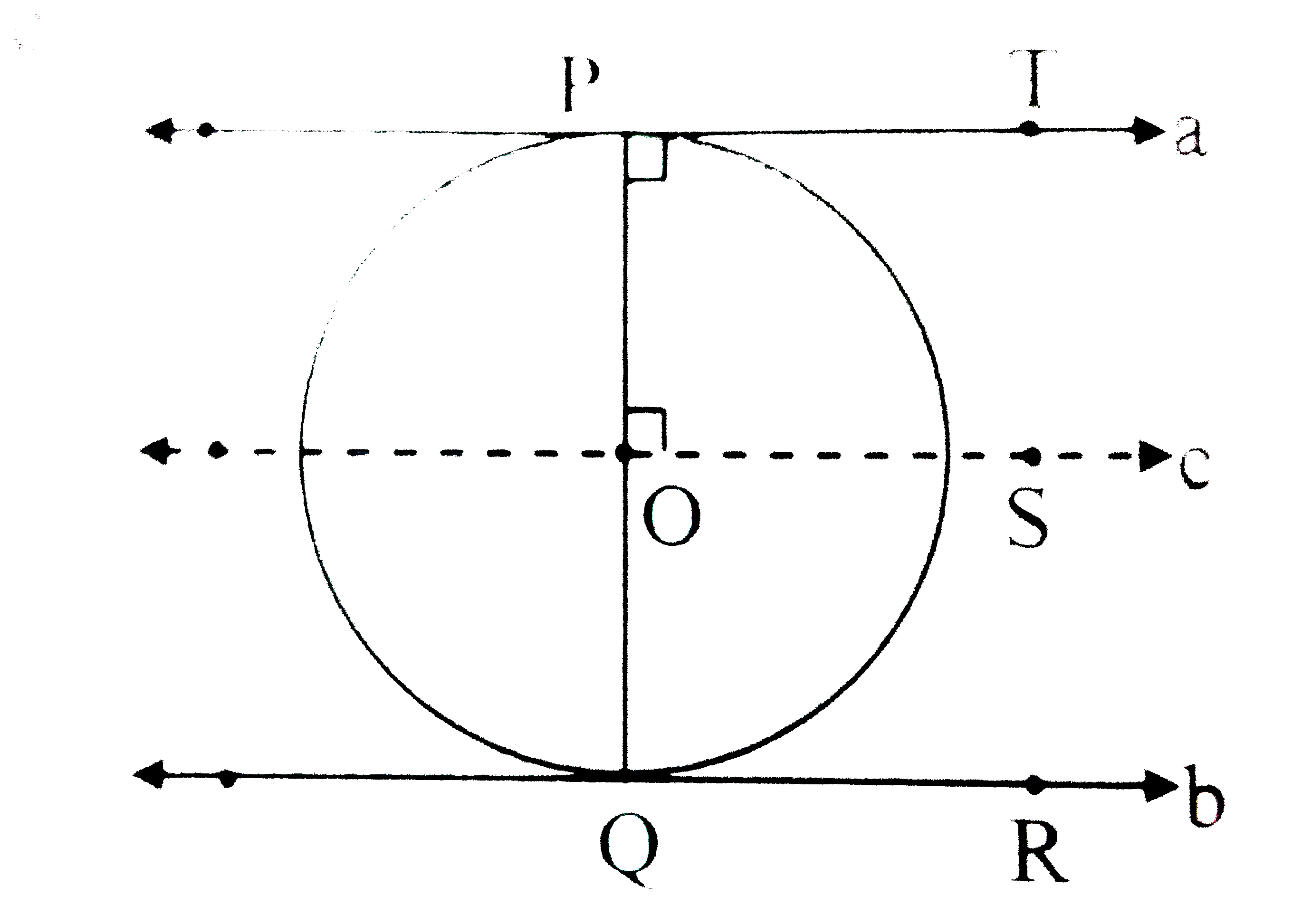 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In a circle, PQ and RS are two parallel tangents at A and B. The tangent at makes an intercept DE between PQ and RS. Then ∠DOE is equal to : (where O is centre).
A90∘B120∘C60∘D45∘ - Question 2 - Select One
How many parallel tangents can a circle have ?
A1B2CinfiniteD0 - Question 3 - Select One
Which of the following pairs of lines can be parallel ?
1. Two tangents to a circle.
2. Two diameters of a circle.
3. A chord of circle and a tangent to a tangent to a circle.
4. Two chords of a circle.
Select the correct answer using the codes given below :A1, 2 and 3B2, 3 and 4C1, 3 and 4D1, 2 and 4
Fill in the blank :
From a point on a circle ............. Tangent (s) can be drawn.View SolutionProve that a diameter AB of a circle bisects all those chords which are parallel to the tangent at the point A.
View SolutionGiven two concentric circles of radii r and 2r, two parallel tangents to the inner circle cut off an arc from the outer circle, find its length.
View SolutionThe extremities of the diameter of a circle are (1, 2) and (3, 4). Then its centre is .........., radius .......... and equation is .......... .
View SolutionTwo circles have an external tangent with length 36 cm. The shortest distance between these circles is 14 cm. If the radius of the longer circle is 4 times the radius of the smaller circle then the radius of the larger circle in cms is
View Solution

