A circle has radius $\sqrt{2}$ cm it is divided into 2 segments by a chord of length 2cm prove that angle subtended by the chord at a point in major segment is $45^{\circ}$

Video Solution

Text Solution

Verified by Experts

Let O be the centre of the given circle and let AB be a chord of length 2 cm.
$∴ O A = O B = \sqrt{2} c m$ .
Clearly, $O A^{2} = + O B^{2} = {{(\sqrt{2})}^{2} + {(\sqrt{2})}^{2}} c m^{2}$
$= 4 c m^{2} = {(2 c m)}^{2} = A B^{2}$ .

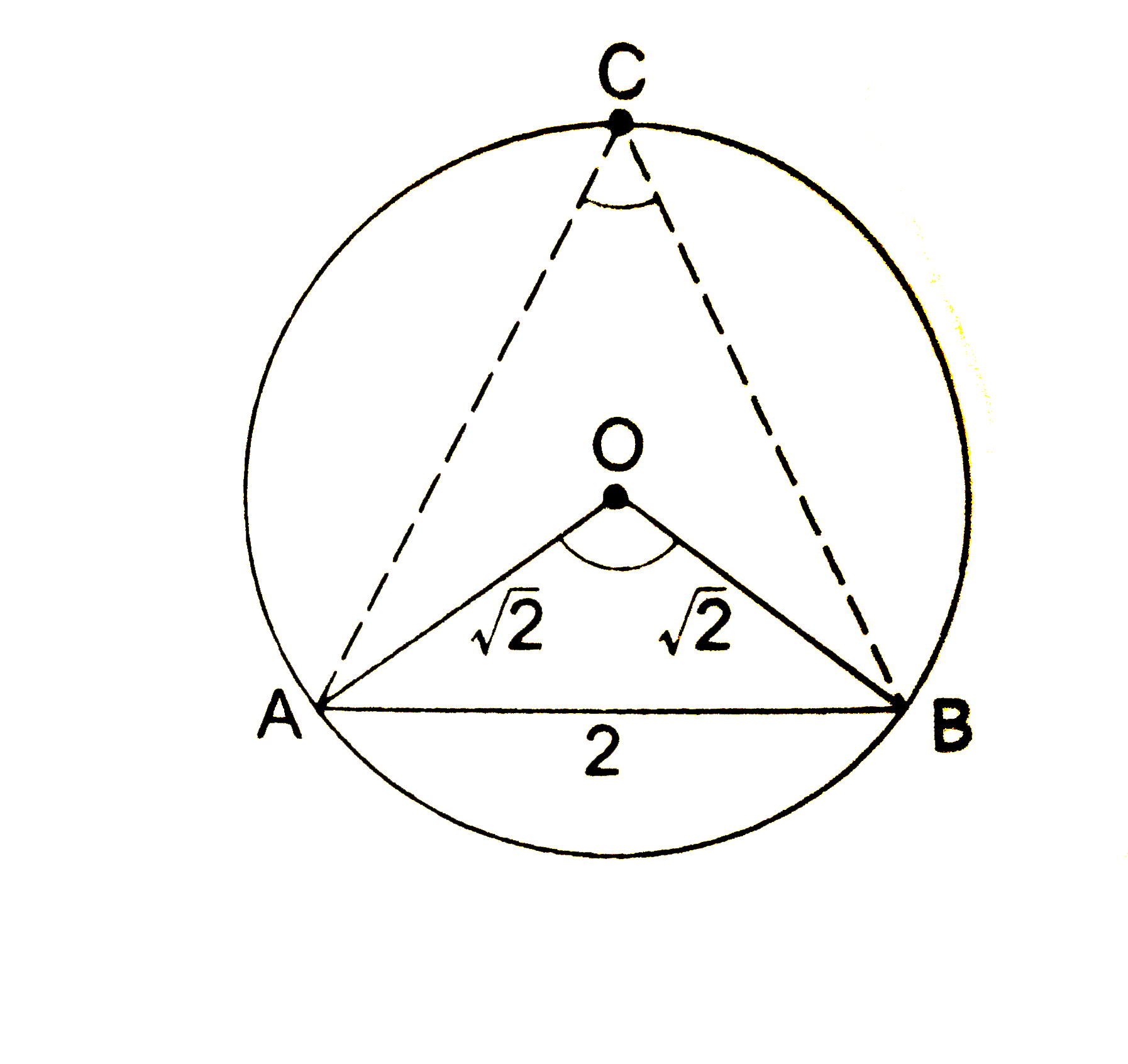
$∴ O A^{2} + O B^{2} = A B^{2}$
Consequently , $∠ A O B = 90^{\circ}$
Let C be a point in the major segment of the circle . Join CA and CB
Since the angle sbtended by an arc of a circle at its centre is twice the angle subtended by it at any point on the remaining part of the circle , we have
$∠ A C B = \frac{1}{2} ∠ A O B = (\frac{1}{2} \times 90^{\circ}) = 45^{\circ}$

Updated on:21/07/2023