A circle of radius 5 is tangent to the line at M(3, -2) and lies above the line. The equation of the circle is
The correct Answer is:C
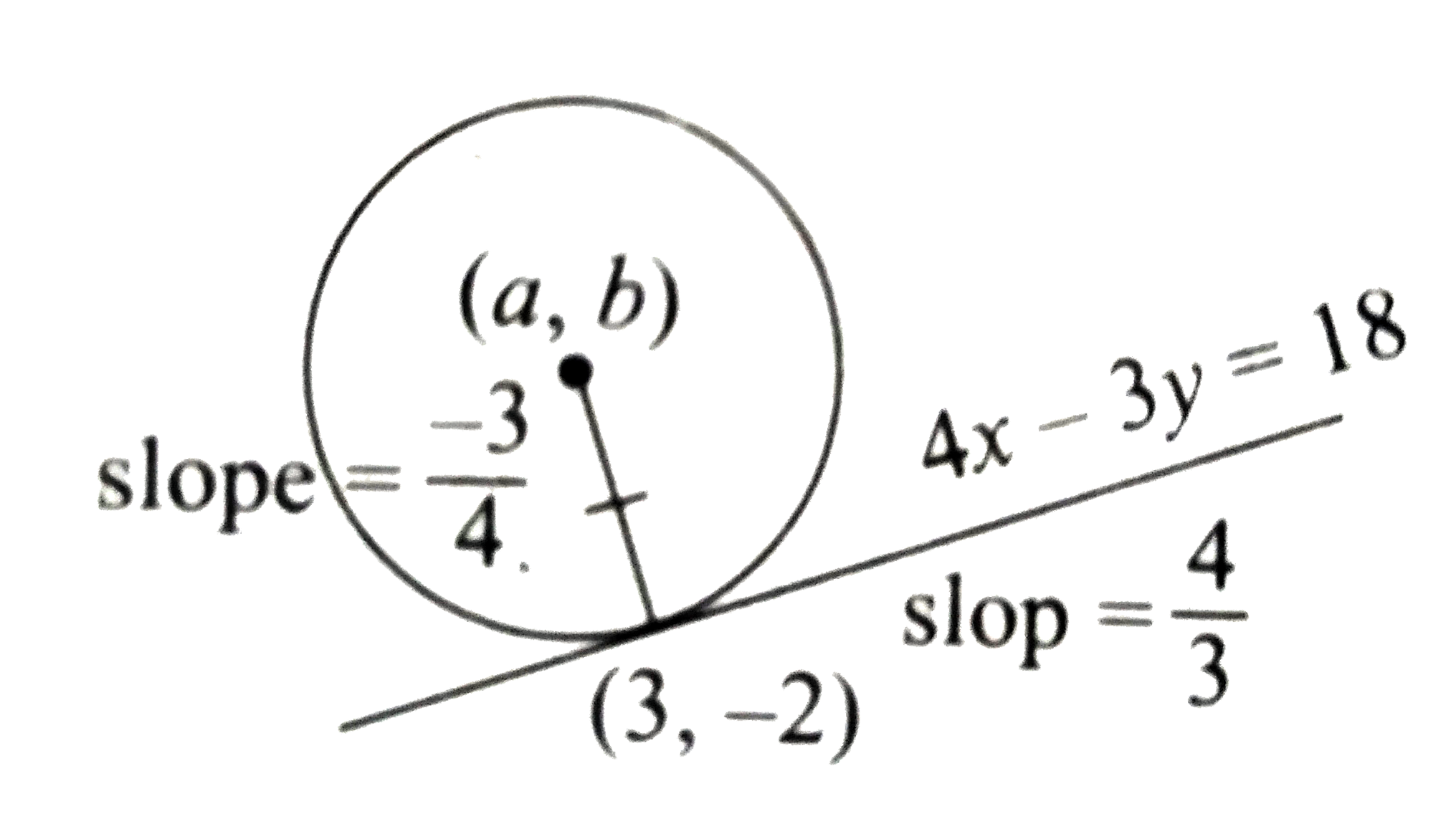
Let , where
and
we have, and
and
centre
Hence, required equation of circle is
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Advanced (Single Correct Answer Type)
14 Videos
- COMPLEX NUMBERSBOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Question Bank
30 Videos
Similar Questions
Knowledge Check
A circle has two of its diameters along the lines 2x+3y−18=0and3x−y−5=0 and touches the line x+2y+4=0. Equation of the circle is
Ax2+y2+6x−8y+16=0Bx2+y2−6x+8y+16=0Cx2+y2−6x−8y+9=0Dx2+y2−6x−8y+16=0The equation of the circle of radius 2√2 whose centre lies on the line x−y=0 and which touches the line x+y=4, and whose centre is coordinate satisfy x+y>4, is
Ax2+y2−8x−8y+24=0Bx2+y2=8Cx2+y2−8x+8y−24=0Dnone of theseA circle of radius √8 is passing through origin the point (4,0) . If the centre lies on the line y = x , then the equation of the circle is
A(x−2)2+(y−2)2=8B(x+2)2+(y+2)2=8C(x−3)2+(y−3)2=8D(x+3)2+(y+3)2=8
A circle has radius 3 units and its centre lies on the line y=x-1. Find the equation of the circle if it passes through (7,3).
View SolutionThe line 5x−y=3 is a tangent to a circle at the point (2,7) and its centre is on theline x+2y=19. Find the equation of the circle.
View SolutionCentre of a circle of radius 4√5 lies on the line y=x and satisfies the inequality 3x+6y>10. If the line x+2y=3 is a tangent to the circle, then the equation of the circle is
View SolutionThe line 2x - y + 1 = 0 is tangent to the circle at the point (2,5) and the centre of the circles lies on x-2y = 4. The radius of the circle is :
View SolutionThe line 2x−y+1=0 is tangent to the circle at the point (2,5) and the centre of the circles lies on x−2y=4. The radius of the circle is
View Solution
