A cyclist moving on a level road at 4 m/s stops pedalling and lets the wheels come to rest. The retardation of the cycle has two components: a constant 0.08 m/s2 due to friction in the working parts and a resistance of 0.02v2/m , where v is speed in meters per second. What distance is traversed by the cycle before it comes to rest? (consider 1n 5=1.61).
Let the cyclist starting to move from the point O and moving along OX, attains a velocity v at point P in time t such that OP=x. Let the acceleration of the moving cycle at P be a. Then we know that
v=dxdt and a=dvdt=d2xdt2=vdvdt ............(1)
By hypothesis, retardation =0.08+0.02v2=0.02(4+v2)
or vdvdx=−0.02(4+v2)
or dx=−10.02vdv4+v2 .............(2)
Integrating equation (2) between the limits x=0, v=4 m/x, and x=x′ meters, v=0, we get
∫x′0dx=−10.04∫042vdv4+v2
or x′=−10.04[ln4−ln20]
=ln50.04=1.610.04=u1614m
Topper's Solved these Questions
- DIFFERENTIAL EQUATIONSBOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Exercise 10.1
6 Videos
- DIFFERENTIAL EQUATIONSBOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Exercise 10.2
6 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGECHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise
337 Videos
- DIFFERENTIATIONBOOK - CENGAGECHAPTER - DIFFERENTIATIONEXERCISE - Numerical Value Type
3 Videos
Similar Questions
A bullet going with speed 350 m/s enters concrete wall and penetrates a distance of 5.0 cm before coming to rest. Find the deceleration.
View SolutionA body moving on the ground with a velocity of 15m/s comes to rest after covering a distance of 25m If acceleration due to gravity is 10m/s2 Calculate the coefficient of friction between the ground and the body .
View Solution
Knowledge Check
A car acceleration from rest at a constant rate 2m/s2 for some time. Then, it retards at a constant rate of 4m/s2 and comes to rest. If it remains motion for 3 second, then the maximum speed attained by the car is:-
A2m/sB3m/sC4m/sD6m/sA scooter moving at a speed of 10 m/s is stopped by applying brakes which produce a uniform acceleration of, -0.5 m/s2. How much distance will be covered by the scooter before it stops ?
A50 mB75 mC100 mDNone of theseA 0.5kg block slides from the point A on a horizontal track with an initial speed 3m/s towards a weightless horizontal spring of length 1m and force constant 2N/m. The part AB of the track is frictionless and the part BC has the coefficient of static and kinetic friction as '0.22' and 0.20 respectively. If the distances AB and BD are 2m and 2.14m respectively, find total distance through which the block moves before it comes to rest completely. (g=10 m//s^(2) ).
A3.23 mB4.14 mC5.24 mD4.67 m
A particle is moving along a straight line.The initial velocity is 10ms and it is , moving with constant retardation of 2ms2 .. The distance travelled by the particle until it comes to rest is
View SolutionTwo blocks m1andm2 are connected by a spring of force constant K and are placed on a frictionless horizontal surface. Initially the spring is given extension x0 when the sysetem is released from rest, find the distance moved by two blocks before they again comes to rest.
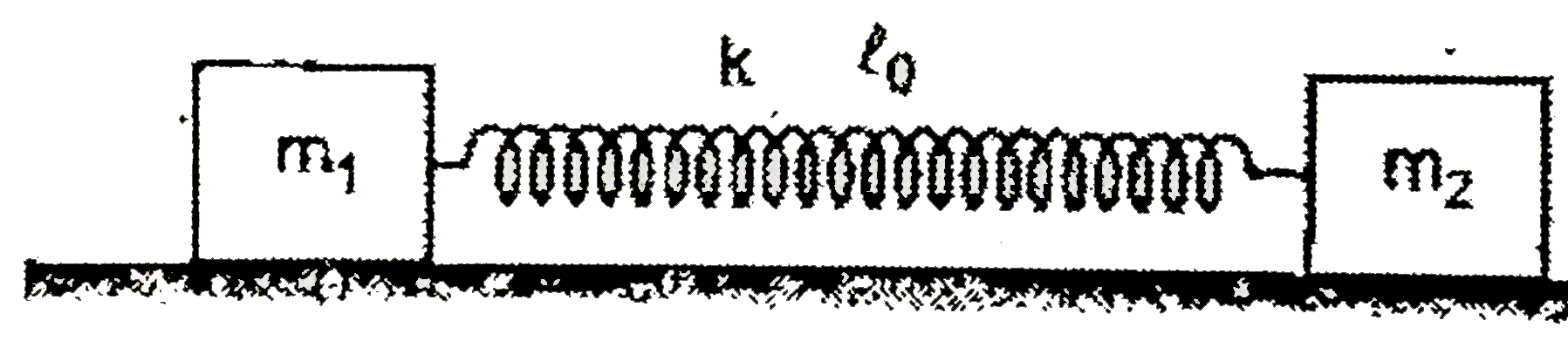
View SolutionThe speed(v) of a particle moving along a straight line is given by v=(t2+3t−4 where v is in m/s and t in seconds. Find time t at which the particle will momentarily come to rest.
View SolutionAn object moving with a speed of 6.25m/s , is decreased at a rate given by (dv/dt)=-2.5sqrt(v) where v is instantaneous speed, The time taken by the object to come to rest, would be
View SolutionA body of mass m is travelling with a velocity vu. When a constant retarding force F is applied, it comes to rest after travelling a distance s1. If the initial velocity is 2u with the same force F, the distance travelled before it comes to rest is s2. then,
View Solution
