A Δ ABC is right angled at A. L is a point on BC such that AL⊥ BC. Prove that ∠BAL=∠ACB.
Video Solution
Text Solution
Sicne ∠A=90∘ (given)
∴∠1+∠3=90∘....(1)
Also, since AL⊥BC (given)
∴∠4=90∘ ...(2)
(by angle sum property, ∠2+∠3+∠4=180∘

From (1) and (2), we get
∠1+∠3=∠2+∠3
⇒∠1=∠2
|
Topper's Solved these Questions
- LINES AND ANGLESBOOK - NAGEEN PRAKASHANCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6 A
19 Videos
- LINES AND ANGLESBOOK - NAGEEN PRAKASHANCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6 B
16 Videos
- LINES AND ANGLESBOOK - NAGEEN PRAKASHANCHAPTER - LINES AND ANGLESEXERCISE - Revision Exercise (long Answer Questions )
6 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Revision Exercise
12 Videos
- NUMBER SYSTEMBOOK - NAGEEN PRAKASHANCHAPTER - NUMBER SYSTEMEXERCISE - Revision Exercise (short Answer Questions)
10 Videos
Similar Questions
Knowledge Check
If ABC is an equilateral triangle and D is a point on BC such that AD⊥BC, then
AAB:BD=1:1BAB:BD=1:2CAB:BD=2:1DAB:BD=3:2ΔABC is right angled at A and AD⊥BC. Then BDDC=
A(ABAC)2BABACC(ABAD)2DABADABC is a triangle right- angled at B and D is a point on BC produced (BD>BC), such that BD=2DC. Which one of the following is correct?
AAC2=AD2−3CD2BAC2=AD2−2CD2CAC2=AD2−4CD2DAC2=AD2−5CD2
A triangle ABC is right angles at A.AL is drawn perpendicular to BC . Prove that ∠BAL= ∠ACB
View SolutionABC is a triangle in which AB=ACandD is a point on AC such that BC2=AC×CD.Prove that BD=BC.
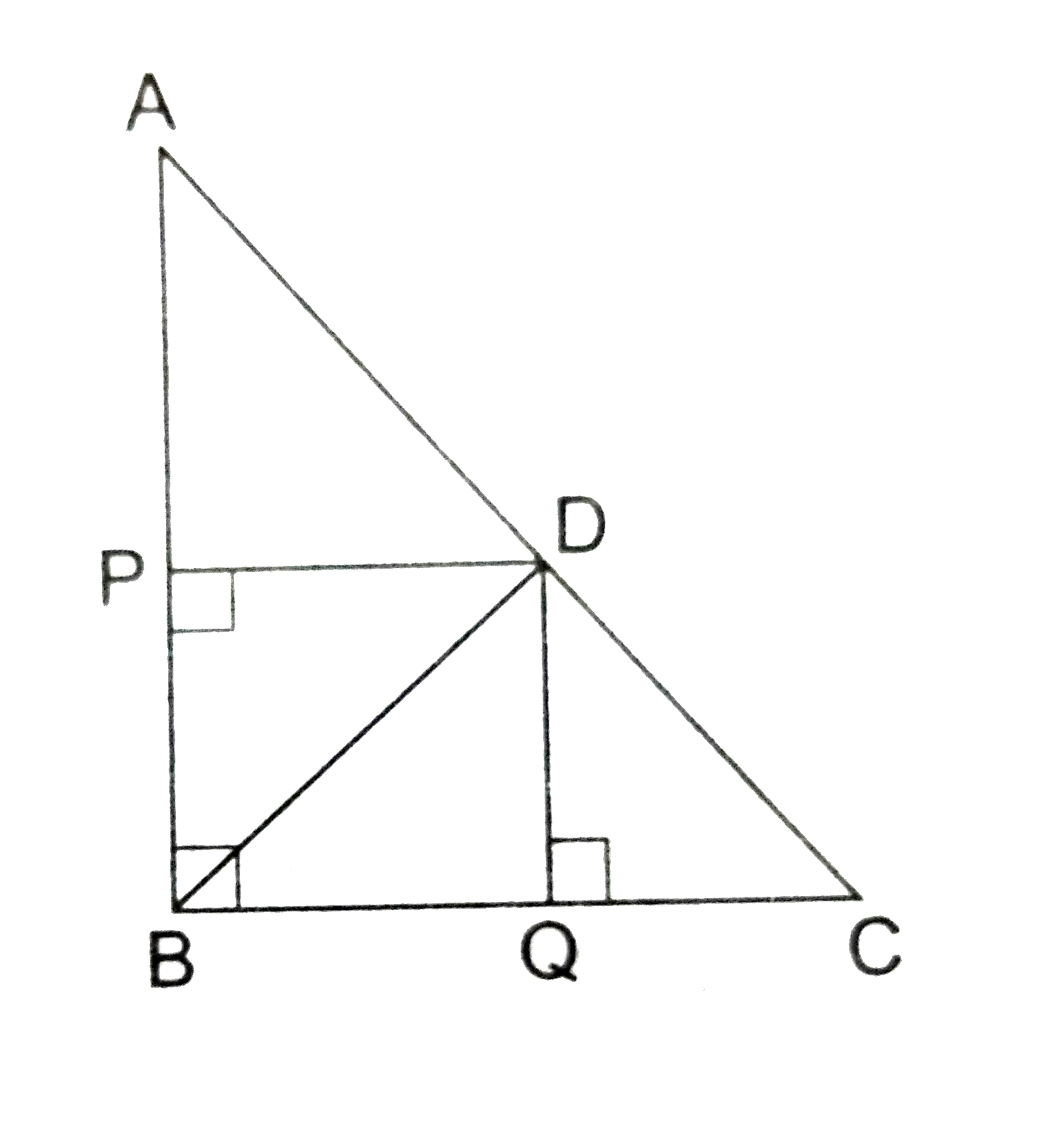
View SolutionIn a right tringle ABC, right-angled at B, D is a point on hypotenuse such that BD⊥AC. If DP⊥ABandDQ⊥BC then prove that
(a)DQ2=DP⋅QC DP2=DQ⋅AP.
View SolutionABC is a triangle right-angled at B and D is a point on BC produced (BD > BC), such that BD = 2 DC. Which one of the following is correct?
View SolutionTriangle ABC is right angle at A. The points P and Q are on hypotenuse BC such that BP=PQ=QC.if AP=3andAQ=4, then length BC is equal to
View Solution
