In a right tringle ABC, right-angled at B, D is a point on hypotenuse such that BD⊥AC. If DP⊥ABandDQ⊥BC then prove that
(a)DQ2=DP⋅QC DP2=DQ⋅AP.
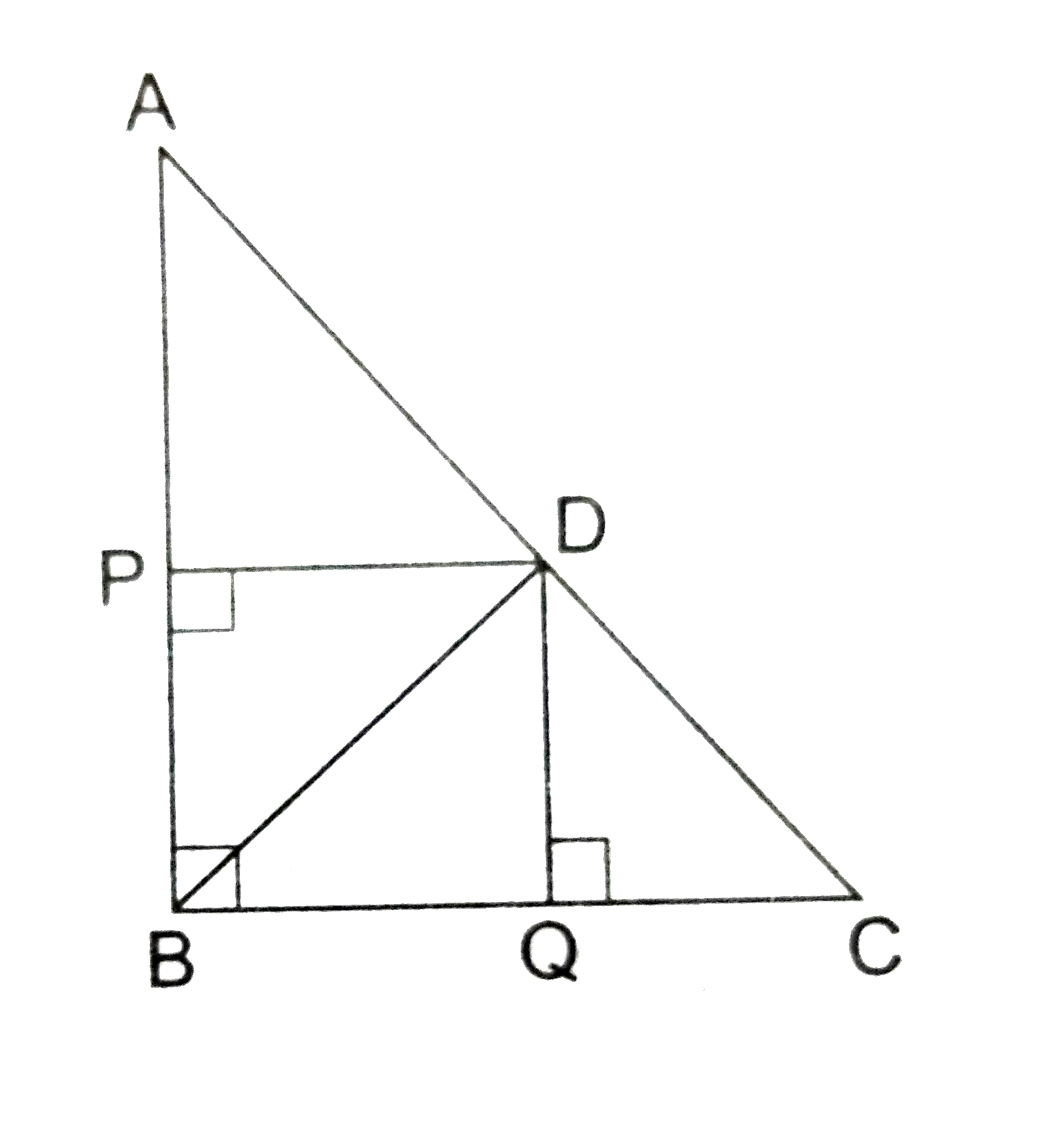
AB⊥BCandDP⊥AB⇒DP||BC⇒DP||BQ
BC⊥ABandDQ⊥BC⇒DQ||DQ||PB
∴BDQP is rectangle.
And so, BPandDPandDQ ltgbr In rt. ΔBQ:∠1+∠2=90∘ ...(i)
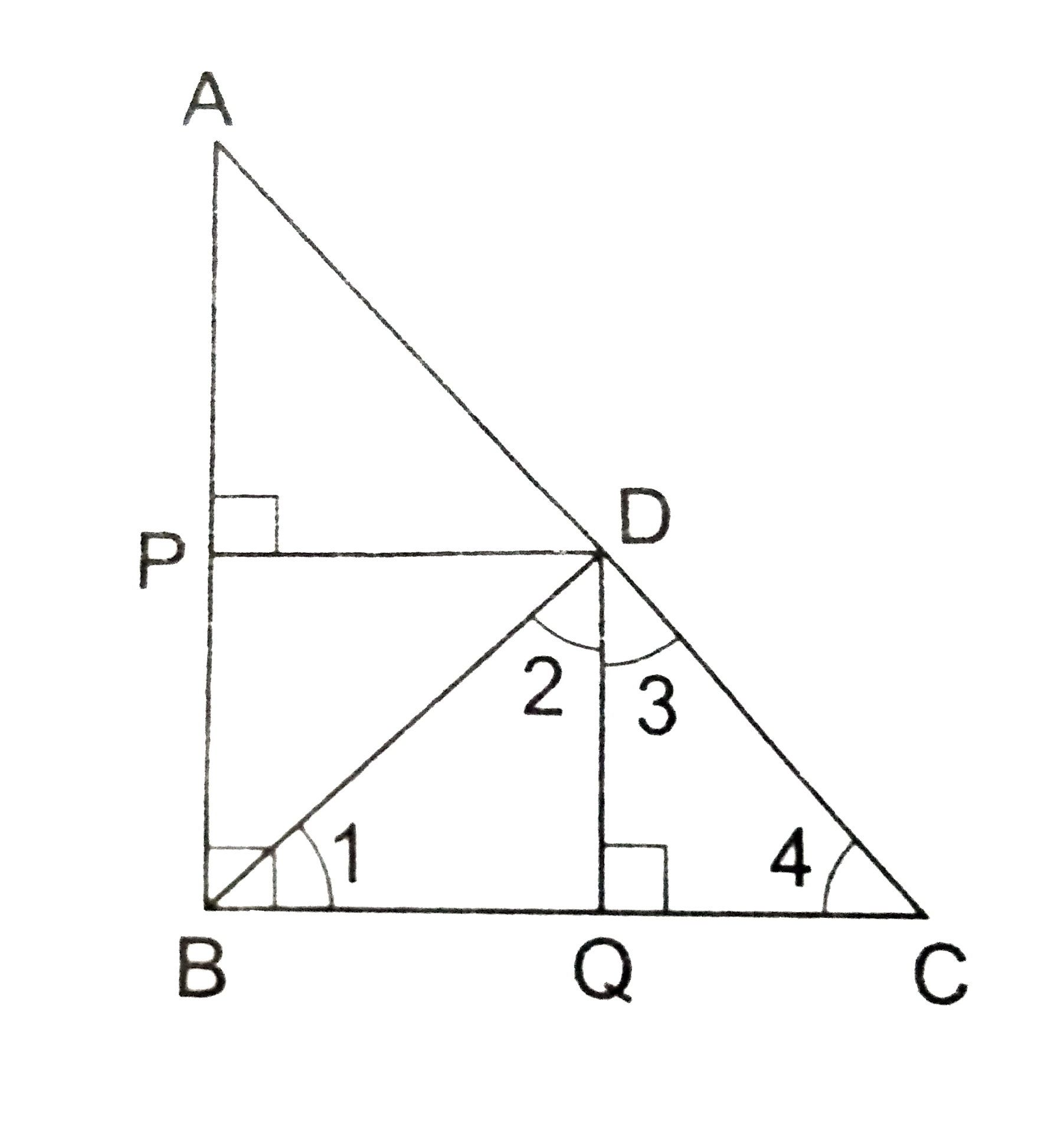
Ib rt. ΔDQC:∠3=∠4=90∘ ...(i)
In rt. ΔBDC:∠2+∠3=90∘ ...(iii)[∴BDC=90∘]
From (i) and (ii), we get ∠1=∠3
From (ii)and (ii), we get ∠2=∠4
∴ΔBQD~ΔDQC [ by AA- similarity]
And so, BQDQ=DQQC⇒PQ2=BQ⋅QC⇒DQ2=DP⋅QC[∴BQ=DP]
(b) By proving ΔPDA~ΔPBD ( in a similar way), we get
PDPB=APDP⇒DP2=BP⋅AP⇒DP2=DQ⋅AP [∴BP=DQ]
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7C13videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7D22videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7A12videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 1127videos
T-RATIOS OF SOME PARTICULAR ANGLES
- BOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself20videos
TRIGONOMETRIC IDENTITIES
Similar Questions
ABC is a triangle right angled at B. D is a point on AC such that ∠ABD=45∘ If AC=6 and AD=2 then AB=
View SolutionA Δ ABC is right angled at A. L is a point on BC such that AL⊥ BC. Prove that ∠BAL=∠ACB.
View SolutionIn a right ABC right-angled at C , if D is the mid-point of BC , prove that BC2=4 (AD2−AC2) .
View SolutionTriangle ABC is right angle at A. The points P and Q are on hypotenuse BC such that BP=PQ=QC.if AP=3andAQ=4, then length BC is equal to
View SolutionABC is right angle triangle where AD is perpendicular on hypotenuse BC. If AC = 2 AB find BD.
View SolutionIn a right triangle ABC, right angled at A, AD is drawn perpendicular to BC. Prove that:
AB2−BD2=AC2−CD2View SolutionIn ΔABC,DandE two points on AB such that AD=BE. If DP||BCandEQ||AC, prove that such PQ∣∣AB.
 View Solution
View SolutionΔABD is a right triangle, right-angled at A and AC⊥BD. Prove that AB2=BC×BD.
View SolutionABC is a right triangle with AB = AC.If bisector of ∠A meet BC at D then prove that BC =2 AD .
View SolutionABC is a triangle in which AB=ACandD is a point on AC such that BC2=AC×CD.Prove that BD=BC.
View Solution

