A game of chance consists of spinning an arrow, which comes to rest pointing at one of the numbers 1,2,3,4,5,6,7,8 and these are equally likely outcomes. Find the probability that the arrow will point at any factor of 8.
58
16
17
18
Step by step video & image solution for A game of chance consists of spinning an arrow, which comes to rest pointing at one of the numbers 1,2,3,4,5,6,7,8 and these are equally likely outcomes. Find the probability that the arrow will point at any factor of 8. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER - 11EXERCISE - SECTION-C10videos
SAMPLE PAPER - 11
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER - 11EXERCISE - SECTION-C10videos
SAMPLE PAPER - 11
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER (SELF ASSESSMENT) 12EXERCISE - PART-B (Section-V)4videos
SAMPLE PAPER (SELF ASSESSMENT) 12
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER - 3EXERCISE - SECTION-C10videos
SAMPLE PAPER - 3
Similar Questions
A game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1,2 3,4,5,6,7,8 and these are equally likely outcomes what is the probability that it will point at :
(i) 8?
(ii) an odd number ?
(iii) a number greater than 2 ?
(iv) a number less than 9 ?View SolutionA game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (See Fig. 131), and these are equally likely outcomes. What is the probability that it will point at (i) 8? (ii) an odd number? (iii) a number greater than 2? (iv) a number less than 9?
View SolutionA game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (see figure) and these are equally likely outcomes. What is the probability that it will point at :
(i) 8? (ii) an odd number?
(iii) a number greater than 2? (iv) a number less 9?View SolutionA game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1,2,3,4,5,6,1,8 (see Figure), and these are equally likely outcomes. What is the probability that it will point at(i) 8?(ii) an odd number?(iii) a number greater than 2?(iv) a number less than 9?
View SolutionRead the following text and answer the following question on the basis of the same.
A game of chances consisting of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (see the figure) and these are equally likely outcomes.
Find the probability that point lie on the number 8.View SolutionRead the following text and answer the following question on the basis of the same.
A game of chances consisting of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (see the figure) and these are equally likely outcomes.
What is the probability that it will point at a number greater than 2?View SolutionRead the following text and answer the following question on the basis of the same.
A game of chances consisting of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (see the figure) and these are equally likely outcomes.
Find out the probability that the pointer is always less than 9.View SolutionA game of chance consist of spinning an arrow which comes to rest pointing at one of the numbers 15,20,25,30,35,40,45,50 and these are equally likely outcomes. What is the probability that it will point at
(i) an even number? (ii) at a number multiple of 5
(iii) at a number divisible by 3 (iv) at a prime number.View SolutionRead the following text and answer the following question on the basis of the same.
A game of chances consisting of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (see the figure) and these are equally likely outcomes.
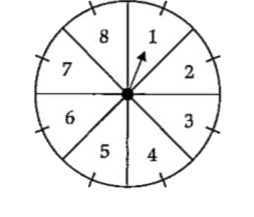
What is the probability that arrow point lies on the odd number?View SolutionIn a game of chance, a spining arrow comes to rest at one of the numbers
1,2,3,4,5,6,7,8,
All these are equally likely outcomes.
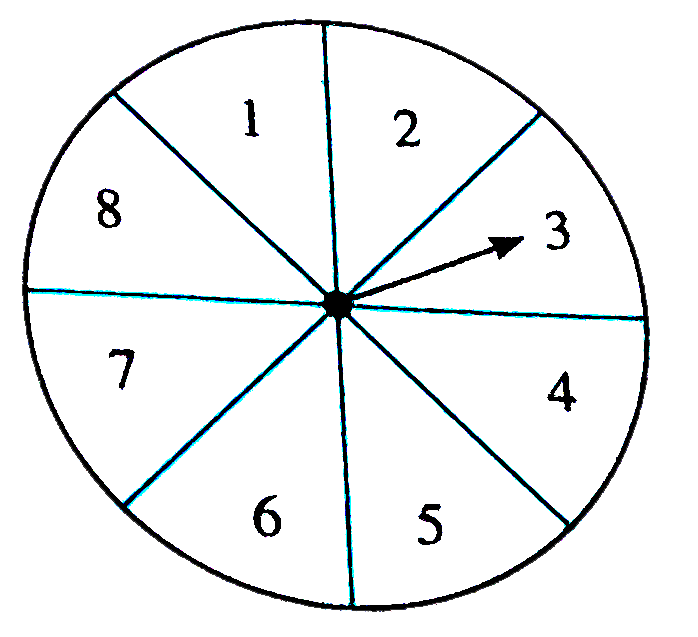
Find the probability that it will rest atView Solution

