Question

A lamp is 3.3 m be the lamp post and CD= 110 cm tall walks away from the base of this lamp post at a speed of 0.8 m/s. find the length of the shadow of boy after 4 seconds.

Explanation

To solve the problem step by step, we will follow these instructions:

Step 1: Convert the height of the boy from centimeters to meters
The height of the boy is given as 110 cm. To convert this to meters:
\( \text{Height of the boy (CD)} = \frac{110 \text{ cm}}{100} = 1.1 \text{ m} \)

Hint: Remember that 1 meter equals 100 centimeters, so you can convert centimeters to meters by dividing by 100.

Step 2: Determine the distance the boy walks in 4 seconds
The boy walks away from the lamp post at a speed of 0.8 m/s. To find out how far he walks in 4 seconds, use the formula:
\( \text{Distance} = \text{Speed} \times \text{Time} \)
Substituting the values:
\( \text{Distance} = 0.8 \text{ m/s} \times 4 \text{ s} = 3.2 \text{ m} \)

Hint: Use the formula for distance, which is speed multiplied by time, to find out how far the boy has walked.

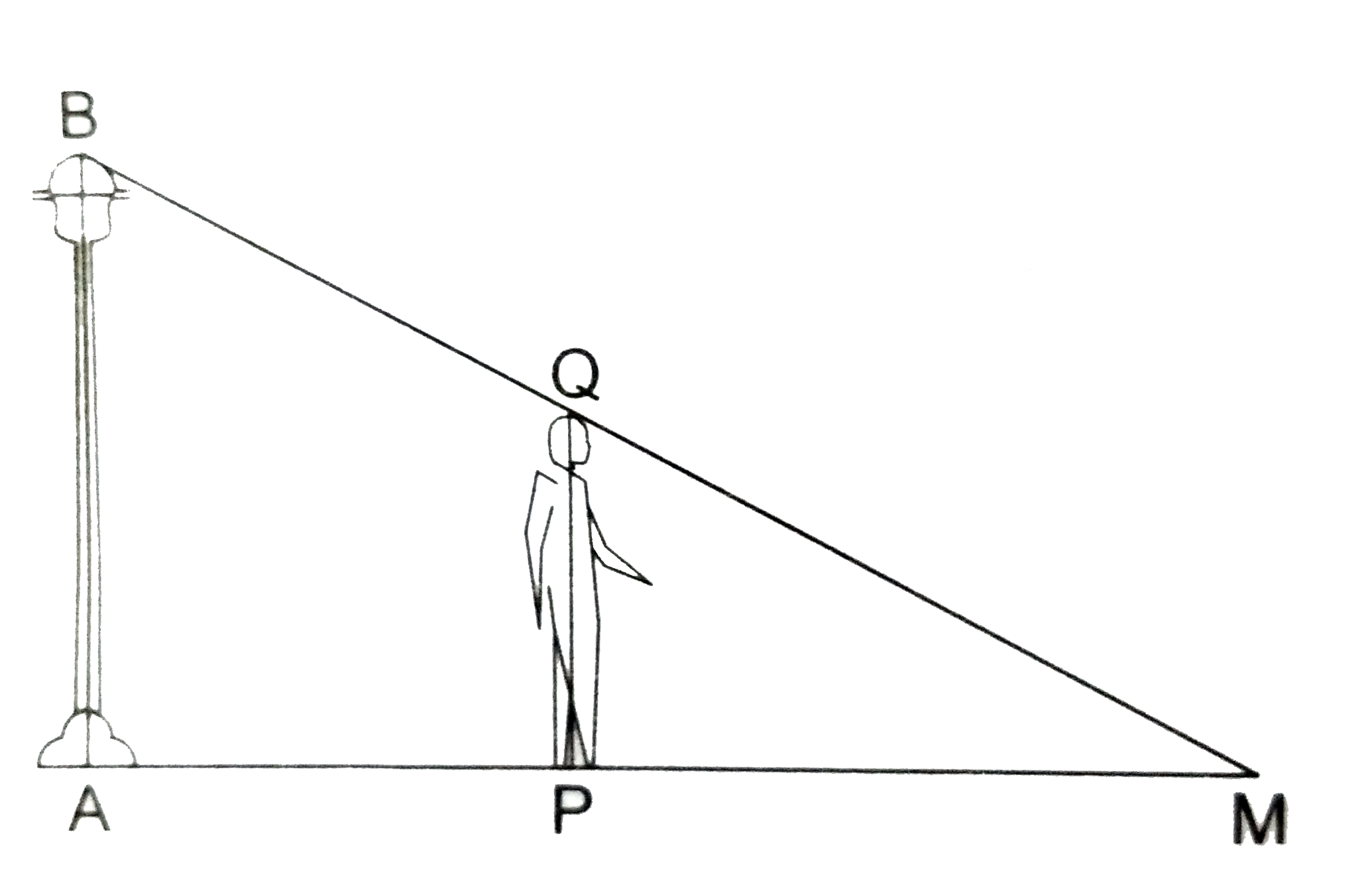
Step 3: Set up the proportions using similar triangles
We have two similar triangles: Triangle ABE (formed by the lamp post and the shadow) and Triangle CDE (formed by the boy and his shadow). The heights of the triangles and their bases can be expressed as:
- Height of the lamp post (AB) = 3.3 m
- Height of the boy (CD) = 1.1 m
- Let the length of the shadow be \( X \) and the distance from the lamp post to the boy be \( Y \).

From the similar triangles, we can set up the proportion:
\( \frac{AB}{CD} = \frac{X + Y}{Y} \)
Substituting the known values:
\( \frac{3.3}{1.1} = \frac{X + 3.2}{3.2} \)

Hint: When dealing with similar triangles, the ratios of corresponding sides are equal. Set up a proportion to relate the heights and bases.

Step 4: Simplify the proportion
First, simplify \( \frac{3.3}{1.1} \):
\( \frac{3.3}{1.1} = 3 \)
Now, we can write:
\( 3 = \frac{X + 3.2}{3.2} \)

Hint: Simplifying fractions can help make calculations easier. Look for common factors.

Step 5: Cross-multiply to solve for \( X \)
Cross-multiplying gives:
\( 3 \times 3.2 = X + 3.2 \)
Calculating the left side:
\( 9.6 = X + 3.2 \)

Hint: Cross-multiplying can help eliminate fractions and make it easier to isolate the variable.

Step 6: Solve for \( X \)
Now, isolate \( X \):
\( X = 9.6 - 3.2 = 6.4 \text{ m} \)

Hint: To isolate a variable, perform the same operation on both sides of the equation.

Step 7: Calculate the length of the shadow
Since \( Y \) (the distance the boy walked) is 3.2 m, we need to find the length of the shadow, which is \( X \):
\( \text{Length of the shadow} = X = 6.4 \text{ m} \)

Hint: The length of the shadow can be found directly from the variable you calculated.

Final Answer
The length of the shadow of the boy after 4 seconds is 6.4 meters.