AD is a median of △ABC. The bisectors of ∠ADBand∠ADC meets AB and AC in E and F respectively prove that EF||BC.
Step by step video, text & image solution for AD is a median of triangleABC. The bisectors of angleADB and angleADC meets AB and AC in E and F respectively prove that EF||BC. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Revision Exercise Short Questions10videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions5videos
STATISTICS
- BOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
Knowledge Check
- Question 1 - Select One
In a ΔABC, AD is a median . The bisectors of ∠ADBand∠ADC meet AB and AC at E and F respectively . If the ratio of AE : BE =2:3 , then find the ratio of EF : BC.
A2:5B4:3C7:3D3:7 - Question 2 - Select One
In a ΔABC,∠C in an obtuse angle . The bisectors of the exterior angles at A and B meet BC and AC produced at D and E respectively . If AB=AD=BE. Find ∠ACB .
A105∘B108∘C110∘D135∘ - Question 3 - Select One
In ΔABC,∠C=70∘ . The bisectors of ∠Aand∠B meet BC and AC in points D and E . Respectively .AD and BE intersect each other at P. What is the measure of ∠DPB?
A55∘B65∘C35∘D45∘
In ΔABC,AB=AC and the bisectors of ∠B and ∠C meet AC and AB at point D and E respectively. Prove that BD=CE.
View SolutionIn △ABCthe bisector of ∠B meets AC at D. A line PQ||AC meets AB,BC and BD at P, Q and R respectively.
show that PR×BQ=QR×BP.
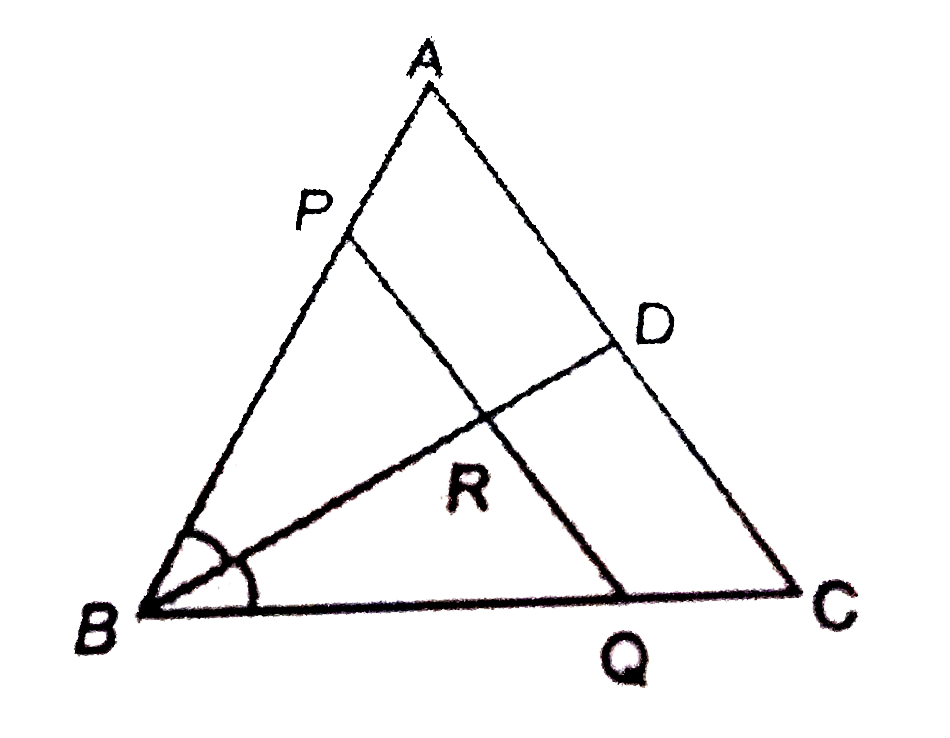 View Solution
View SolutionIn a ΔABC,∠C in an obtuse angle . The bisectors of the exterior angles at A and B meet BC and AC produced at D and E respectively . If AB=AD=BE. Find ∠ACB .
View SolutionThe side BC of a triangle ABC is bisected at D;o is any point in AD,BOandCO produced meet ACandAB in EandF respectively and AD is produced to X so that D is the mid-point of OX. Prove that AO:AX=AF:AB and show that FEBC.
View SolutionThe median AD of the triangle ABC is bisected at E and BE meets AC at F. Find AF:FC.
View Solution

