A line L intersects three sides BC, CA and AB of a triangle in P,Q,R respectively, show that BPPC.CQQA.ARRB=−1
Step by step video & image solution for A line L intersects three sides BC, CA and AB of a triangle in P,Q,R respectively, show that (BP)/(PC).(CQ)/(QA).(AR)/(RB)=-1 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- COORDINATE SYSTEM AND COORDINATES Book:ARIHANT MATHS ENGLISHChapter:COORDINATE SYSTEM AND COORDINATES Exercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 7 Videos
- COORDINATE SYSTEM AND COORDINATES Book:ARIHANT MATHS ENGLISHChapter:COORDINATE SYSTEM AND COORDINATES Exercise:EXERCISE : 7
Explore 4 Videos
- CONTINUITY AND DIFFERENTIABILITYBook:ARIHANT MATHS ENGLISHChapter:CONTINUITY AND DIFFERENTIABILITYExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 20 Videos
- DEFINITE INTEGRALBook:ARIHANT MATHS ENGLISHChapter:DEFINITE INTEGRALExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 38 Videos
Similar Questions
A(x1,y1),B(x2,y2),C(x3,y3) are three vertices of a triangle ABC, lx+my+n=0 is an equation of line L. If L intersects the sides BC,CA and AB of a triangle ABC at P,Q,R respectively, then BPPC×CQQA×ARRB is equal to
View SolutionO is any point in the plane of the triangle ABC,AO,BO and CO meet the sides BC,CA nd AB in D,E,F respectively show that ODAD+OEBE+OFCF=1.
View Solution
Knowledge Check
In a triangle ABC, the incircle (centre O) touches BC , CA and AB at points P,Q and R respectively. Calculate:
(i) ∠QOR (ii) ∠QPR
given that ∠A=60∘A(i) 120∘ (ii) 60∘B(i) 110∘ (ii) 50∘C(i) 145∘ (ii) 80∘D(i) 90∘ (ii) 40∘
P and Q are respectively the mid-points of sides AB and BC of a triangle ABC and Ris the mid-point of AP, show that (i) ar(PRQ)=12ar(ARC)(ii) ar(RQC)=38ar(ABC)(iii) ar(PBQ)=ar(ARC)
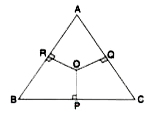
View SolutionIn the following figure, OP, OQ and OR are drawn perpendiculars to the sides BC, CA and AB repectively of triangle ABC. Prove that :
AR2+BP2+CQ2=AQ2+CP2+BR2
View SolutionLet A, B, C respresent the vertices of a triangle, where A is the origin and B and C have position b and c respectively. Points M, N and P are taken on sides AB, BC and CA respectively, such that (AM)/(AB)=(BN)/(BC)=(CP)/(CA)=alpha Q. AN+BP+CM is
View SolutionThrough A, B and C lines RQ, PR and QP have been drawn, respectively parallel to sides BC, CA and AB of a ΔABC as shown in figure. Show that BC=12QR.

View SolutionLet A, B, C represent the vertices of a triangle, where A is the origin and B and C have position b and c respectively.* Points M, N and P are taken on sides AB, BC and CA respectively, such that AMAB=BNBC=CPCA=α . If △ represent the area enclosed by the three vectors AN, BP and CM, then the value of α, for which △ is least
View SolutionThe midpoints of the sides BC, CA and AB of a △ABC are D(2,1), E(-5,7)and F(-5, -5) respectively. Find the equations of the sides of △ABC.
View SolutionIn the following diagram, lines l, m and n are parallel to each other. Two transversals p and q intersect the parallel lines at points A, B, C and P, Q, R as shown.
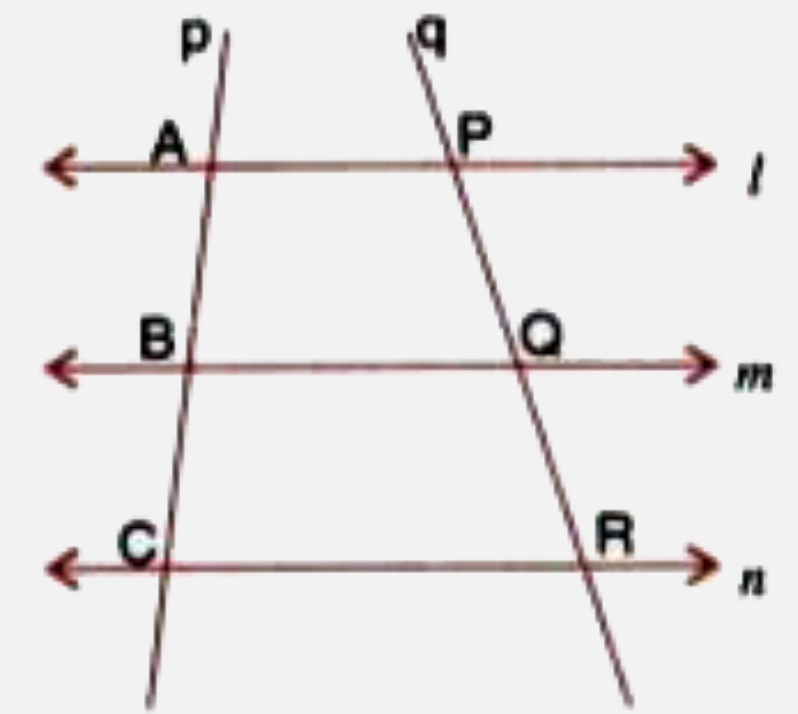
Prove that : ABBC=PQQR
Join A and R. Let AR meets BQ at point D.
View Solution