A line segment AB is trisected (divided into three equal parts) by pounts C and D .Are line segments AC,CD.DB congruent ?

The correct Answer is:Points C and D trisect the line segment AB. Thus ,AC=CD=DB. Since line segments of equal length are congruent , AC,CD, and DB are congruent.
Topper's Solved these Questions
Similar Questions
Draw a line segment of length 8 cm . Divide it into three equal parts .
View SolutionIs line a part of line segments ?
View SolutionFind the coordinates of the points of trisection (i.e., points dividing in three equal parts) of the line segment joining the points A(2,2) and B(7,4).
View SolutionFind the coordinates of the points of trisection (i.e., points dividing in three equal parts) of the line segment joining the points A(2,2) and B(7,4).
View SolutionDraw a line segment EF equal to a given line segment AB
View SolutionThe points M and N divide the line seqment AB of ΔABC in three equal parts. If MP||NQ||BC and points P and Q lie on line AC, then prove that P and Q trisect the line AC.
View SolutionThe length of the a line segment AB is 2 units. It is divided into two parts at the point C such that AC2=AB×CB. What is the length of CB ?
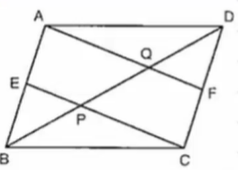
View SolutionIn Figure, ABCD is a parallelogram. E and F are the mid-points of the sides AB and CD respectively. Prove that the line segments AF and CE trisect (divide into three equal parts) the diagonal BD .
View SolutionIn Figure, ABCD is a parallelogram. E and F are the mid-points of the sides AB and CD respectively. Prove that the line segments AF and CE trisect (divide into three equal parts) the diagonal BD .
View SolutionIn Figure, ABCD is a parallelogram. E and F are the mid-points of the sides AB and CD respectively. Prove that the line segments AF and CE triset (divide into three equal parts) the diagonal BD.
View Solution
