Question

A man observes when he has climbed up ${\displaystyle \frac{1}{3}}$
of the length of an inclined ladder, placed against a wall, the angular
depression of an object on the floor is ${\displaystyle \alpha \stackrel{.}{}}$
When he climbs the ladder completely, the angleof depression is ${\displaystyle \beta }$
. If the inclination of the ladder to the floor is ${\displaystyle \theta ,}$
then prove that ${\displaystyle \cot \theta =\frac{3\cot \beta -\cot \alpha }{2}}$

Explanation

Let AB be the ladder and the object be ay point O.

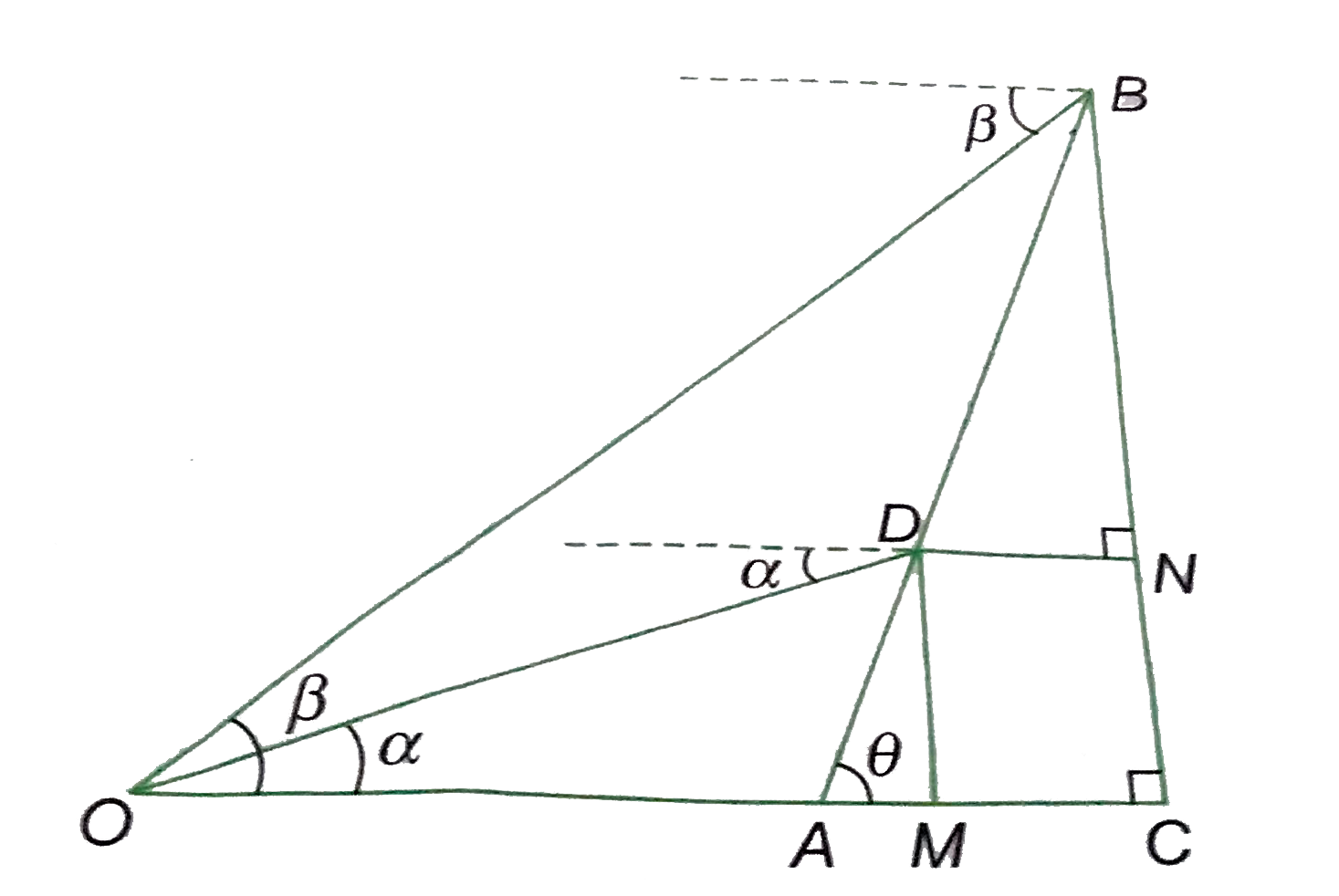
Also ,let l length of the ladder and the man be at point D intitially .
In triangle AMD, DM = AD sin `theta=1/3lsin theta`
In triangle ACB,BC `=l sin theta`
In triangle OMD, OM `= DM cot alpha = 1/3lsinthetacotalpha`
In triangle OCB, OC = BC cot `beta = l sin theta cot beta`
Now ,MC = OC - OM = l sin `theta cot beta - 1/3 l sin theta cot alpha .....(1)`
Also , MC = DN `=2/3 l cos theta ` (From triangle BND)
`therefore l sin theta cot beta - 1/3 l sin theta alpha = 2/3 l cos theta `
`rArr ( 3 cot beta - cot alpha ) sin theta = 2 cos theta `
`rArr cot theta = ( 3 cot beta - cot alpha )/(2)`