A manufacturing companu makes two types of teaching aids A and B of Mathematics for Class X. Each type of A requires 9 labour hours for fabircating and 1 labour for finishing. Each type of B requires 12 labour hours for fabircating and 3 labour hours for finishing. For fabricating and finishing, the maximum labour hours available per week are 180 and 30 respectively. The company makes a profit of 80 on each piece of type A and 120 on each piece of type B. How many pieces of type A and types B should be manufactured per week to get a maximum profit? Formulae this as Linear Programming Problems and solved it. Identify the feasible region from the rough sketch.
The correct Answer is:1680 per weak
To solve the problem of maximizing the profit from manufacturing teaching aids A and B, we will formulate it as a Linear Programming Problem (LPP) and solve it step by step.
Step 1: Define the Variables
Let:
- X = number of pieces of type A manufactured
- Y = number of pieces of type B manufactured
Step 2: Formulate the Objective Function
The profit from each piece of type A is 80 and from type B is 120. Therefore, the objective function to maximize profit Z is given by:
Z=80X+120Y
Step 3: Formulate the Constraints
From the problem, we have the following constraints based on labor hours for fabricating and finishing:
1. Fabricating Constraint:
Each type A requires 9 hours and each type B requires 12 hours for fabricating. The total available hours for fabricating is 180.
9X+12Y≤180
Simplifying this gives:
3X+4Y≤60(Constraint 1)
2. Finishing Constraint:
Each type A requires 1 hour and each type B requires 3 hours for finishing. The total available hours for finishing is 30.
1X+3Y≤30
This remains as:
X+3Y≤30(Constraint 2)
3. Non-negativity Constraints:
Since the number of pieces cannot be negative, we have:
X≥0,Y≥0
Step 4: Graph the Constraints
To find the feasible region, we need to graph the constraints.
1. For Constraint 1: 3X+4Y=60
- If X=0: 4Y=60 → Y=15 (Point: (0, 15))
- If Y=0: 3X=60 → X=20 (Point: (20, 0))
2. For Constraint 2: X+3Y=30
- If X=0: 3Y=30 → Y=10 (Point: (0, 10))
- If Y=0: X=30 (Point: (30, 0))
Step 5: Identify the Feasible Region
Plotting these points on a graph will show the feasible region bounded by the lines of the constraints. The intersection points of the lines will also be calculated.
Step 6: Find Intersection Points
To find the intersection of the two lines:
1. From 3X+4Y=60
2. From X+3Y=30
Substituting X=30−3Y into the first equation:
3(30−3Y)+4Y=60
90−9Y+4Y=60
−5Y=−30⇒Y=6
Substituting Y=6 back into X+3Y=30:
X+3(6)=30⇒X+18=30⇒X=12
Thus, the intersection point is (12,6).
Step 7: Evaluate the Objective Function at Corner Points
The corner points of the feasible region are:
1. (0,0)
2. (0,10)
3. (12,6)
4. (20,0)
Calculating Z at each corner:
1. Z(0,0)=80(0)+120(0)=0
2. Z(0,10)=80(0)+120(10)=1200
3. Z(12,6)=80(12)+120(6)=960+720=1680
4. Z(20,0)=80(20)+120(0)=1600
Step 8: Conclusion
The maximum profit occurs at the point (12,6), meaning the company should manufacture:
- 12 pieces of type A
- 6 pieces of type B
Let:
- X = number of pieces of type A manufactured
- Y = number of pieces of type B manufactured
The profit from each piece of type A is 80 and from type B is 120. Therefore, the objective function to maximize profit Z is given by:
Z=80X+120Y
From the problem, we have the following constraints based on labor hours for fabricating and finishing:
Each type A requires 9 hours and each type B requires 12 hours for fabricating. The total available hours for fabricating is 180.
9X+12Y≤180
Simplifying this gives:
3X+4Y≤60(Constraint 1)
Each type A requires 1 hour and each type B requires 3 hours for finishing. The total available hours for finishing is 30.
1X+3Y≤30
This remains as:
X+3Y≤30(Constraint 2)
Since the number of pieces cannot be negative, we have:
X≥0,Y≥0
To find the feasible region, we need to graph the constraints.
- If X=0: 4Y=60 → Y=15 (Point: (0, 15))
- If Y=0: 3X=60 → X=20 (Point: (20, 0))
- If X=0: 3Y=30 → Y=10 (Point: (0, 10))
- If Y=0: X=30 (Point: (30, 0))
Plotting these points on a graph will show the feasible region bounded by the lines of the constraints. The intersection points of the lines will also be calculated.
To find the intersection of the two lines:
1. From 3X+4Y=60
2. From X+3Y=30
3(30−3Y)+4Y=60
90−9Y+4Y=60
−5Y=−30⇒Y=6
Substituting Y=6 back into X+3Y=30:
X+3(6)=30⇒X+18=30⇒X=12
Thus, the intersection point is (12,6).
The corner points of the feasible region are:
1. (0,0)
2. (0,10)
3. (12,6)
4. (20,0)
1. Z(0,0)=80(0)+120(0)=0
2. Z(0,10)=80(0)+120(10)=1200
3. Z(12,6)=80(12)+120(6)=960+720=1680
4. Z(20,0)=80(20)+120(0)=1600
The maximum profit occurs at the point (12,6), meaning the company should manufacture:
- 12 pieces of type A
- 6 pieces of type B
Topper's Solved these Questions
- QUESTION PAPER-2018BOOK - ICSECHAPTER - QUESTION PAPER-2018EXERCISE - Section -B
8 Videos
- QUESTION PAPER 2022 TERM 1BOOK - ICSECHAPTER - QUESTION PAPER 2022 TERM 1EXERCISE - SECTION C
8 Videos
- RELATIONS AND FUNCTIONS BOOK - ICSECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - MULTIPLE CHOICE QUESTIONS (Competency based questions)
20 Videos
Similar Questions
Find λ if the scalar projection of →a=λˆi+ˆj+4ˆk on →b=2ˆi+6ˆj+3ˆk is 4 units.
View SolutionThe cost function of a firm is given by C(x)=1500+25x+x210 . Then the marginal cost of the firm MC(x) will be :
View SolutionThe price of the oranges in the market is dependent on the amount of oranges (in kgs) which can be represented as y = 3x + 5. Reema went to buy the oranges for a family function in her house. The total number of oranges she wants to buys is 5≤x≤10 according to her assumption of people coming to the party. Answer the following questions on the basis of the given information.
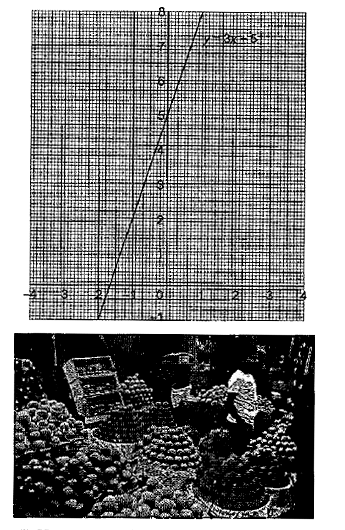
How many ordered pairs can be represented for the equation y=3x+5 for ≤x≤x10 ?
View Solution(Manufacturing problem) A manufacturing company makes two models A and B of a product. Each piece of Model A requires 9 labour hours for fabricating and 1 labour hour for finishing. Each piece of Model B requires 12 labour hours for fabricating and 3 labour hours for finishing. For fabricating and finishing, the maximum labour hours available are 180 and 30 respectively. The company makes a profit of Rs 8000 on each piece of model A and Rs 12000 on each piece of Model B. How many-pieces of Model A and Model B should be manufactured per week to realise a maximum profit? What is the maximum profit per week?
View Solution30 labourers working 7 hours a day can finish a piece of work in 18 days. If the labourers work 6 hours a day, then the number of labourers to finish the same piece of work in30 days, will be: 15 b. 21 c. 22 d. 25
View SolutionA manufacturing company makes two types of teaching aids A and two types of teaching aids A and B of mathematics. Each type of A requires a labour hours of fabricating and 1 labour hour for finish I fabricating and 1 labour hour for finishing. Each type of B requires 12 hours for fabricating and 3 labour hours for finishing. For fabricating and finishing, the maxim finishing. For fabricating and finishing, the maximum labour hours available per week are 180 and 30 respectively. The company makes a profit of 80 on each piece oA and 120 on each piece of type B. How many pieces of type A and type B should be manufactured per
View SolutionA manufacturing company makes two types of teaching aids A and B . Each type of A requires 9 labour hours for fabricating and 1 labour hours for finishing.Each type of B requires 12 labour hours for fabricating and 3 labour hours for finishing.For fabricating and finishing, the maximum labour hours abailable per week are 180 and 30 respectively.The company makes a profit of ₹ 80 on each type A and ₹ 120 on each type B. How many pieces of type A and type B should be manufactured per week to get a maximum profit? Make it as an LPP and solve graphically.What is the maximum profit per week?
View SolutionA manufacturer produces two models A and B of a product. Each piece of model A requires 9 labour hours for fabricating and 1 labour hour for finishing. Each piece of model B requires 12 Labour hours for fabricating and finishing the maximum labour hours available are 180 and 30 respectively. The company makes a profit of Rs 8000 on each piece of model A and Rs 12000 on each piece of model B . Formulate an L.P.P. So as to maximize his profit.
View SolutionA manufacturer produces two models A and B of a product each piece of model A requires 9 labour hours for fabricating and 1 labour hours for fabricating and 3 labour hour for for finishing for fabnricating and finishing the maximum labour hours available are 180 and 30 respectively the company makes a profit of Rs 8000 on each piece of model A and Rs 12000 on each piece of model B formulate a LPP so as to maxmize his profit per week and solve the problem graphically
View SolutionA manufacturing company makes two types of teaching aids A and B . Each type of A requires 9 labour hours for fabricating and 1 labour hours for finishing.Each type of B requires 12 labour hours for fabricating and 3 labour hours for finishing.For fabricating and finishing, the maximum labour hours abailable per week are 180 and 30 respectively.The company makes a profit of ₹ 80 on each type A and ₹ 120 on each type B. How many pieces of type A and type B should be manufactured per week to get a maximum profit? Make it as an LPP and solve graphically.What is the maximum profit per week?
View SolutionA manufacturing company makes tow models A and B of product. Each piece of model A reqwuires 9 labour hours for fabricating and 1 hour labour for finishing . Each piece of model Brequires 12 labour hours for fabricating and 3 labour hours for fininshing. for fabricating and finishing, the maximum labour hours available are 180 and 30 respectively. The company makes a profit of Rs. 8,000 on each piece of modle A and Rs 12,000 on each piece of model B. How many pieces of model A and B should be manufactured per week to realise a maximum profit ? What is the maximum profit per week ?
View Solution(Manufacturing problem): A manufacturing company makes two models A and B of a product. Each piece of Model A requires 9 labours for fabricating and 1 labour hour for finishing. Each piece of Model B requires 12 labour hours for fabricating and 3 labour hours for finishing. For fabricating and finishing the maximum labour hors available are 180 and 30 respectively. The company makes a profit of Rs. 8000 on each piece of model Aand Rs. 12000 on each piece of Model B. How many piecesof Model A and Model B should be manufactured per week to realise a maximum profit? What is the maximum profit per week?
View Solution(Manufacturing problem): A manufacturing company makes two models A and B of a product. Each piece of Model A requires 9 labours for fabricating and 1 labour hour for finishing. Each piece of Model B requires 12 labour hours for fabricating and 3 labour hours for finishing. For fabricating and finishing the maximum labour hors available are 180 and 30 respectively. The company makes a profit of Rs. 8000 on each piece of model Aand Rs. 12000 on each piece of Model B. How many piecesof Model A and Model B should be manufactured per week to realise a maximum profit? What is the maximum profit per week?
View SolutionA manufacturing company makes two modeis A and B of a product. Each piece of model A requires 9 labour hours for fabricating and 1 labour hour for finishing. Fach piece of model B requires 12 labour hours for fabricating and 3 labour hours for finishing. For fabricating and finishing, the maximum labour hours available are 180 and 30 respectively. The company makes a profit of Rs. 8,000 on each piece of modal A and Rs. 12,000 on week maximum profit ? What is the maximum profit per week ?
View Solution(Manufacturing problem) A manufacturing company makes two models A and B of a product. Each piece of Model A requires 9 labour hours for fabricating and 1 labour hour for finishing. Each piece of Model B requires 12 labour hours for fabricating and 3 labour hours for finishing. For fabricating and finishing, the maximum labour hours available are 180 and 30 respectively. The company makes a profit of Rs 8000 on each piece of model A and Rs 12000 on each piece of Model B. How many-pieces of Model A and Model B should be manufactured per week to realise a maximum profit? What is the maximum profit per week?
View SolutionA manufacturing company makes two models A and B of a product. Each piece of mode a requires 9 labour hours for fabricating and 1 labour hour for finishing. Each piece of Mode B requires 12 labour hours for fabricating and 3 labour hours for finishing. For fabricati2 and finishing, the maximum labour hours available are 180 and 30 respectively. The company makes a profit of Rs. 8000 on each piece of model A and Rs. 12000 on each piece of Model B. How many pieces of Model A and Model B should be manufactured per week to realise a maximum profit? What is the maximum profit per week?
View Solution
