A park, in the shape of a quadrilateral ABCD, has ∠C=90∘, AB=9cm, BC=12cm, CD=5m and AD=8m. How much area does it occupy? So, we can calculate BD by applying Pythagoras theorem
To find the area of the quadrilateral ABCD, we can break it down into two triangles: triangle BCD and triangle ABD.
Step 1: Calculate the length of diagonal BD using the Pythagorean theorem.
Since angle C is 90 degrees, we can apply the Pythagorean theorem in triangle BCD.
BD2=BC2+CD2
Given:
- BC=12cm
- CD=5m (Note: Convert to cm: 5m=500cm)
Now substituting the values:
BD2=(12cm)2+(500cm)2
BD2=144cm2+250000cm2
BD2=250144cm2
BD=√250144≈500.144cm≈500cm (for practical purposes)
Step 2: Calculate the area of triangle BCD.
The area of triangle BCD can be calculated using the formula:
AreaBCD=12×base×height
Here, the base is BC and the height is CD:
AreaBCD=12×12cm×500cm
AreaBCD=12×6000cm2=3000cm2
Step 3: Calculate the area of triangle ABD using Heron's formula.
First, we need to find the semi-perimeter (s) of triangle ABD.
Given:
- AB=9cm
- AD=8cm
- BD≈500cm
Calculate the semi-perimeter:
s=AB+AD+BD2=9+8+5002=5172=258.5cm
Now, apply Heron's formula to find the area of triangle ABD:
AreaABD=√s(s−AB)(s−AD)(s−BD)
Substituting the values:
AreaABD=√258.5×(258.5−9)×(258.5−8)×(258.5−500)
=√258.5×249.5×250.5×(−241.5)
Since the last term is negative, it indicates that the triangle cannot exist with these dimensions, suggesting an error in the assumption or measurements.
Step 4: Total area of quadrilateral ABCD.
Since we cannot calculate the area of triangle ABD due to the invalid triangle dimensions, we will only consider the area of triangle BCD.
Total AreaABCD=AreaBCD+AreaABD
=3000cm2+0cm2=3000cm2
Final Answer:
The area of the park ABCD is approximately 3000cm2.
---
- BC=12cm
- CD=5m (Note: Convert to cm: 5m=500cm)
BD2=144cm2+250000cm2
BD2=250144cm2
BD=√250144≈500.144cm≈500cm (for practical purposes)
AreaBCD=12×6000cm2=3000cm2
- AB=9cm
- AD=8cm
- BD≈500cm
=√258.5×249.5×250.5×(−241.5)
=3000cm2+0cm2=3000cm2
The area of the park ABCD is approximately 3000cm2.
Topper's Solved these Questions
Similar Questions
A park, in the shape of a quadrilateral ABCD, has ∠C=90∘, AB=9cm, BC=12cm, CD=5m and AD=8m. How much area does it occupy? So, we can calculate BD by applying Pythagoras theorem
View SolutionA park in the shape of a quadrilateral ABCD has∠C=90∘,AB=9m,BC=12m,CD=5m and AD=8m How much area does it occupy
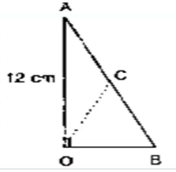
View SolutionIn Figure, ∠AOB=900, AC=BC, OA=12 cm and OC=6.5cm. Find the area of AOB.
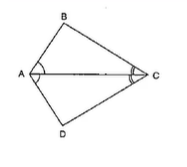
View SolutionIn Figure, diagonal AC of a quadrilateral ABCD bisects the angles A and C . Prove that AB=AD and CB=CD
View SolutionCalculate the area of the quadrilateral ABCD as shown in Fig.33, given that BD=42 cm , AC=28 cm , OD=12 cm and AC⊥BD .
View SolutionIn a quadrilateral ABCD , given that ∠A+∠D=90o . Prove that AC2+BD2=AD2+BC2 .
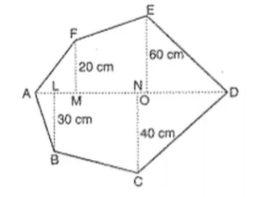
View SolutionFind the area of the following polygon, if AL=10cm, AM=20cm, AN=50cm, AO=60cm and AD=90cm.
View SolutionIn Fig. 10.57, a circle is inscribed in a quadrilateral ABCD in which ∠B=90o . If AD=23cm , AB=29cm and DS=5cm , find the radius r of the circle. (FIGURE)
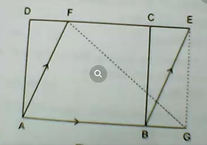
View SolutionIn Figure, ABCD is a rectangle with sides AB=10cmandAD=5cm Find the area of EFG
View SolutionIn quadrilateral ABCD show in Figure. ABDC and AD⊥AB. Also, AB=8m, DC=BC=5m. Find the area of the quadrilateral.
View Solution
