A particle moves so that s=6+48t-t3. The direction of motion reverses after moving a distance of
63
104
134
288
The correct Answer is:C
Step by step video, text & image solution for A particle moves so that s=6+48t-t^3. The direction of motion reverses after moving a distance of by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- APPLICATIONS OF DERIVATIVESBook:TARGET PUBLICATIONChapter:APPLICATIONS OF DERIVATIVESExercise:EVALUATION TEST
Explore 20 Videos
- APPLICATIONS OF DERIVATIVESBook:TARGET PUBLICATIONChapter:APPLICATIONS OF DERIVATIVESExercise:CRITICAL THINKING
Explore 129 Videos
- APPICATIONS OF DEFINITE INTEGRALBook:TARGET PUBLICATIONChapter:APPICATIONS OF DEFINITE INTEGRALExercise:EVALUATION TEST
Explore 18 Videos
- BINOMIAL DISTRIBUTIONBook:TARGET PUBLICATIONChapter:BINOMIAL DISTRIBUTIONExercise:EVALUTION TEST
Explore 12 Videos
Similar Questions
A particle is moving along the x-axis such that s=6t−t2, where s in meters and t is in second. Find the displacement and distance traveled by the particle during time interval t=0 to t=5s.
View SolutionFrom the v versus t graph of figure (a) the time (s) at which the particle is at rest (b) at what time, if any does the particle reverse the direction of its motion? (c) The distance and displacement of the particle from t=0s to t=6 s.
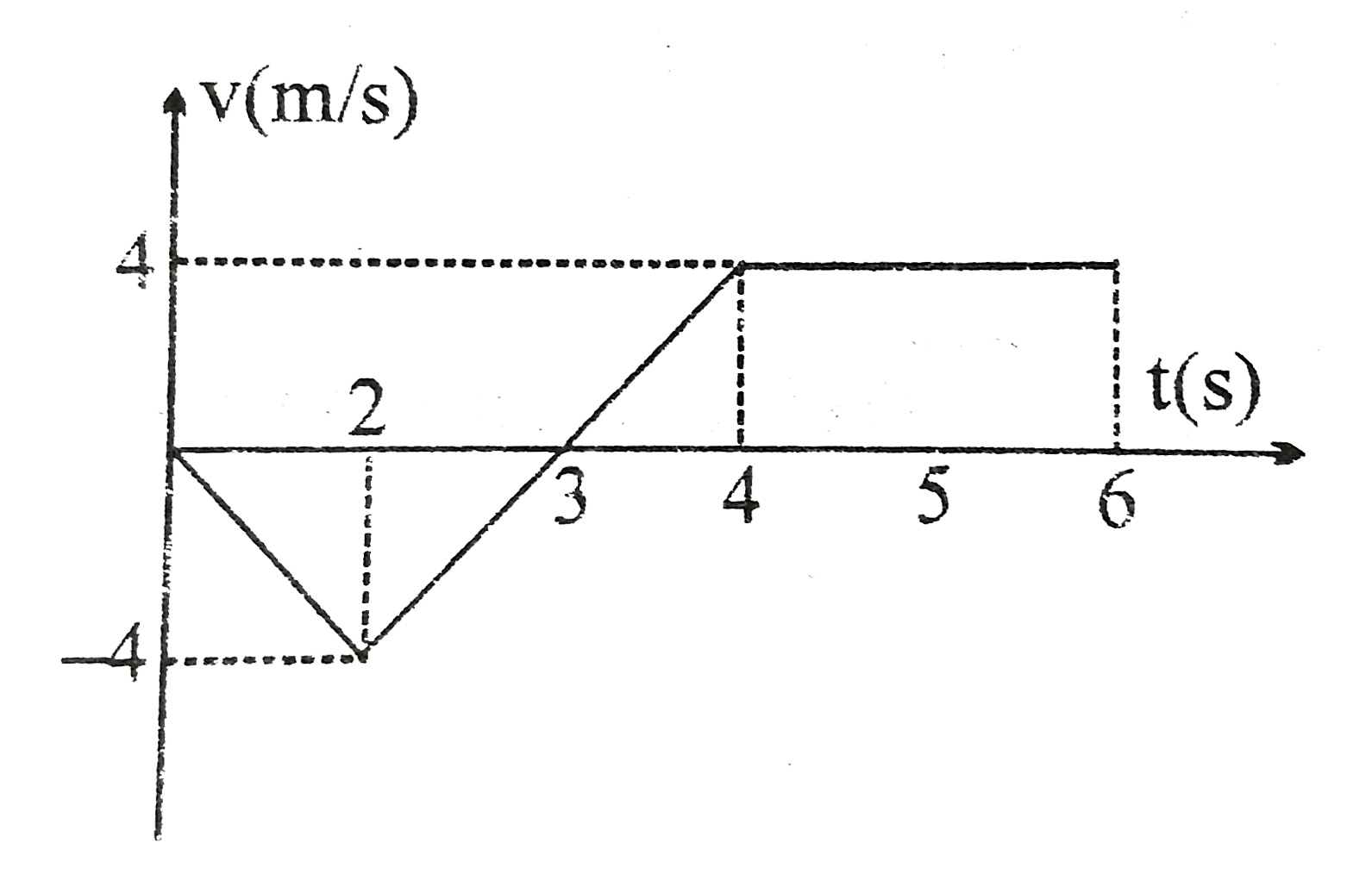
View Solution
Knowledge Check
A particle moves so that x=2+27t-t3. The direction of motion reverses after moving a distance of . . . Units.
A80B56C60D65
The position vector →r of a moving particle at time t after the start of the motion is given by →r=(5+20t)ˆi+(95+10t−5t2)ˆj. At the t = T, the particle is moving at right angles to its initial direction of motion. Find the value of T and the distance of the particle from its initial position at this time.
View SolutionThe po9sition of aparticle is given by the equation.
s=f(t)=t3−6t2−9t
where r is is measure in seconds and s in meters.
(a). Find the velocity at the t
(b). What is thhe velocity after 2s ? After 4 s ?
(c). When is the particle at rest ?
(d). When is the particle moving formward that is, ini the positive direction)?
(e). Find the total distance traveled by the particle during the first five sec.?
View SolutionA particle moves along the x-direction with constant acceleration. The displacement, measured from a convenient position, is 2 m at time t=0 and is zero when t=10s. If the velocity of the particle is momentary zero when t=6s, determine the acceleration a and the velocity v when t=10s.
View SolutionA particle moves along the X-axis. Its X-coordinate varies with time according to the expression x=4t+2r2, where x is in meters and t is in seconds. The position-time graph for this motion is shown in the figures previously. Note that the particle moves in the negative X-direction for the first second of motion, is at rest at the moment t=1s, and then heads back in the positive X-direction for t>1s.
Find the displacement of the particle in the time interval t=1sto t=3s.
View SolutionA particle moves along the X-axis. Its X-coordinate varies with time according to the expression x=4t+2r2, where x is in meters and t is in seconds. The position-time graph for this motion is shown in the figures previously. Note that the particle moves in the negative X-direction for the first second of motion, is at rest at the moment t=1s, and then heads back in the positive X-direction for t>1s.
Find the displacement of the particle in the time interval t=0 s to t=1 s.
View SolutionA particle is moving such that s=t3−6t2+18t+9, where s is in meters and t is in meters and t is in seconds. Find the minimum velocity attained by the particle.
View SolutionA particle moves along the X-axis. Its X-coordinate varies with time according to the expression x=4t+2r2, where x is in meters and t is in seconds. The position-time graph for this motion is shown in the figures previously. Note that the particle moves in the negative X-direction for the first second of motion, is at rest at the moment t=1s, and then heads back in the positive X-direction for t>1s.
Find the instantaneous velocity of the particle at t=2.5s.
View Solution