A point moves so that the sum of the squares of its distances from two intersecting straight lines is constant. Prove that its locus is an ellipse.
a pair of straight lines
a parabola
an ellipse
a hyperbola
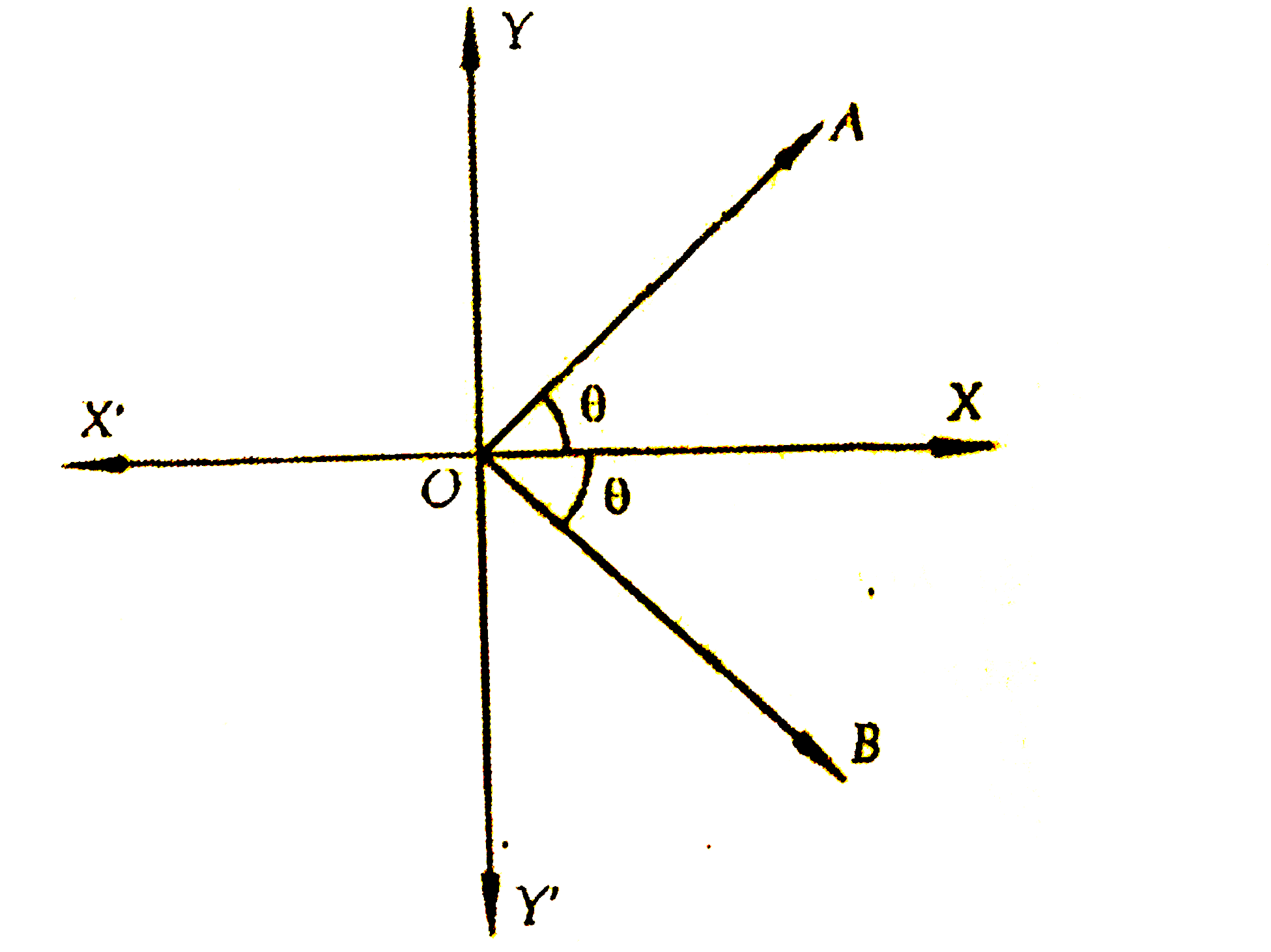
Let OA and OB be two intersecting lines containing an angle 2θ between them .
Let OX and OY be the bisectors of angle between these lines taking OX and OY as the coordinate axes , the equations of OA and OB are y= x tan θandy=−x tan θ respectively
Let P(h,k) be a variable point such that the sum squares of its distance from OA and OB is contant equal toλ2 .
i.e.,{|hsinθ−Kcosθ|√sin2θ+cos2θ}2+{|hsinθ+kcosθ|√sin2θ+cos2θ}2=λ2
⇒2h2sin2θ+2k2cos2θ=λ2
hence , the locus of (h,k) is
2x2sin2θ=λ2or,x2(λ22cosec2θ)+y2λ22sec2θ=1
Clearly , it represents an ellipse .\
Topper's Solved these Questions
- ELLIPSEBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ELLIPSEEXERCISE - Section I - Solved Mcqs
59 Videos
- ELLIPSEBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ELLIPSEEXERCISE - Section II - Assertion Reason Type
7 Videos
- DIFFERENTIATIONBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DIFFERENTIATIONEXERCISE - Chapter Test
30 Videos
- EXPONENTIAL AND LOGARITHMIC SERIESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - EXPONENTIAL AND LOGARITHMIC SERIESEXERCISE - Chapter Test
20 Videos
Similar Questions
A point moves so that the differerence of the squares of its distance from the x-axis and the y-axis is constant. Find the equation of its locus.
View SolutionProve that the locus of the point that moves such that the sum of the squares of its distances from the three vertices of a triangle is constant is a circle.
View SolutionThe locus of a point which moves so that the difference of the squares of its distance from two given points is constant, is a
View SolutionA point moves so that the sum of the squares of the perpendiculars let fall from it on the sides of an equilateral triangle is constant. Prove that its locus is a circle.
View SolutionA point moves so that the sum of the squares of the perpendiculars let fall from it on the sides of an equilateral triangle is constant. Prove that its locus is a circle.
View SolutionThe locus of a point which moves such that the sum of the square of its distance from three vertices of a triangle is constant is a/an circle (b) straight line (c) ellipse (d) none of these
View SolutionA point moves such that the sum of the squares of its distances from the sides of a square of side unity is equal to 9, the locus of such point is
View SolutionA point moves such that the sum of the squares of its distances from the two sides of length ‘a’ of a rectangle is twice the sum of the squares of its distances from the other two sides of length b. The locus of the point can be:
View SolutionProve that the locus of a point which moves such that the sum of the square of its distances from the vertices of a triangle is constant is a circle having centre at the centroid of the triangle.
View SolutionThe locus of a point which moves such that the sum of the squares of the distances from the three vertices of a triangle is constant, is a circle whose centre is at the:
View Solution
