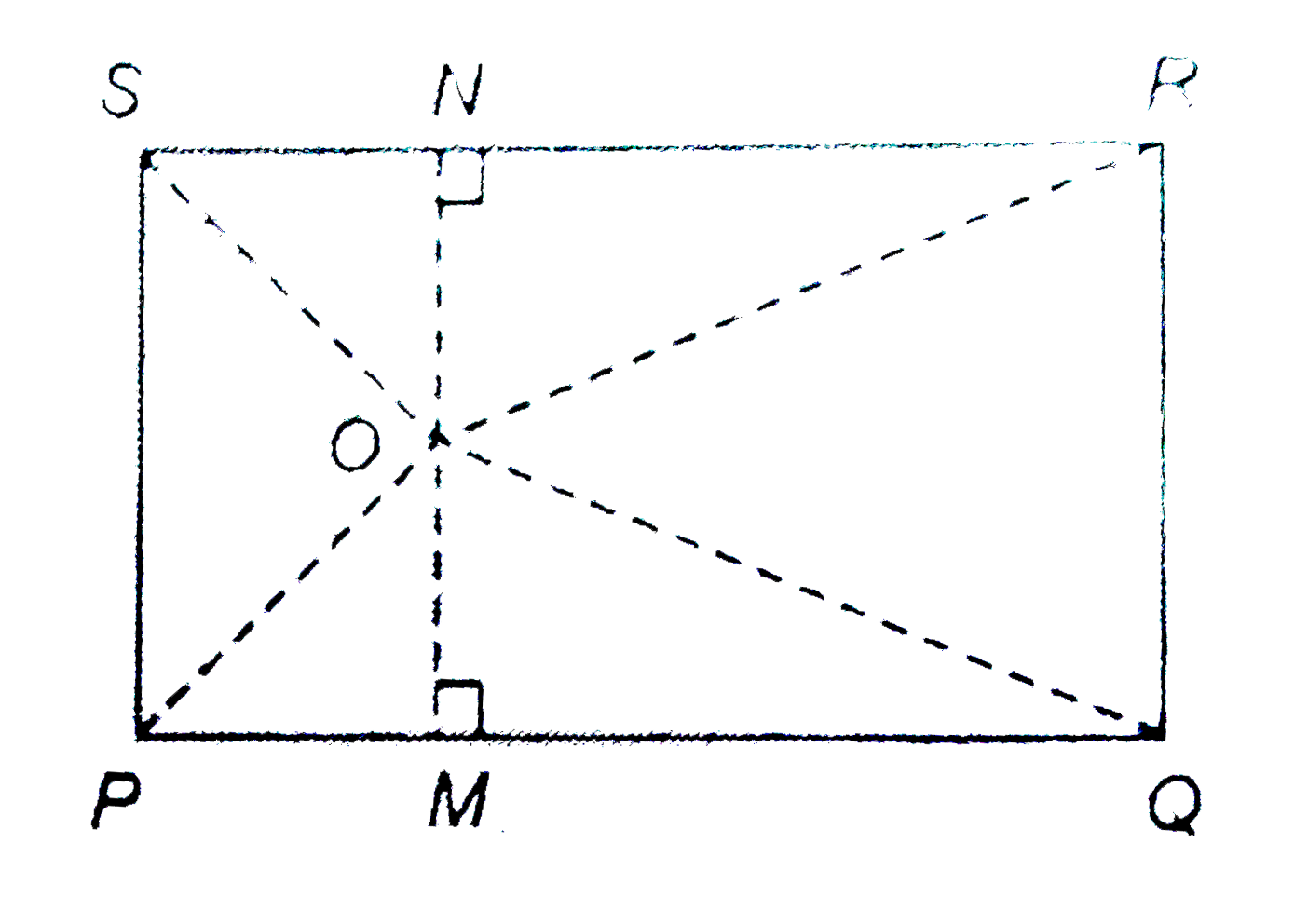
A point O inside a rectangle ABCD is joined to the vertices. Prove that the sum of the areas of a pair of opposite triangles so formed is equal to the sum of the areas of other pair of triangles.
Through O, draw MN⊥PQ
∴ area of ΔOPQ+ area of ΔORS
=12×PQ×OM+12×RS×ON
=12×PQ×OM+12×PQ×ON
(∴RS=PQ, opposite sides of a rectangle)
=12×PQ×(OM+ON)=12×PQ×MN
=12×PQ×QR=12× area of rectangle PQRS ....(1)
Again
area of ΔOPS+ area of ΔOQR= area of □PQRS−( area of ΔOPQ+ area of ΔORS)
= area of □PQRS−12× area of □PQRS [from (1)]
=12× area of □PQRS ...(2)
From (1) and (2), we get.
area of ΔOPQ+ area of ΔORS= area of ΔOPS+ area of ΔOQR
Topper's Solved these Questions
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Problems From NCERT/exemplar
12 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Exercise
34 Videos
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Revision Exercise (long Answer Questions )
5 Videos
Similar Questions
A point O inside a rectangle ABCD is joined to the vertices. Prove that the sum of the areas of a pair of opposite triangles so formed is equal to the sum of the other pair of triangles. Given: A rectangle ABCD and O is a point inside it. OA, OB, OC and OD have been joined. To Prove: ar (AOD)+ ar (BOC)= ar (AOB)+ ar(COD)
View SolutionProve that three times the sum of the squares of the sides of a triangle is equal to four times the sum of the squares of the medians of the triangle.
View SolutionIf the sides of a quadrilateral touch a circle, prove that the sum of a pair of opposite sides is equal to the sum of the other pair.
View SolutionIf one angle of a triangle is equal to the sum of the other two angles, then the triangle is
View SolutionProve that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle
View SolutionProve that the sum of opposite pair of angles of a cyclic quadrilateral is 180∘
View SolutionEquilateral triangles are drawn on the sides of a right triangle. Show that the area of the triangle on the hypotenuse is equal to the sum of the areas of triangles on the other two sides.
View SolutionEquilateral triangles are drawn on the sides of a right triangle. Show that the area of the triangle on the hypotenuse is equal to the sum of the areas of triangles on the other two sides.
View SolutionThe perimeter of an isosceles triangle is 40 cm. The base is two-third of the sum of equal sides. Find the area of the triangle .
View SolutionIf the areas of two similar triangles are equal, prove that they are congruent.
View Solution
