A point on the hypotenuse of a triangle is at distance a and b from the sides of the triangle. Show that the maximum length of the hypotenuse is (a23+b23)32 .
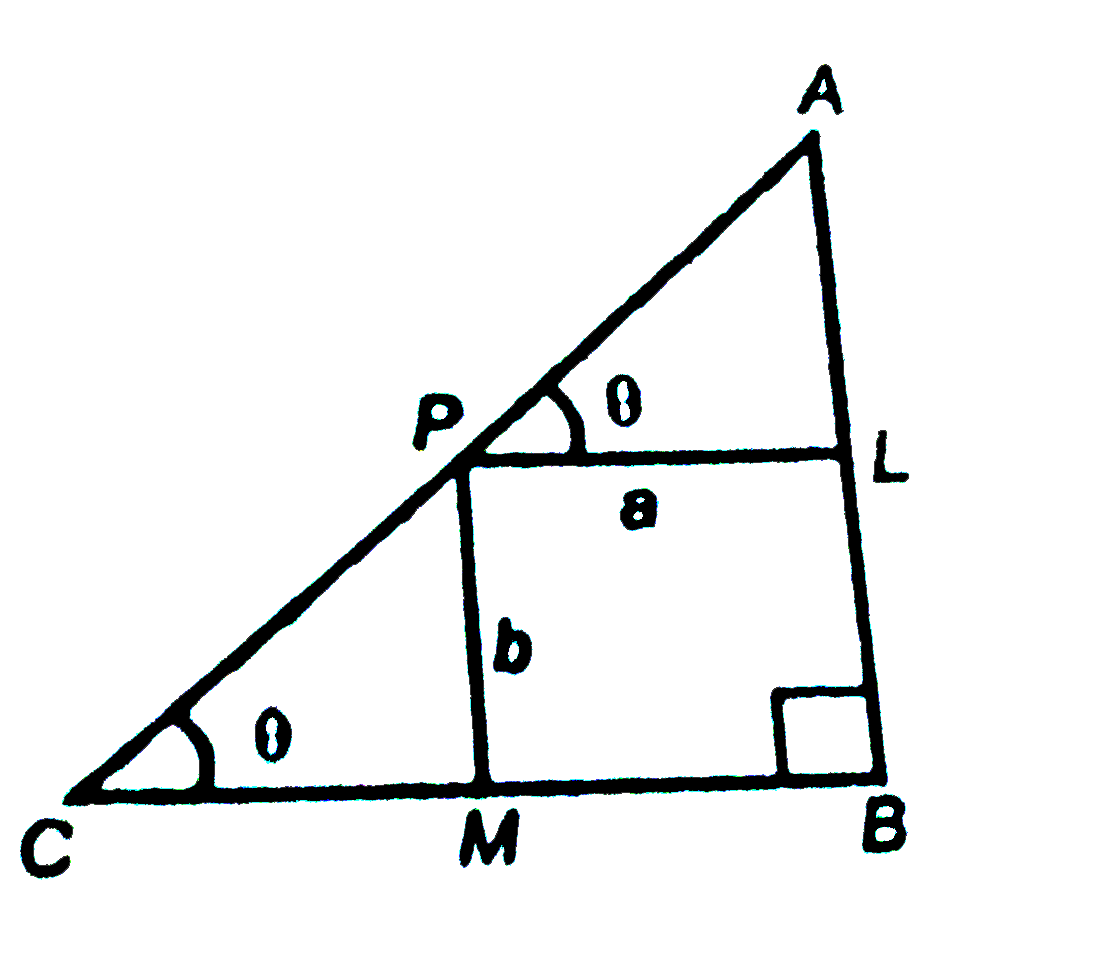
Let a point P on the hypotenuse AC of a right ΔABC such that
PL⊥AB=aandPM⊥BC=b Again, let
∠APL=∠ACB=θ then,
Ap=asecθ,PC=bcosecθ
Again if the length of hypotenuse is l, then l=AP+PC⇒l=asecθ+bcosecθ,0<θ<π2
Differentiate w.r.t.θ,
dldθ=asecθtanθ−bcosecθcotθ
For maxima/minima, dldθ=0
⇒asecθtanθ=bcosecθcotθ
⇒asinθcos2θ=bcosθsin2θ⇒sin3θcos3θ=ba
⇒rtan3θ=ba⇒tanθ=(ba)13
Now d2ldθ2=a(secθ×sec2θ+tanθ×secθtenθ)
−b[cosecθ(−cosec2θ)+cotθ(−cosecθcotθ)]
=asecθ(sec2θ+tan2θ)+bcosecθ(cosec2θ+cot2θ)
since0<θ<π2 so the trigonometric ratios will be positive and a>0,b>0
∴d2ldθ2>0
⇒ whentanθ=(ba)13

then l is minimum,
∴ minimum value of
l=asecθ+bcosecθ
a√a23+b23a13+b√a23+b23b13
=√a23+b23(a23+b23)=(a23+b23)32.
Topper's Solved these Questions
Similar Questions
A point on the hypotenuse of a right triangle is at distances a and b from the sides of the triangle. Show that the minimum length of the hypotenuse is (a23+b23)32
View SolutionThe hypotenuse of a triangle is 2.5 cm. If one of the sides is 1.5 cm. find the length of the other side.
View SolutionProve that the mid-point of the hypotenuse of a right triangle is equidistant from its vertices.
View SolutionAn isosceles right triangle has area 8 cm2. The length of its hypotenuse is
View SolutionIf A be the area of a right triangle and b one of the sides containing the right angle, prove that the length of the altitude on the hypotenuse is 2AB√b4+4A2
View SolutionThe hypotenuse of a right triangle is 25 cm. The difference between the lengths of the other two sides of the triangle is 5 cm. Find the lengths of these sides.
View SolutionProve that (2, −2), (−2, 1) and (5, 2) are the vertices of a right angled triangle. Find the area of the triangle and the length of the hypotenuse.
View SolutionThe hypotenuse of a right triangle is 13cm long. If one of the remaining two sides is of length 5cm, find the length of another side.
View SolutionThe lengths of the sides of a triangle are in the ratio 2:3:4. If the perimeter of the triangle is 63 cm, find the lengths of the sides of the triangle.
View SolutionThe hypotenuse of a right triangle is 6 m more than the twice of the shortest side. If the third side is 2 m less than the hypotenuse, find the sides of the triangle.
View Solution

