Find the area under the given curves and given lines:(i) and x-axis(ii) , and x-axis
(i) Given curve represents a parabola whose vertex is (0, 0).
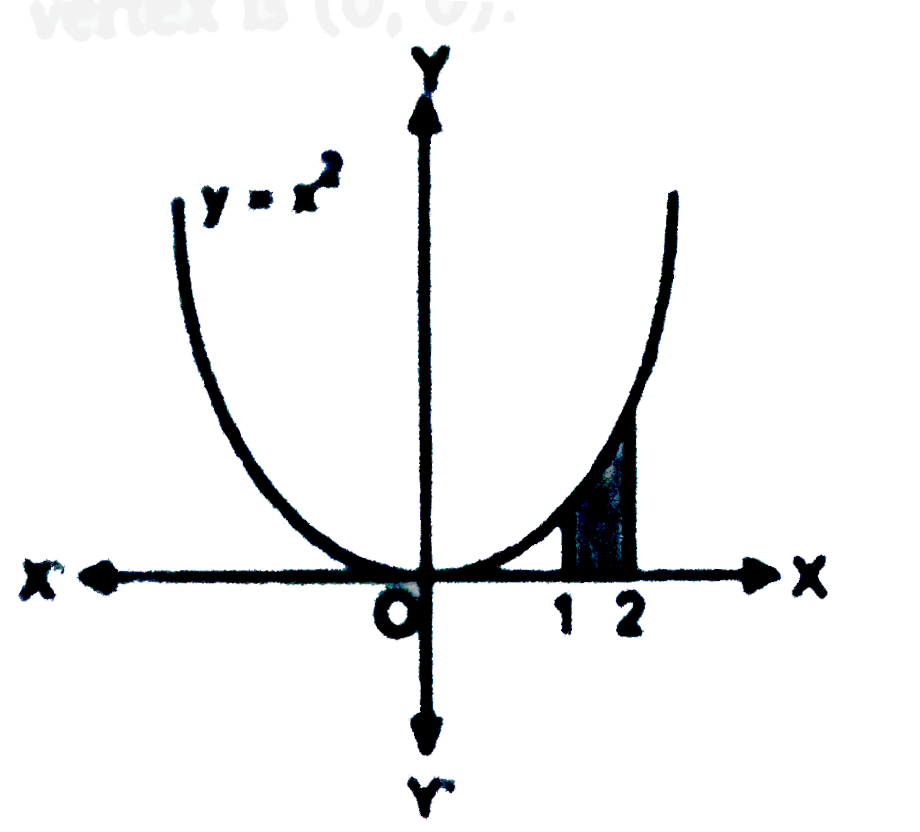
Required area (shaded region)
sq. units.
(ii) Given ,
Here the degree of x is even, so the curve is symmetric about Y-axis and passes through the origin (0, 0).
Required area= area between the curve , X-axis, and .
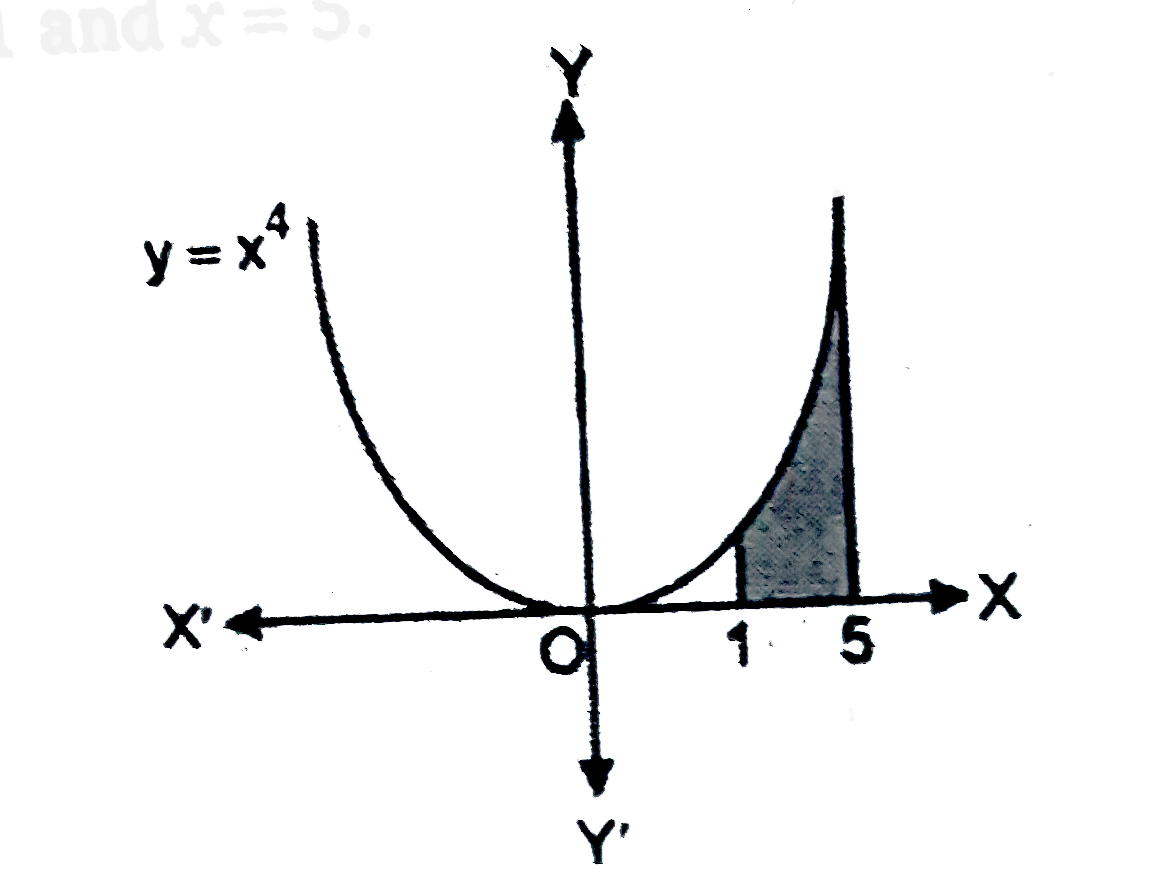
sq. units.
Topper's Solved these Questions
- APPLICATIONS OF INTEGRALSBook:NAGEEN PRAKASHAN ENGLISHChapter:APPLICATIONS OF INTEGRALSExercise:Exercise 8.2
Explore 7 Videos
- APPLICATIONS OF DERIVATIVESBook:NAGEEN PRAKASHAN ENGLISHChapter:APPLICATIONS OF DERIVATIVESExercise:Miscellaneous Exercise
Explore 24 Videos
- Continuity and DifferentiabilityBook:NAGEEN PRAKASHAN ENGLISHChapter:Continuity and DifferentiabilityExercise:Miscellaneous Exercise
Explore 23 Videos
Similar Questions
Find the area of the region bounded by the curve y2=xand the lines x=1,x=4and the x-axis.
View SolutionFind the area bounded by the curve y=2x1+x2 and the lines x = -1 ,x = 2 and the x-axis
View SolutionFind the area bounded by the curve y2=4ax and the lines y=2 and y-axis.
View SolutionFind the area bounded by the curve |x|+y=1 and axis of x.
View SolutionFind the area bounded by the curve y=x2,x=1,x=2 and x-axis .
View SolutionFind the area of the region enclosed by the given curves .
y=x3+3,y=0,x=−1,x=2
View SolutionFind the area bounded by the curve y=2cosx and the X-axis from x = 0 to x=2π.
View SolutionFind the area bounded by the curve y=x(x−1)(x−2) and the x-axis.
View SolutionFind the area bounded by the curve 2x2−y=0 and the lines x=3,y=1 and the x-axis all in first quadrant.
View SolutionFind the area bounded by the parabola y=x2, the x -axis and the lines x=−1,x=2 .
View Solution