A raft is pulled to the bank by means of a rope which is wound on a drum, at a rate of 3m/min. Determine the speed of the raft at the moment when it is 25m distant from the bank if the drum is situated on the bank 4m above water level.
To solve the problem, we will use related rates in calculus. We will define the variables, set up the relationship using the Pythagorean theorem, and then differentiate to find the speed of the raft.
1. Define the Variables:
- Let x be the horizontal distance of the raft from the bank (in meters).
- Let L be the length of the rope from the drum to the raft (in meters).
- The height of the drum above the water level is constant at 4 meters.
2. Set Up the Relationship:
According to the Pythagorean theorem, we have:
L2=x2+42
Simplifying this gives:
L2=x2+16
3. Differentiate with Respect to Time:
We will differentiate both sides with respect to time t:
ddt(L2)=ddt(x2+16)
This gives:
2LdLdt=2xdxdt
We can simplify this to:
LdLdt=xdxdt
4. Substitute Known Values:
We know that the rope is being pulled at a rate of dLdt=−3 m/min (negative because the length of the rope is decreasing). We need to find dxdt when x=25 m.
5. Calculate L when x=25:
Substitute x=25 into the Pythagorean relationship:
L2=252+42=625+16=641
Therefore, L=√641.
6. Substitute L and Solve for dxdt:
Now, substituting into the differentiated equation:
√641⋅(−3)=25⋅dxdt
Rearranging gives:
dxdt=−3√64125
7. Calculate the Speed of the Raft:
Now we can compute the numerical value:
√641≈25.31(using a calculator)
Thus,
dxdt≈−3×25.3125≈−3.0384 m/min
Therefore, the speed of the raft is approximately 3.0384 m/min towards the bank.
Final Answer:
The speed of the raft when it is 25 m from the bank is approximately 3.0384 m/min.
- Let x be the horizontal distance of the raft from the bank (in meters).
- Let L be the length of the rope from the drum to the raft (in meters).
- The height of the drum above the water level is constant at 4 meters.
According to the Pythagorean theorem, we have:
L2=x2+42
Simplifying this gives:
L2=x2+16
We will differentiate both sides with respect to time t:
ddt(L2)=ddt(x2+16)
This gives:
2LdLdt=2xdxdt
We can simplify this to:
LdLdt=xdxdt
We know that the rope is being pulled at a rate of dLdt=−3 m/min (negative because the length of the rope is decreasing). We need to find dxdt when x=25 m.
Substitute x=25 into the Pythagorean relationship:
L2=252+42=625+16=641
Therefore, L=√641.
Now, substituting into the differentiated equation:
√641⋅(−3)=25⋅dxdt
Rearranging gives:
dxdt=−3√64125
Now we can compute the numerical value:
√641≈25.31(using a calculator)
Thus,
dxdt≈−3×25.3125≈−3.0384 m/min
Therefore, the speed of the raft is approximately 3.0384 m/min towards the bank.
The speed of the raft when it is 25 m from the bank is approximately 3.0384 m/min.
Topper's Solved these Questions
- DIFFERENTIATION OF FUNCTIONS BOOK - IA MARONCHAPTER - DIFFERENTIATION OF FUNCTIONS EXERCISE - 2.6 (APPLICATION OF THE DERIVATIVE)
3 Videos
- DIFFERENTIATION OF FUNCTIONS BOOK - IA MARONCHAPTER - DIFFERENTIATION OF FUNCTIONS EXERCISE - 2.7 (ADDITIONAL PROBLEMS)
7 Videos
- DIFFERENTIATION OF FUNCTIONS BOOK - IA MARONCHAPTER - DIFFERENTIATION OF FUNCTIONS EXERCISE - 2.4 (DIFFERENTIATION OF INVERSE, IMPLICIT AND PARAMETRICALLY REPRESENTED FUNCTIONS)
2 Videos
- BASIC CLASSES OF INTEGRABLE FUNCTIONS BOOK - IA MARONCHAPTER - BASIC CLASSES OF INTEGRABLE FUNCTIONS EXERCISE - 5.8 INTEGRATION OF OTHER TRANSCENDENTAL FUNCTIONS
5 Videos
- IMPROPER INTEGRALSBOOK - IA MARONCHAPTER - IMPROPER INTEGRALSEXERCISE - 8.4 ADDITIONAL PROBLEMS
1 Videos
Similar Questions
A raft is pulled to the bank by means of a rope which is wound on a drum, at the rate of 3m/s.Determine the speed of the raft at the moment when it is 25m distance from the bank, if the drum is situated on the bank 4m above water level.
View SolutionA man who is 1.6 m tall walks away from a lamp which is 4 m above ground at the rate of 30 m/min. How fast is the man's shadow lengthening?
View Solution
Knowledge Check
A bucket is drawn from a well by means of a rope which is wound around a wheel of radius 48 cm. If the bucket ascends in 1 minute 12 seconds at a speed of 1.2 m/sec. find the length of the rope.
A8.64 cmB86.4 cmC864 cmD8640 cmA man on the tower 15 metres above the water pulls in a rope, attached to a boat, at the rate of 0.8m/sec .When the boat is 8m from the tower, it is approaching the river bank at the rate of
A17m/sec.B1.7m/sec.C0.7m/sec.D7m/sec.Which of the following statements are true ?
(i) A uniform wooden plank of mass 150kg and length 8m is floating on still water with a man of 50kg at one end of it. The man walks to the other end of the plank and stops. The distance covered by the plank is 2m.
(ii) A kid of mass 15kg is sitting in a boat of mass 45kg in a lake. The distance of kid from the bank of lake is 12m. Now the body moves inside the boat at a distance 4m towards the bank and stops. The distance of the kid from the bank (there is no friction between the water and the boat ) is 11m.
(iii) Two persons of masses 40kg and 60kg are sitting at the midpoint of a 12−m- long boat (140kg) standing still in water. Now they move to opposite ends of the boat. Neglecting the friction between boat and water, the distance traveled by the boat in water is 50cm.
(iv) In a gravity free space , a man of mass m standing at a height h above the floor, throws a ball of mass m0 straight down with a speed v0. When the ball reaches the floor, the distance of the man above the floor is (1+m0m)h.A(i),(ii)B(i) ,(iii) ,(iv)C(ii),(iii),(iv)Dall of the above
A conical vessel whose height is 10 meters and the radius of whose base is half that of the height is being filled with a liquid at a uniform rate of 1.5m3/min. Find the rate which the level of the water in the vessel is rising when it is 3m below the top of the vessel.
View SolutionA surveryor on one bank of canal observed the image of the 4inch and 17ft marks on a vertical staff,which is partially immersed in the water and held against the bank directly opposite to him, coincides If the 17ftmark and the suveryor's eye are both 6ftabove the water level ,estimate the width of the canal ,assuming that the reflective index of the water is4/3.Zero mark is at the bottom of the canal.
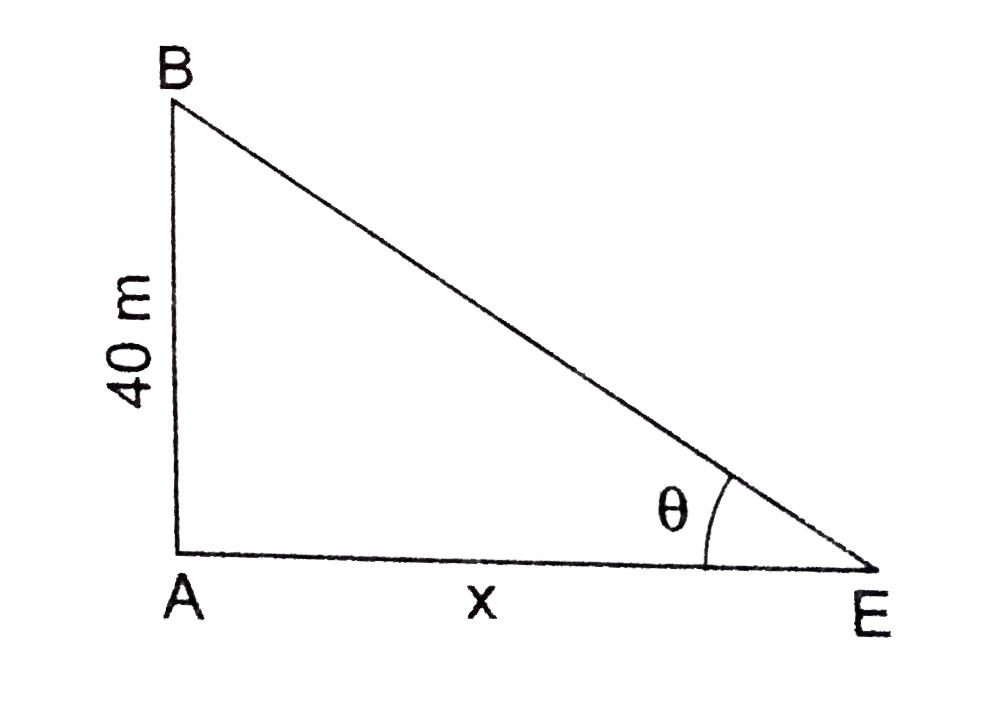
View SolutionA man is moving away from a 40-m high tower at a speed of 2 m/s. Find the rate at which the angle of elevation of the top of the tower is changing when he is at a distance of 30 metres from the foot of the tower. Assume that the eye level of the man is 1.6 m from the ground.
View SolutionA short electric dipole has dipole moment of 4×10−9Cm. Determine the electric potential due to the dipole at a point distant 0.3 m from the centre of the dipole situated on (a) the axial line (b) on equatorial line and (c) on a line making an angle of 60∘ wth the dipole axis.
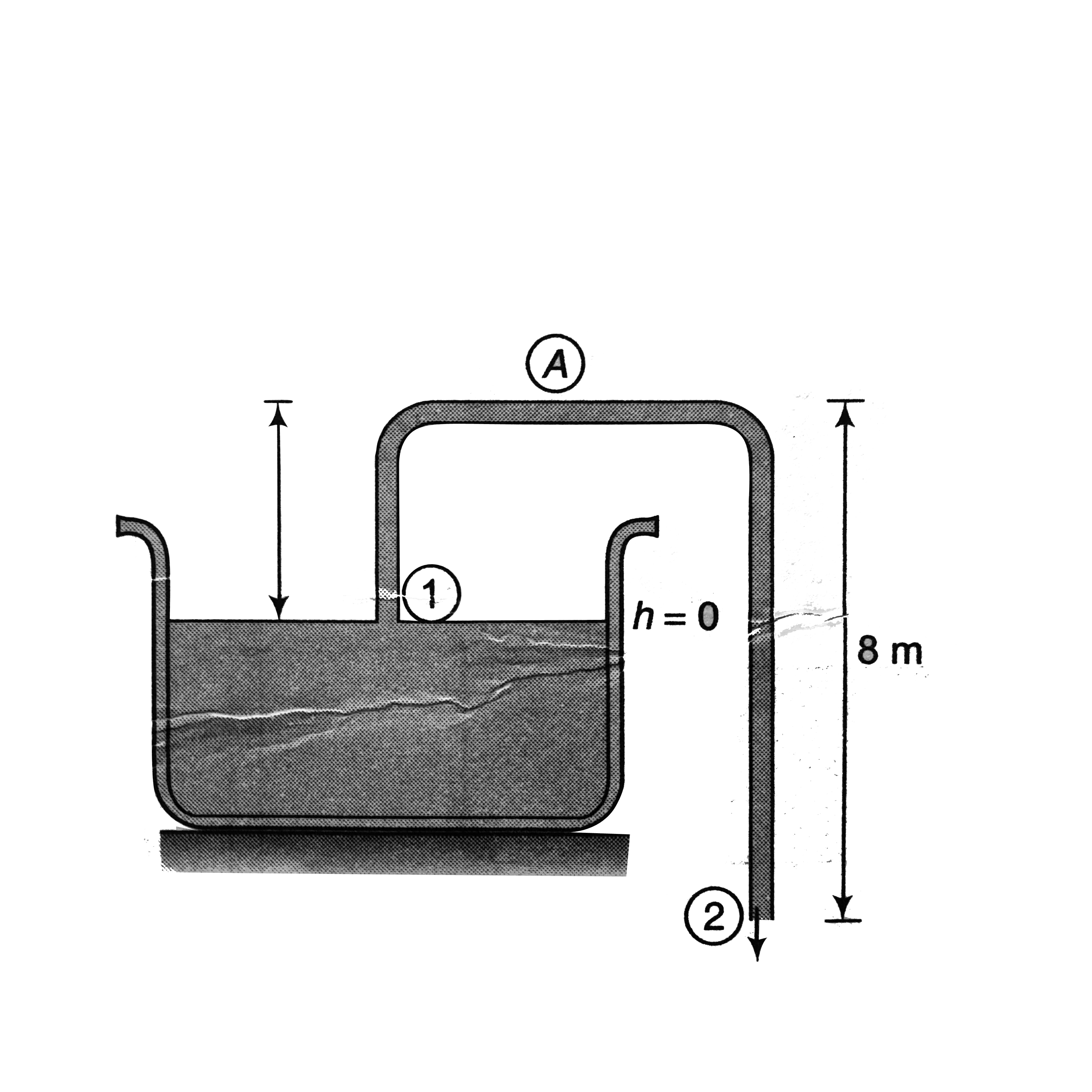
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
 .
.
View Solution
