A right triangle is drawn in a semicircle of radius 12 with one of its legs along the diameter. If the maximum area of the triangle is M , then the value of 32√3Mc is______Solution in Bengali
The correct Answer is:9
BC×Ce=AC×CD
therfore(BC)(CE)=x(1−x)
But BC=CE
thereBC=√x(1−x)
∴Area△=x√x−x22
or △=x3−x44
or d△2dx=3x2−4x34
If d△2dx=0 then x=34 which is the point of maxima
Hence maximum area is 3√332

Topper's Solved these Questions
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Single Correct Answer Type
13 Videos
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Linked comprehension Type
2 Videos
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Matrix Match Type
11 Videos
- METHODS OF DIFFERENTIATIONBOOK - CENGAGE PUBLICATIONCHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - Multiple Correct Answer Type
7 Videos
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
Similar Questions
Given P(x)=x4+ax3+bx2+cx+d such that x=0 is the only real root of P'(x)=0. If P(−1)<P(1), then in the interval [−1,1]
View SolutionLet f:[0,1]→R be a function. Suppose the function f is twice differentiable, f(0)=f(1)=0 and satisfies f''(x)−2f'(x)+f(x)≥ex,x∈[0,1] Which of the following is true for 0<x<1?
View Solutionconsider function f(x) =x4−14x2+24x−3. Now match the following lists:

View SolutionIf y=cos−1√√1+x2+12√1+x2,thendydxisequa<o 12(1+x2),x∈R (b) 12(1+x2),x>0 −12(1+x2),x<0 (d) 12(1+x2),x<0
View SolutionLet f:R→R,y=f(x),f(0)=0,f'(x)>0andf''(x)>0. Three point A(α,f(α)),B(β,f(β)),C(γ,f(γ))ony=f(x) such that 0<α<β<γ.
Which of the following is false ?
View SolutionProve that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle
View SolutionA right triangle is drawn in a semicircle of radius 12 with one of its legs along the diameter. If the maximum area of the triangle is M , then the value of 32√3Mc is______
View SolutionIn a Delta ABC, a semicircle is inscribed,whose diameter lies on the side c.Then the radius of the semicircle is (Where Delta is the area of the triangle ABC)
View Solution6 मी त्रिज्या वाले अर्धवृत्त में बनाए जा सकने वाले सबसे बड़े त्रिभुज का क्षेत्रफल कितना होगा?
View SolutionThe legs of a right triangle are in the ratio 3 : 4 and its area is 1014cm2 . Find the lengths of its legs.
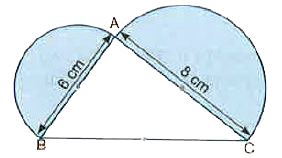
View SolutionIn Fig 12.58, ABC is a triangle right angled at A. Semicircles are drawn on AB and AC as diameters. Find the area of the shaded region.
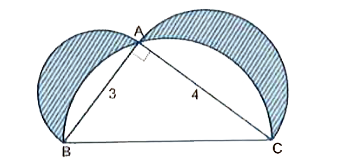
View SolutionABC is a right-angled triangle at A. Semicircles drawn on AB, AC and BC as diameters. Find area of the shaded region.
View SolutionProve that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle
View SolutionA right triangle is drawn in a semicircle of radius 12 with one of its legs along the diameter. If the maximum area of the triangle is M , then the value of 32√3Mc is______
View SolutionThe area of a right triangle is 50 m2 . If one of the legs is 20 m, find the length of the other leg.
View Solutionसिद्ध कीजिए कि एक समकोण त्रिभुज के कर्ण पर खींचे गए अर्धवृत्त का क्षेत्रफल अन्य दो भुजाओं पर खींचे गए अर्धवृत्तों के क्षेत्रफलों के योग के बराबर होता है।
View Solution
