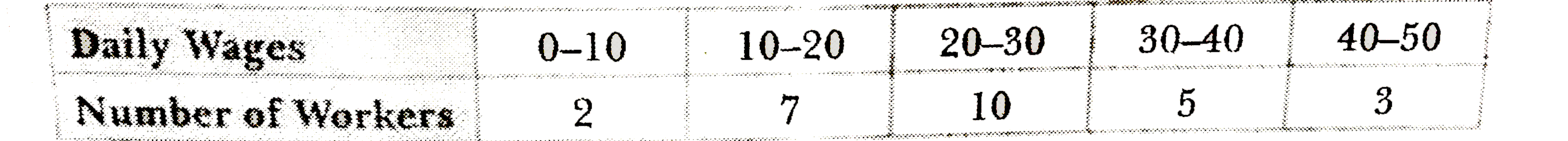A simple of 25 variates has mean 40 and standard deviation 5 and a second sample of 35 variates has mean 45 and the standard deviation 2. Find the mean and standard deviation of the two samples of variates , taken together.
The correct Answer is:C
To find the combined mean and standard deviation of two samples, we can follow these steps:
Step 1: Calculate the Combined Mean
Given:
- Sample A:
- Number of variates (n1) = 25
- Mean (x̄1) = 40
- Sample B:
- Number of variates (n2) = 35
- Mean (x̄2) = 45
The formula for the combined mean (x̄) is:
¯x=(xˉ1⋅n1)+(xˉ2⋅n2)n1+n2
Substituting the values:
¯x=(40⋅25)+(45⋅35)25+35
Calculating the numerator:
=1000+157560=257560≈42.92
Step 2: Calculate the Combined Standard Deviation
Given:
- Standard deviation of Sample A (σ1) = 5
- Standard deviation of Sample B (σ2) = 2
The formula for the combined standard deviation (σ) is:
σ=√(σ21⋅n1)+(σ22⋅n2)+(d21⋅n1)+(d22⋅n2)n1+n2
Where:
- d1=|¯x−xˉ1|
- d2=|¯x−xˉ2|
Calculating d1 and d2:
d1=|42.92−40|=2.92
d2=|42.92−45|=2.08
Now substituting the values into the standard deviation formula:
σ=√(52⋅25)+(22⋅35)+(2.922⋅25)+(2.082⋅35)60
Calculating each term:
- 52⋅25=25⋅25=625
- 22⋅35=4⋅35=140
- 2.922⋅25=8.5284⋅25=213.21
- 2.082⋅35=4.3264⋅35=151.65
Now summing these values:
=625+140+213.21+151.65=1130.86
Now substituting back into the standard deviation formula:
σ=√1130.8660≈√18.84767≈4.34
Final Results:
- Combined Mean ≈ 42.92
- Combined Standard Deviation ≈ 4.34
Given:
- Sample A:
- Number of variates (n1) = 25
- Mean (x̄1) = 40
- Sample B:
- Number of variates (n2) = 35
- Mean (x̄2) = 45
¯x=(xˉ1⋅n1)+(xˉ2⋅n2)n1+n2
¯x=(40⋅25)+(45⋅35)25+35
Calculating the numerator:
=1000+157560=257560≈42.92
Given:
- Standard deviation of Sample A (σ1) = 5
- Standard deviation of Sample B (σ2) = 2
σ=√(σ21⋅n1)+(σ22⋅n2)+(d21⋅n1)+(d22⋅n2)n1+n2
Where:
- d1=|¯x−xˉ1|
- d2=|¯x−xˉ2|
d1=|42.92−40|=2.92
d2=|42.92−45|=2.08
σ=√(52⋅25)+(22⋅35)+(2.922⋅25)+(2.082⋅35)60
Calculating each term:
- 52⋅25=25⋅25=625
- 22⋅35=4⋅35=140
- 2.922⋅25=8.5284⋅25=213.21
- 2.082⋅35=4.3264⋅35=151.65
=625+140+213.21+151.65=1130.86
σ=√1130.8660≈√18.84767≈4.34
- Combined Mean ≈ 42.92
- Combined Standard Deviation ≈ 4.34
Topper's Solved these Questions
- STATISTICSBOOK - MODERN PUBLICATIONCHAPTER - STATISTICSEXERCISE - Objective A(Multiple Choice Questions)
14 Videos
- STATISTICSBOOK - MODERN PUBLICATIONCHAPTER - STATISTICSEXERCISE - Objective B(Fill in the Blanks)
6 Videos
- STATISTICSBOOK - MODERN PUBLICATIONCHAPTER - STATISTICSEXERCISE - Exercise 15( c) (Short Answer Type Questions)
1 Videos
- SETS BOOK - MODERN PUBLICATIONCHAPTER - SETS EXERCISE - CHAPTER TEST 1
12 Videos
- STRAIGHT LINES BOOK - MODERN PUBLICATIONCHAPTER - STRAIGHT LINES EXERCISE - Chapter test
12 Videos
Similar Questions
Knowledge Check
A sample of 90 values has mean 55 and standard deviation 3. A second sample of 110 values has mean 60 and standard deviations 2. The combined variance is equal to (upto two decimal)
A12.43B11.24C10.43D13.25A sample of 35 observations has the means 80 and SD. As 4. A second sample of 65 observations from the same population has mean 70 and S.D.3. The S.D. of the combined sample is -
A5.85B5.58C34.2DNone of theseA sample of 90 values has standard deviation 3 and their mean is 55. A second sample of 110 values has mean 60 and its standard deviation is 2. The combined variance is equal to
A12.44B13.24C16.42D13.65
Determine the mean and standard deviation for the following distribution

View SolutionFind out standard deviation and coefficient of variation .

View SolutionCalculate the mean and standard deviation of first n natural numbers.
View SolutionIf mean deviation is 12 the value of standard deviation will be-
View SolutionThe mean life of a sample of 60 bulbs was 650 and the standard deviation was 8 h. A second sample of 80 bulbs has a mean life of 660 h and standard deviation 7h. Find the over all standard deviation.
View Solution