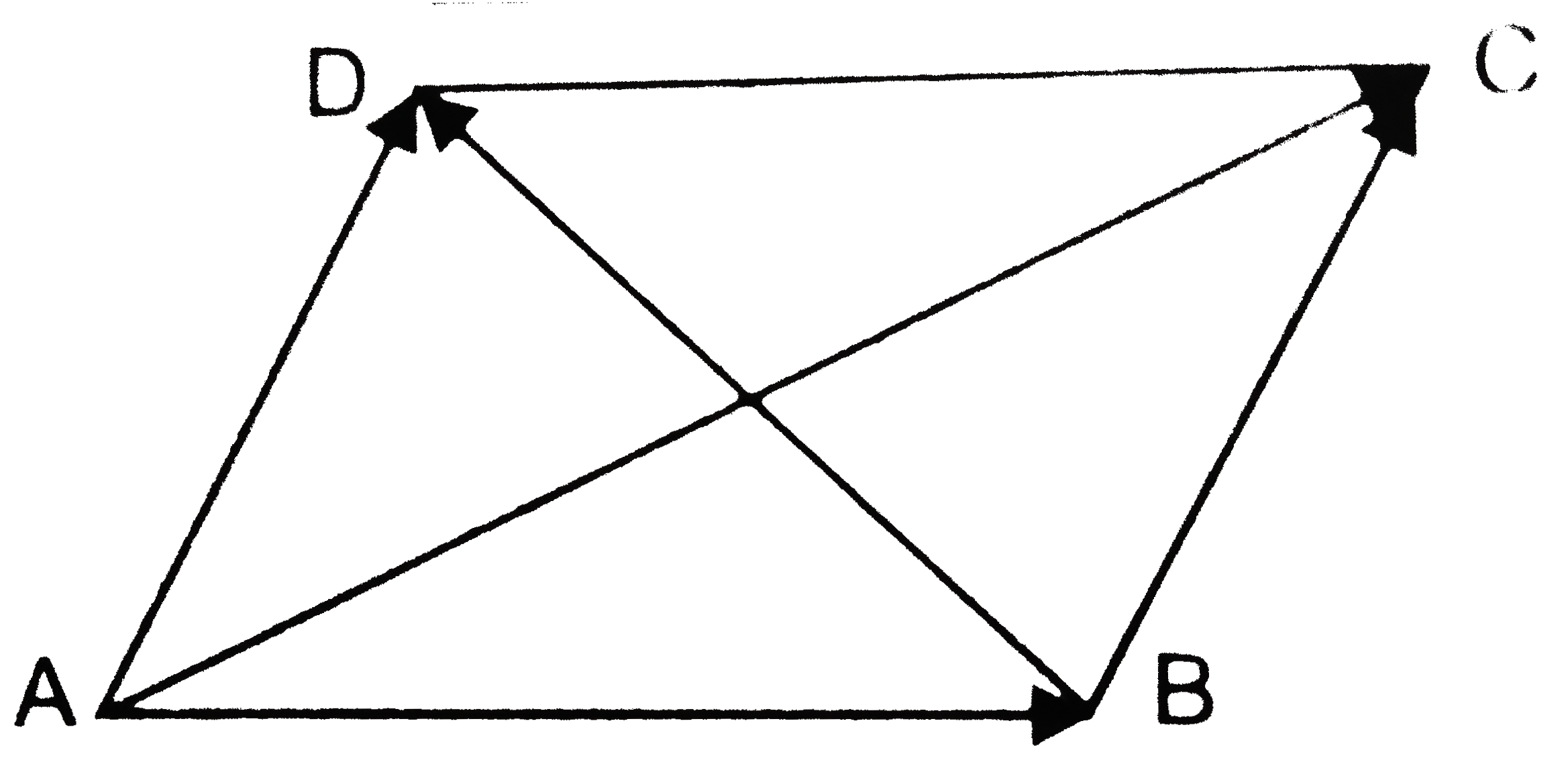
A straight line L cuts the sides AB, AC, AD of a parallelogram ABCD at

ABCD a parallelogram, and $A_{1} and B_{1}$ are the midpoints of sides BC and CD, respectively. If ${- \to a A}_{1}$ $+ {- - \to A B}_{1} = λ - - \to A C$ , then $λ$ is equal to
A $\frac{1}{2}$
B $1$
C $\frac{3}{2}$
D $2$
E and F are the interior points on the sides BC and CD of a parallelogram ABCD. Let $- - \to B E = 4 - - \to E C and - - \to C F = 4 - - \to F D$ . If the line EF meets the diagonal AC in G, then $- - \to A G = λ - - \to A C$ , where $λ$ is equal to :
A $\frac{1}{3}$
B $\frac{21}{25}$
C $\frac{7}{13}$
D $\frac{21}{5}$
E and F are the interior points on the sides BC and CD of a parallelogram ABCD. Let $- - \to B E = 4 - - \to E C and - - \to C F = 4 - - \to F D$ . If the line EF meets the diagonal AC in G, then $- - \to A G = λ - - \to A C$ , where $λ$ is equal to :
A $\frac{1}{3}$
B $\frac{21}{25}$
C $\frac{7}{13}$
D $\frac{21}{5}$

A straight line L cuts the sides AB, AC, AD of a parallelogram ABCD at $B_{1}, C_{1}, d_{1}$ respectively. If $- - \to A B_{1} = λ_{1} - - \to A B, - -- \to A D_{1} = λ_{2} - - \to A D and - - \to A C_{1} = λ_{3} - - \to A C,$ then $\frac{1}{λ_{3}}$ equal to