A study was conducted to find out the concentration of sulphur dioxide in the air in parts per million (ppm) of a certain city. The data obtained for 30 days is as follows: 0.03 0.08 0.08 0.09 0.04 0.170.16 0.05 0.02 0.06 0.18 0.200.11 0.08 0.12 0.13 0.22 0.070.08 0.01 0.10 0.06 0.09 0.180.11 0.07 0.05 0.07 0.01 0.04 (i) Make a grouped frequency distribution table for this data with class intervals as 0.00 - 0.04, 0.04 - 0.08, and so on.(ii) For how many days, was the concentration of sulphur dioxide more than 0.11 parts per million?
The correct Answer is:D
To solve the problem, we will follow these steps:
Step 1: Organize the Data
We have the following data for the concentration of sulphur dioxide in parts per million (ppm) over 30 days:
0.03, 0.08, 0.08, 0.09, 0.04, 0.17, 0.16, 0.05, 0.02, 0.06, 0.18, 0.20, 0.11, 0.08, 0.12, 0.13, 0.22, 0.07, 0.08, 0.01, 0.10, 0.06, 0.09, 0.18, 0.11, 0.07, 0.05, 0.07, 0.01, 0.04
Step 2: Define Class Intervals
We will create class intervals as follows:
- 0.00 - 0.04
- 0.04 - 0.08
- 0.08 - 0.12
- 0.12 - 0.16
- 0.16 - 0.20
- 0.20 - 0.24
Step 3: Count the Frequency for Each Interval
Now we will count how many days fall into each class interval.
- 0.00 - 0.04: 0.03, 0.02, 0.01, 0.01, 0.04 (Total: 4 days)
- 0.04 - 0.08: 0.04, 0.05, 0.06, 0.07, 0.07, 0.06, 0.08, 0.08, 0.08 (Total: 9 days)
- 0.08 - 0.12: 0.09, 0.09, 0.10, 0.11, 0.08, 0.12 (Total: 6 days)
- 0.12 - 0.16: 0.13, 0.12, 0.16 (Total: 3 days)
- 0.16 - 0.20: 0.17, 0.18, 0.18, 0.20 (Total: 4 days)
- 0.20 - 0.24: 0.22 (Total: 1 day)
Step 4: Create the Frequency Distribution Table
Now we can summarize this information in a frequency distribution table:
| Class Interval | Frequency (Number of Days) |
|----------------|---------------------------|
| 0.00 - 0.04 | 4 |
| 0.04 - 0.08 | 9 |
| 0.08 - 0.12 | 6 |
| 0.12 - 0.16 | 3 |
| 0.16 - 0.20 | 4 |
| 0.20 - 0.24 | 1 |
| Total | 30 |
Step 5: Answer the Second Question
Now we need to find out for how many days the concentration of sulphur dioxide was more than 0.11 ppm. This means we will count the days in the intervals:
- 0.12 - 0.16 (3 days)
- 0.16 - 0.20 (4 days)
- 0.20 - 0.24 (1 day)
Adding these gives us:
3 + 4 + 1 = 8 days
Final Answers:
(i) The grouped frequency distribution table is as shown above.
(ii) The concentration of sulphur dioxide was more than 0.11 ppm for 8 days.
We have the following data for the concentration of sulphur dioxide in parts per million (ppm) over 30 days:
0.03, 0.08, 0.08, 0.09, 0.04, 0.17, 0.16, 0.05, 0.02, 0.06, 0.18, 0.20, 0.11, 0.08, 0.12, 0.13, 0.22, 0.07, 0.08, 0.01, 0.10, 0.06, 0.09, 0.18, 0.11, 0.07, 0.05, 0.07, 0.01, 0.04
We will create class intervals as follows:
- 0.00 - 0.04
- 0.04 - 0.08
- 0.08 - 0.12
- 0.12 - 0.16
- 0.16 - 0.20
- 0.20 - 0.24
Now we will count how many days fall into each class interval.
- 0.04 - 0.08: 0.04, 0.05, 0.06, 0.07, 0.07, 0.06, 0.08, 0.08, 0.08 (Total: 9 days)
- 0.08 - 0.12: 0.09, 0.09, 0.10, 0.11, 0.08, 0.12 (Total: 6 days)
- 0.12 - 0.16: 0.13, 0.12, 0.16 (Total: 3 days)
- 0.16 - 0.20: 0.17, 0.18, 0.18, 0.20 (Total: 4 days)
- 0.20 - 0.24: 0.22 (Total: 1 day)
Now we can summarize this information in a frequency distribution table:
|----------------|---------------------------|
| 0.00 - 0.04 | 4 |
| 0.04 - 0.08 | 9 |
| 0.08 - 0.12 | 6 |
| 0.12 - 0.16 | 3 |
| 0.16 - 0.20 | 4 |
| 0.20 - 0.24 | 1 |
| Total | 30 |
Now we need to find out for how many days the concentration of sulphur dioxide was more than 0.11 ppm. This means we will count the days in the intervals:
- 0.12 - 0.16 (3 days)
- 0.16 - 0.20 (4 days)
- 0.20 - 0.24 (1 day)
3 + 4 + 1 = 8 days
(i) The grouped frequency distribution table is as shown above.
(ii) The concentration of sulphur dioxide was more than 0.11 ppm for 8 days.
Similar Questions
A study was conduced to find out the concentration of sulphur dioxide in the air in parts per million (ppm) of a certain city. The data obtained for 30 days is as follow:
0.03 0.08 0.08 0.09 0.04 0.170.16 0.05 0.02 0.06 0.18 0.200.11 0.08 0.12 0.13 0.22 0.070.08 0.01 0.10 0.06 0.09 0.180.11 0.07 0.05 0.07 0.01 0.04
You were asked to prepare a frequency distribution table, regarding the concentration of sulphur dioxide in the air in parts per million of a certain city for 30 days. Using this table, find the probability of the concentration of sulphur dioxide in the interval 0.12-0.16 on any of these days.View SolutionA study was conducted to find out the concentration of sulphur dioxide in the air in parts per million (ppm) of a certain city. The data obtained for 30 days is a follows :
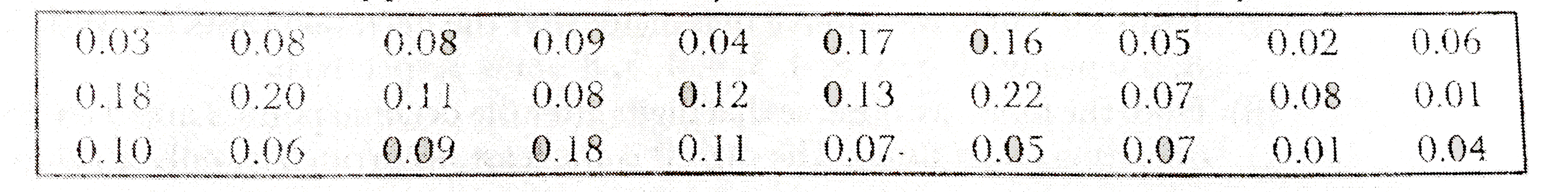
(i) Make a grouped frequency distribution table for this data with class-intervals as 0.00−0.04,0.04−0.08 and so on.
(ii) For how many day's was the concentration of sulphar dioxide more than 0.11 parts per million (ppm)?View Solution
Knowledge Check
- Question 1 - Select One
In a first order reaction, the concentration of the reactant decreases from 0.8 M to 0.4 M in 15 minutes. The time taken for the concentration of to change from 0.1 M to 0.025 M is
A30 minB15 minC7.5 minD60 min
0.02×0.05=
(a) 0.1
(b) 0.01
(c) 0.001
(d) 0.0001View Solution0.012×0.15=
(a) 0.8
(b) 0.08
(c) 0.008
(d) 0.0018View SolutionTo find out the concentrations of SO2 in the air (in parts per million, i.e., ppm), the data was collected for 30 localities in a certain city and is presented below: Concentration of SO2 (in ppm) Frequency 0.00 - 0.04 4 0.04 - 0.08 9 0.08 - 0.12 9 0.12 - 0.16 2 0.16 - 0.20 4 0.20 - 0.24 2 Find the mean concentration of SO2 in the air.
View SolutionGiven below is the frequency distribution table regarding the concentration of sulphur dioxide in the air in parts per million of a certain city for 30 days.
Concentration0.00−0.040.04−0.080.08−0.120.12−0.160.16−0.200.20−0.24No. of days:489243
Find the probability of concentration of sulphur dioxide in the interval 0.12-0.16 on any of these days.View SolutionEvaluate: 0.8×0.8×0.8+0.5×0.5×0.50.8×0.8−0.8×0.5+0.5×0.5
View Solution8 ml is equal to (a) 0.8 L (b) 0.08 L (c) 0.008 L (d) None of these
View SolutionWhich of the following are like decimals: 0.34, 0.07, 5.35, 24.70 45.05, 4.505, 20.55, 20.5 8.80, 17.08, 8.94, 0.27 4.50, 16.80, 0.700, 7.08
View Solution

