A tyre company is able to manufacture (hundred) grade tyres and y (hundred) grade tyres per day, where If the profit oneach grade A tyre is twice the profit on grade B tyre,what is the most profitable number of grade A tyresper day to manufacture?
Step by step video & image solution for A tyre company is able to manufacture x (hundred) grade A tyres and y (hundred) grade B tyres per day, where y(5-x) = 10(4 - X), 0 le x le 4. If the profit oneach grade A tyre is twice the profit on grade B tyre,what is the most profitable number of grade A tyresper day to manufacture? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A tyre company is able to manufacture x (hundred) grade A tyres and y (hundred) grade B tyres per day, where y(5−x)=10(4−X),0≤x≤4. If the profit oneach grade A tyre is twice the profit on grade B tyre,what is the most profitable number of grade A tyresper day to manufacture?
View SolutionA manufacture produces two types of soap bars using two machines, A and B.A is operated for 2 minutes and B for 3 minutes to manufacture the first type, while it takes 3 minutes on machine A and 5 minutes on machine B to manufacture the second type. Each machine can be operated at the most for 8 hours per day. The two types of soap bars are sold at a profit of R. 0.25 and Rs 0.50 each. Assuming that the manufacture can sell all the soap bars he can manufacture, hwo many bars of soap of each type should be manufactured per day so as to maximize his profit ?
View Solution
Knowledge Check
A manufacturer produces two types of soaps using two machines A and B . A is operated for 2 minutes and B for 3 minutes to manufacture first type , while it takes 3 minutes on machine A and 5 minutes on machine B to manufature second type . Each machine can be operated at the most for 8 hours per day . The two types of soap are sold at a profit of Rs 0.25 and Rs 0.05 each respectively . Assuming that the manufactured can sell all the soaps he can manufacture , how many soaps of each type should be manufature per day so as to maximize his profit .
A50 soaps of type I , 20 soaps of types IIB96 soaps of type IIC45 soaps of type ID55 soaps of type IMaximum profit: An barrels manufacturer can produce up to 300 barrels per day. The profit made from the sale of these barrels can be modelled by the function P(x)=−10x2+3500x−66000 where P(x) is the profit in rupees and x is the number of barrels made and sold.

Based on this model answer the following questions:
What is the maximum profit which can manufacturer earn?ARs 240250BRs 480500CRs 680250DRs 240250Maximum profit: A barrels manufacturer can produce up to 300 barrels per day. The profit made from the sale of these barrels can be modelled by the function P(x)=−10x2+3500x−66000 where P(x) is the profit in rupees and x is the number of barrels made and sold.

Based on this model answer the following questions:
When no barrels are produce what is a profit loss?A22000B66000C11000D33000
A company manufactures two types of ladies dresses ,C & D. The raw material & labour available per day is given in the table.
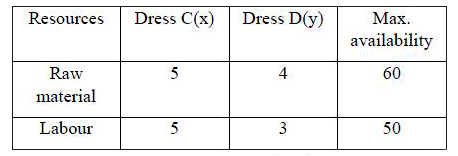
P is the profit, if P = 50x + 100y, solve this LPP to find x & y to get the maximum profit.
View SolutionThe profit function P(x) of a firm, selling x items per day is given by P(x)= (150−x)x−1625. Find the number of items the firm should manufacture to get maximum profit. Find the maximum profit
View SolutionMaximum profit: An barrels manufacturer can produce up to 300 barrels per day. The profit made from the sale of these barrels can be modelled by the function P(x)=−10x2+3500x−66000 where P(x) is the profit in rupees and x is the number of barrels made and sold.

Based on this model answer the following questions:
What is the profit/loss if 175 barrels are produced
View SolutionMaximum profit: An barrels manufacturer can produce up to 300 barrels per day. The profit made from the sale of these barrels can be modelled by the function P(x)=−10x2+3500x−66000 where P(x) is the profit in rupees and x is the number of barrels made and sold.

Based on this model answer the following questions:
What is the profit/loss if 400 barrels are produced
View SolutionMaximum profit: An barrels manufacturer can produce up to 300 barrels per day. The profit made from the sale of these barrels can be modelled by the function P(x)=−10x2+3500x−66000 where P(x) is the profit in rupees and x is the number of barrels made and sold.

Based on this model answer the following questions:
What is the break even point ? (Zero profit point is called break even)
View Solution
