A variable line L is drawn through O(0,0) to meet the line L1 and L2 given by y-x-10 =0 and y-x-20=0 at Points A and B, respectively.
Locus of P, if OP2=OA×OB, is
a. (y+x)2=50
b. (y−x)2=200
c. (y−x)2=100
d. none of theseSolution in Bengali
(y−x)2=100
(y+x)2=50
(y−x)2=200
none of these
The correct Answer is:C
r2=10×20(sinθ−cosθ)2
or (rsinθ−r cosθ)2=200
Hence, the locus is (y−x)2=200.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)8videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (NUMERICAL VALUE TYPE)13videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MULTIPLE CORRECT ANSWERS TYPE)30videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
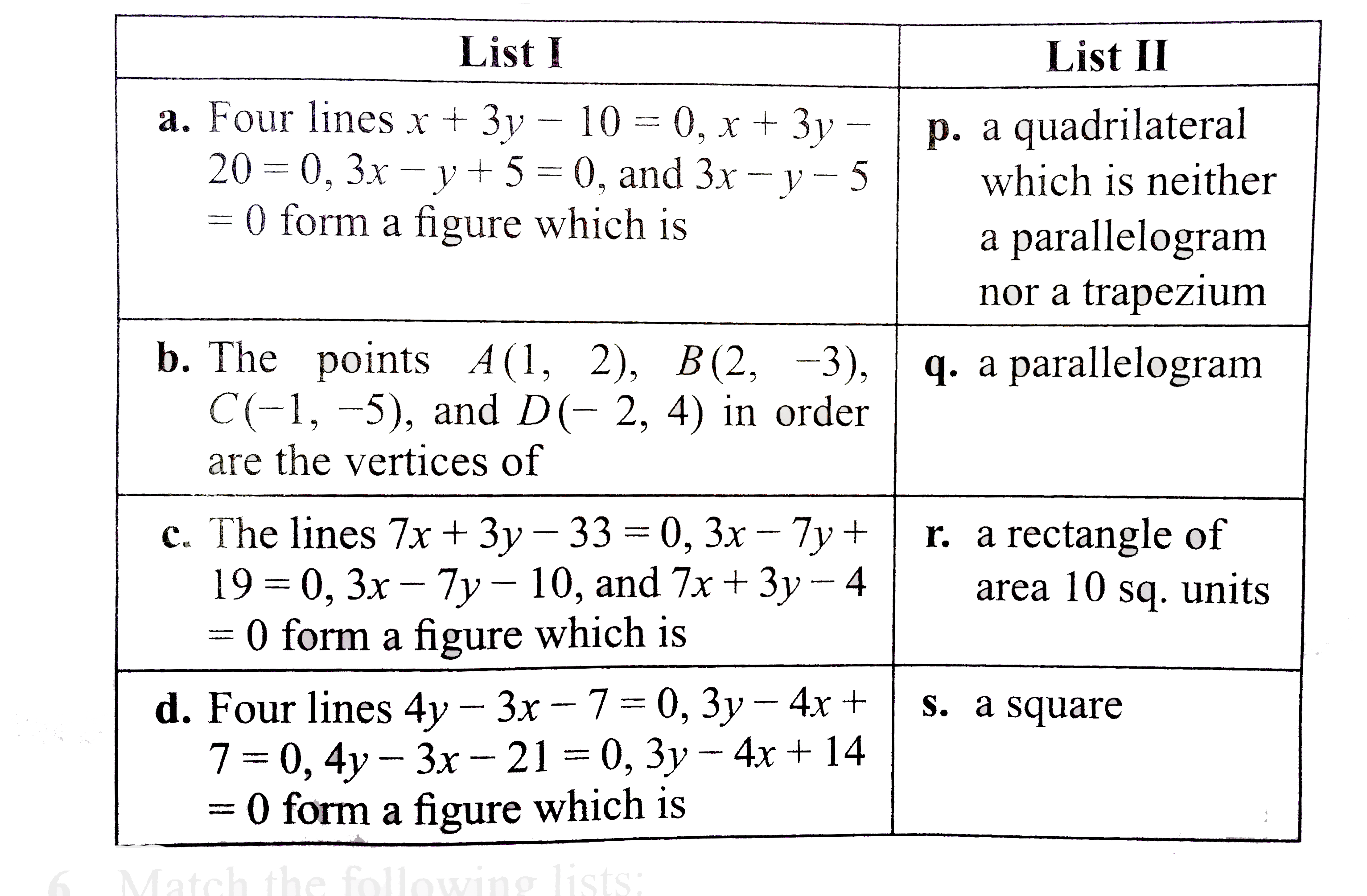
Match the following lists:
 09:22View Solution
09:22View SolutionFind the number of permutations of the set {1,2,3,4} in which no two adjacent positions are filled by consecutive integers ( increasing orders).
View SolutionIf P is a point (x,y) on the line y=−3x such that P and the point (3, 4) are on the opposite sides of the line 3x−4y=8, then x>815 (b) x>85 y<−85 (d) y<−815
04:02View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
05:34View Solutiona variable line L is drawn trough O(0,0) to meet the lines L_(1):y-x-10=0 and L_(2):y-x-20=0 at point A&B respectively.A point P is taken on line L the (1) if (2)/(OP)=(1)/(OA)+(1)/(OB) then locus of P is is (3) if (1)/((OP)^(2))=(1)/((OA)^(2))+(1)/((OB)^(2)) then locus of point P is:
03:13View SolutionA variable line L is drawn through O(0,0) to meet the line L1 and L2 given by y-x-10 =0 and y-x-20=0 at Points A and B, respectively.
Locus of P, if OP2=OA×OB, is05:55View SolutionLet C:x2−3,D:y=kx2 be two parabolas and
L1 : xa,L2:x=1(a≠0) be two straight lines.
IF the line L1 meets the parabola C at a point B on the line L2, other than A, then a may be equal to06:29View SolutionA variable line 'L' Passing through the origin cuts two parallel lines x-y+10=0 and x-y+20=0 at two points A and B respectively . If P is a point on line 'L' such that OA , OP, OB are in harmonic progression then the locus of P is
06:01View SolutionConsider the lines
L1:x+y=10, L2:x+y=60
L3:x=40, L4:y=40.
L1 meets x - axis and y - axis at A and B respectively.
L4 meets y - axis at C and L2 at D
L3 meets L2 at E and x-axis at F.
Perimeter of the hexagon ABCDEF is04:44View SolutionA variable line L is drawn through O (0,0) to meet the lines L 1 :y−x−10=0 and L 2 :y−x−20=0 at the points A and B respectively. A point P is taken on L such that OP 2 = OA 1 + OB 1 and P,A,B lies on same side of origin O. The locus of P is
05:21View SolutionA variable line L is drawn through O(0,0) to meet the line L1 and L2 given by y-x-10 =0 and y-x-20=0 at Points A and B, respectively.
Locus of P, if OP2=OA×OB, is a. (y+x)2=50 b. (y−x)2=200 c. (y−x)2=100 d. none of these14:00View SolutionA variable line L si drawn through O(0,0) to meet lines L1andL2 given by y−x−10=0andy−x−20=0 at point A and B, respectively.
A point P IS taken on L such that 2/OP=1/OA+1/OB. Then the locus of P is02:40View SolutionA variable line L si drawn through O(0,0) to meet lines L1andL2 given by y−x−10=0andy−x−20=0 at point A and B, respectively.
Locus of P, if OP2=OA×OB, is02:15View SolutionA variable line L si drawn through O(0,0) to meet lines L1andL2 given by y−x−10=0andy−x−20=0 at point A and B, respectively.
Locus of P if (1/OP2)=(1/OA2), is02:42View SolutionA variable line L drawn through O(0,0) to meet line l1: y-x-10=0 and L2:y-x-20=0 at the point A and B respectively then locus of point p is ' such that (OP)2=OA.OB,
07:11View Solution
