A vertical pole stands at a point A on the boundary of a circular park of radius a and subtends an angle α at another point β on the boundary. If the chord AB subtends an angle α at the centre of the park, the height of the pole is
2a sinα2tanα
2a cosα2tanα
2a sinα2cotα
2a cosα2cotα
The correct Answer is:A
Topper's Solved these Questions
- HEIGHTS AND DISTANCES BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - QUESTIONS FROM PREVIOUS YEARS. AIEEE/JEE MAIN PAPERS
20 Videos
- ELLIPSEBOOK - MCGROW HILL PUBLICATIONCHAPTER - ELLIPSEEXERCISE - Previous Years B-Architecture Entrance Examination Papers
6 Videos
- HYPERBOLABOOK - MCGROW HILL PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - QUESTION FROM PREVIOUS YEARS. B-ARCHITECTURE ENTRANCE EXAMINATION PAPERS
8 Videos
Similar Questions
A vertical pole stands at a point O on a horizontal ground. A and B are points on the ground d metres apart. The pole subtends angles αandβ at A and B respectively. AB subtends an angle at O. the height of the pole is ______.
View SolutionA tower stands at the centre of a circular park . A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60∘ at the foot of the tower , and the angle of elevation of the top of the tower from A or B is 30∘. The height of the tower is
View Solution
Knowledge Check
A pole stands at a point A on the boundary of a circular park of radius a and subtends an angle α at another point B on the boundary. If the chord AB subtends an angle α at the centre of the path, the height of the pole is
A2acos(α/2)tanαB2asin(α/2)cotαC2asin(α/2)tanαD2acos(α/2)cotαA vertical pole stands at a point A on the boundary of a circular park of radius 2 km and subtends an angle 60∘ at another point B on the boundary. If the chord AB subtends the same angle 60∘ at the centre of the park, the height of the pole is
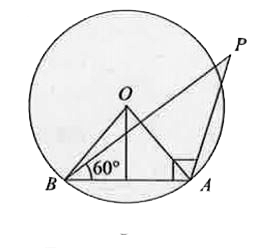 A2√3 kmB√3 kmC2/√3 kmmD1 km
A2√3 kmB√3 kmC2/√3 kmmD1 kmA lamp post standing at a point A on a circular path of radius r subtends an angle α at some point B on the path, and AB subtends an angle of 45∘ at any other point on the path, then height of the lampost is
A√2rcotαB(r/√2)tanαC√2rtanαD(r/√2)cotα
The area of segment of a circle of radius α subtending an angle of 2α at the centre is :
View SolutionThe area of segment of a circle of radius α subtending an angle of 2α at the centre is :
View SolutionA tower stands at the centre of a circular park. A and B are two points on the boundary of the park such that AB(=alpha) subtends an angle of elevation of the top of the first tower from A or B is 30∘. The height of the tower is
View SolutionA lamppost stands in the centre of a circular garden and makes angle α at point A and B on the boundary where AB subtends an angle 2β at the foot of the lamppost. If γ is the angle which the lamppost subtends at C, the middle point of the line joining A and B, then tanγ =
View SolutionA tower stands at the centre of a circular park . A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60∘ at the foot of the tower , and the angle of elevation of the top of the tower from A or B is 30∘. The height of the tower is
View Solution
