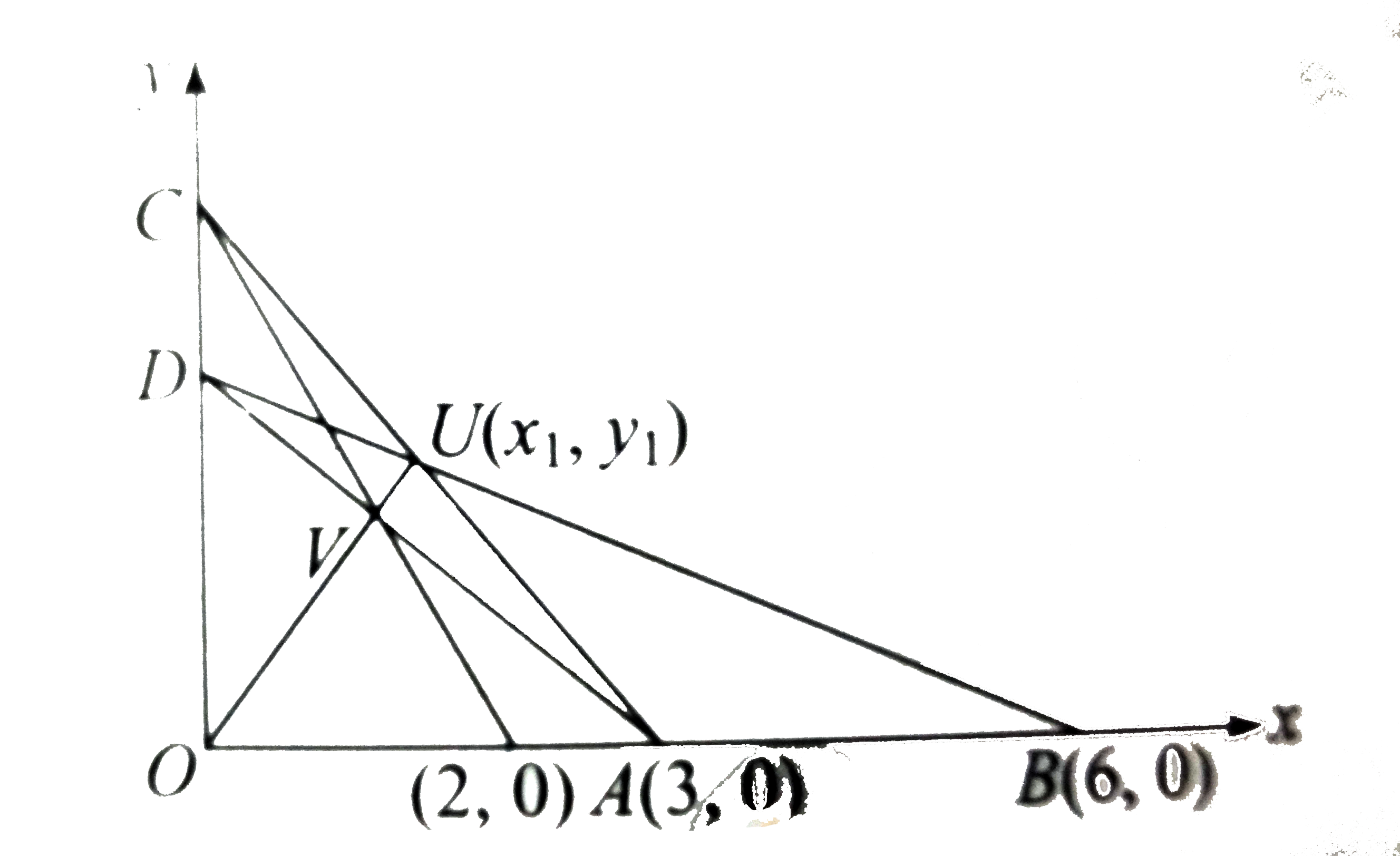
A(3,0) and B(6,0) are two fixed points and U( $x_{1}, y_{1}$ ) is a variable point of the plane .AU and BU meets the y axis at C and D respectively and AD meets OU at V. Then for any position of U in the plane CV passes through fixed point (p,q) whose distance from origin is____units

A

1units

B

2 units

C

3 units

D

4 units

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Equation of AU is
$y - y_{1} = \frac{0 - y_{1}}{3 - x_{1}} (x - x_{1})$
So that the coordinates of C are $(0, 3 y_{1} / (3 - x_{1}))$
Similarly, the coordinates of D are $(0, 6 y_{1} / (6 - x_{1}))$
Now, equation of AD is $\frac{x}{3} + \frac{y (6 - x_{1})}{6 y_{1}} = 1$ (i)
and equation of OU is $y_{1} x = x_{1} y$ (ii)

Solving (i) and (ii), we get
$\frac{x_{1} y}{3 y_{1}} + \frac{y (6 - x_{1})}{6 y_{1}} = 1$
$\Rightarrow y (2 x_{1} + 6 - x_{1}) = 6 y_{1}$
$\Rightarrow y = \frac{6 y_{1}}{6 + x_{1}} \Rightarrow x = \frac{6 x_{1}}{6 + x_{1}}$
Hence, the coordinates of V are $(\frac{6 x_{1}}{6 + x_{1}}, \frac{6 y_{1}}{6 + x_{1}})$
Therefore, equation of CV is $y - \frac{3 y_{1}}{3 - x_{1}} = \frac{\frac{6 y_{1}}{6 + x_{1}} - \frac{3 y_{1}}{3 - x_{1}}}{\frac{6 x_{1}}{6 + x_{1}} - 0} (x - 0)$
$\Rightarrow y = \frac{3 y_{1}}{3 - x_{1}} - \frac{9 x_{1} y_{1}}{6 x_{1} (3 - x_{1})} x \Rightarrow y = \frac{3 y_{1}}{3 - x_{1}} (1 - \frac{x}{2})$
which passes through the point (2,0) for all values of $(x_{1}, y_{1})$

|

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
An equation of the plane through the points (1,0,0), (0,2,0) and at a distance $6 / 7$ units from the origin, is
A $6 x + 3 y + z - 6 = 0$
B $6 x + 3 y + 2 z - 6 = 0$
C $6 x + 3 y + z + 6 = 0$
D $6 x + 3 y + 2 z + 6 + 0$
Question 2 - Select One
A straight line through the point (1,1) meets the x-axis at 'A' and the y axis at 'B'. The locus of the mid point of AB is :
A2xy + x + y = 0
Bx + y - 2xy = 0
Cx + y + 2 = 0
Dx + y - 2 = 0
Question 3 - Select One
Two point charges $+ q$ and $- q$ are held fixed at $(- d, o)$ and $(d, 0)$ respectively of a x-y coordinate system. Then
A(a) The electric field E at all points on the x-axis has the same direction
B(b) Electric field at all points on y-axis is along x-axis
C(c) Work has to be done in bringing a test charge from $\infty$ to the origin
D(d) The dipole moment is $2 q d$ along the x-axis

CENGAGE-STRAIGHT LINE-Multiple Correct Answers Type

Home
Ask Doubt
Profile