AB is a chord of x2+y2=4 and P(1, 1) trisects AB. Then the length of the chord AB is (a) 1.5 units (c) 2.5 units (b) 2 units (d) 3 units Solution in Bengali
1.5 units
2 units
2.5 units
3 units
The correct Answer is:D
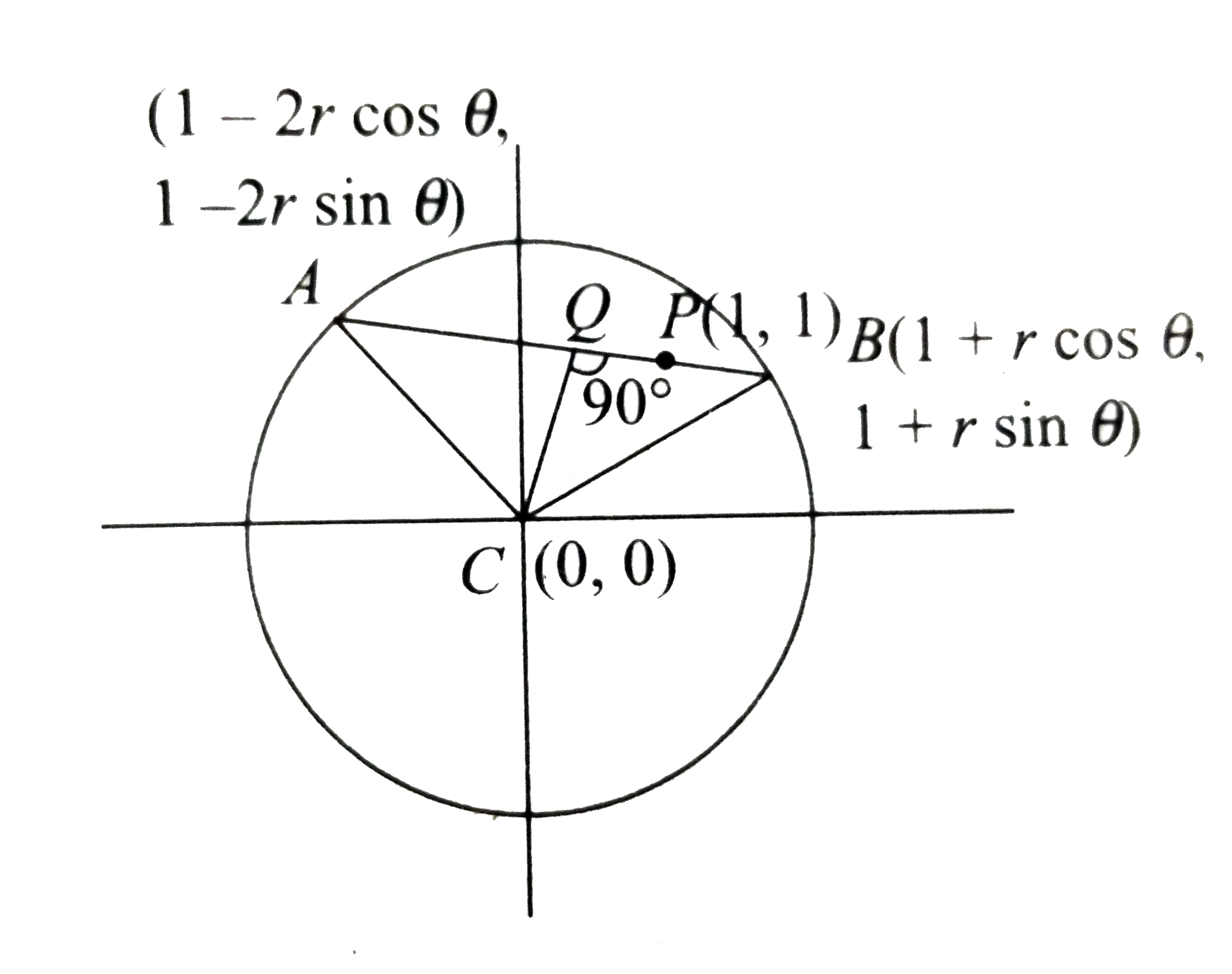
Given in AP:AB=2:1
Let AP=2r and BP=r
Using parametric equations
A=(1−2rcosθ,1−2rsinθ)
B=(1+rcosθ,1+rsinθ)
A lies on the circle x2+y2=4
⇒(1−2rcosθ)2+(1−2rsinθ)2=4 (1)
B lies on the circle x2+y2=4
⇒(1+rcosθ)2+(1+rsinθ)2=4 (2)
Solving (1) and (2), we get r=1
⇒AB=√(3rcosθ)2+(3rsinθ)2=3r=3
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Multiple Correct Answers Type9videos
CIRCLES
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - For problems 3 and 42videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - MULTIPLE CORRECT ANSWER TYPE6videos
COMPLEX NUMBERS
Similar Questions
The line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
05:28View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is05:23View SolutionPARAGRAPH X Let S be the circle in the xy -plane defined by the equation x2+y2=4. (For Ques. No 15 and 16) Let E1E2 and F1F2 be the chords of S passing through the point P0(1, 1) and parallel to the x-axis and the y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope −1 . Let the tangents to S at E1 and E2 meet at E3 , the tangents to S at F1 and F2 meet at F3 , and the tangents to S at G1 and G2 meet at G3 . Then, the points E3, F3 and G3 lie on the curve x+y=4 (b) (x−4)2+(y−4)2=16 (c) (x−4)(y−4)=4 (d) xy=4
02:43View SolutionLet z1 and z2 be two distinct complex numbers and z=(1−t)z1+tz2, for some real number t with 0<t<1 and i=√−1. If arg(w) denotes the principal argument of a non-zero compolex number w, then
01:45View SolutionAB is a chord of x^(2)+y^(2)=4 and P(1,1) trisects AB.Then the length of the chord AB is (a) 1.5 units (c)2.5 units (b)2 units (d) 3 units
01:48View SolutionLet AB be the chord 4x-3y+5=0 of the circle x^2+y^2-2x+4y-20 = 0.If C =(7, 1) then the area of triangle ABC is: (A) 15 sq unit (B) 20 sq unit (C) 24 sq unit (D) 45 sq unit
View SolutionThe middle point of A (1, 2) and B (x, Y) is C (2, 4). If BD is perpendicular to AB such that CD=3 unit, then what is the length BD ?
View SolutionFrom a point A(1, 1) on the circle x2+y2−4x−4y+6=0 two equal chords AB and AC of length 2 units are drawn. The equation of chord BC, is
04:26View SolutionLet AB is the focal chord of a parabola and D and C be the foot of the perpendiculars from A and B on its directrix respectively. If CD = 6 units and area of trapezium ABCD is 36 square units, then the length (in units) of the chord AB is
02:44View SolutionIf a, b and c are unit vectors then |a−b|2+|b−c|2+|c−a|2 does not exceed.
05:45View SolutionIf a, b, c and d are unit vectors, then |a−b|2+|b−c|2+|c−d|2+|d−a|2+|c−a|2+|b−d|2 does not exceed
02:56View SolutionAB is a chord of x2+y2=4 and P(1, 1) trisects AB. Then the length of the chord AB is (a) 1.5 units (c) 2.5 units (b) 2 units (d) 3 units
02:48View SolutionIn triangle ABC, ∠ABC=90∘, AB= c unit, BC= a unit, AC=b unit, CD is perpendicular to AB and CD= p unit.
Prove that : 1p2=1a2+1b2
 02:49View Solution
02:49View SolutionFrom a point A(1, 1) on the circle x2+y2−4x−4y+6=0 two equal chords AB and AC of length 2 units are drawn. The equation of chord BC, is
05:15View SolutionLet AB is the focal chord of a parabola and D and C be the foot of the perpendiculars from A and B on its directrix respectively. If CD = 6 units and area of trapezium ABCD is 36 square units, then the length (in units) of the chord AB is
04:18View Solution

