ABC is a right-angled triangle in which ∠A=90∘and AB=AC. Find ∠Band∠C.
To solve the problem, we need to find the measures of angles B and C in triangle ABC, where angle A is 90 degrees and sides AB and AC are equal.
1. Identify the Given Information:
- Triangle ABC is a right-angled triangle.
- Angle A = 90°.
- Sides AB = AC.
2. Use the Property of Isosceles Triangles:
- Since AB = AC, triangle ABC is an isosceles triangle.
- In an isosceles triangle, the angles opposite the equal sides are also equal.
- Therefore, angle B = angle C.
3. Apply the Angle Sum Property:
- The sum of the angles in any triangle is 180°.
- Thus, we can write the equation:
Angle A+Angle B+Angle C=180°
- Substituting the known value of angle A:
90°+Angle B+Angle C=180°
4. Substitute Angle B for Angle C:
- Since angle B = angle C, we can substitute:
90°+Angle B+Angle B=180°
- This simplifies to:
90°+2⋅Angle B=180°
5. Solve for Angle B:
- Rearranging the equation gives:
2⋅Angle B=180°−90°
2⋅Angle B=90°
- Dividing both sides by 2:
Angle B=90°2=45°
6. Find Angle C:
- Since angle B = angle C, we have:
Angle C=45°
Conclusion:
- Angle B = 45°
- Angle C = 45°
- Triangle ABC is a right-angled triangle.
- Angle A = 90°.
- Sides AB = AC.
- Since AB = AC, triangle ABC is an isosceles triangle.
- In an isosceles triangle, the angles opposite the equal sides are also equal.
- Therefore, angle B = angle C.
- The sum of the angles in any triangle is 180°.
- Thus, we can write the equation:
Angle A+Angle B+Angle C=180°
- Substituting the known value of angle A:
90°+Angle B+Angle C=180°
- Since angle B = angle C, we can substitute:
90°+Angle B+Angle B=180°
- This simplifies to:
90°+2⋅Angle B=180°
- Rearranging the equation gives:
2⋅Angle B=180°−90°
2⋅Angle B=90°
- Dividing both sides by 2:
Angle B=90°2=45°
- Since angle B = angle C, we have:
Angle C=45°
- Angle B = 45°
- Angle C = 45°
Similar Questions
Knowledge Check
- Question 1 - Select One
ABC is right angled triangle in which ∠A=90∘, AB= 5 cm and AC= 12 cm. What is the approximate volume (in cm3) of the double cone formed by rotating the triangle about its hypotenuse?
A145B290C435D580 - Question 2 - Select One
△ABC is an isosceles right triangle in which ∠A=90∘ and BC = 6 cm. Then AB = ?
A2√2cmB3√2cmC4√2cmD2√3cm
ABC is a right angled triangle in which ∠B=90∘andAB=BC. Find ∠Aand∠C.
View SolutionIn ABC, ∠A=1000 and AB=AC. Find ∠B and ∠C.
View SolutionIn ΔABC,∠A=1000 and AB=AC. Find ∠B and ∠C
View SolutionIn a ABC, if ∠A=1200and AB=AC. Find ∠B and ∠C
View SolutionIn the adjoining figure, ΔABC is a right-angled triangle in which ∠B=90∘, ∠A=30∘ and AC=20cm. Find (i)BC, (ii)AB.
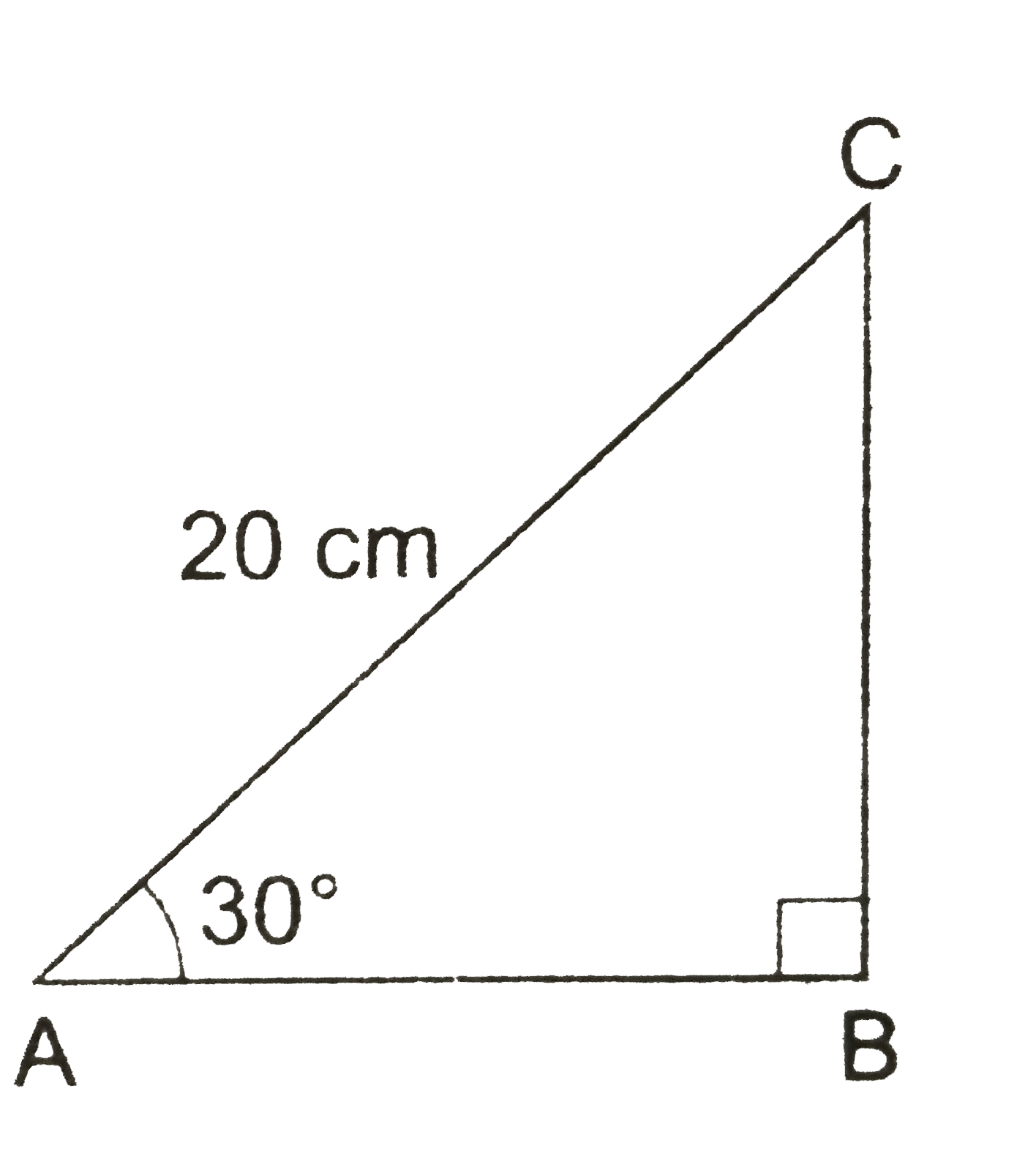 View Solution
View SolutionIn fig ABC is a right angled triangle in which ∠A=90∘, AB = 21 cm and AC=28cm Semi-circles are described on AB, BC and AC as diameters. Find the area of the shaded region
View Solution

