ABC is a right-angled triangle is which ∠A=900and AB=AC. Find ∠B and ∠C
To solve the problem, we need to find the angles B and C in the right-angled triangle ABC, where ∠A=90∘ and AB=AC.
1. Identify the Given Information:
- We have a right-angled triangle ABC with ∠A=90∘.
- The lengths of sides AB and AC are equal, i.e., AB=AC.
Hint: Write down what you know about the triangle and the properties of right-angled triangles.
2. Use the Property of Isosceles Triangles:
- Since AB=AC, triangle ABC is isosceles with respect to sides AB and AC.
- In an isosceles triangle, the angles opposite the equal sides are also equal. Thus, ∠B=∠C.
Hint: Recall the property of angles in isosceles triangles.
3. Use the Angle Sum Property:
- The sum of angles in any triangle is 180∘.
- Therefore, we can write the equation:
∠A+∠B+∠C=180∘
- Substituting ∠A=90∘:
90∘+∠B+∠C=180∘
Hint: Remember the sum of angles in a triangle.
4. Simplify the Equation:
- Rearranging the equation gives:
∠B+∠C=180∘−90∘=90∘
- Since ∠B=∠C, we can substitute ∠C with ∠B:
∠B+∠B=90∘
- This simplifies to:
2∠B=90∘
Hint: Use algebraic manipulation to isolate the variable.
5. Solve for Angle B:
- Dividing both sides by 2 gives:
∠B=90∘2=45∘
Hint: Perform the division carefully to find the measure of angle B.
6. Find Angle C:
- Since ∠B=∠C, we have:
∠C=45∘
Hint: Use the equality of angles derived from the isosceles property.
Final Answer:
- ∠B=45∘
- ∠C=45∘
- We have a right-angled triangle ABC with ∠A=90∘.
- The lengths of sides AB and AC are equal, i.e., AB=AC.
- Since AB=AC, triangle ABC is isosceles with respect to sides AB and AC.
- In an isosceles triangle, the angles opposite the equal sides are also equal. Thus, ∠B=∠C.
- The sum of angles in any triangle is 180∘.
- Therefore, we can write the equation:
∠A+∠B+∠C=180∘
- Substituting ∠A=90∘:
90∘+∠B+∠C=180∘
- Rearranging the equation gives:
∠B+∠C=180∘−90∘=90∘
- Since ∠B=∠C, we can substitute ∠C with ∠B:
∠B+∠B=90∘
- This simplifies to:
2∠B=90∘
- Dividing both sides by 2 gives:
∠B=90∘2=45∘
- Since ∠B=∠C, we have:
∠C=45∘
- ∠B=45∘
- ∠C=45∘
Topper's Solved these Questions
Similar Questions
ABC is a right-angled triangle is which ∠A=900and AB=AC. Find ∠B and ∠C
View SolutionABC is a right-angled triangle in which ∠A=90∘and AB=AC. Find ∠Band∠C.
View SolutionABC is a right angled triangle in which ∠A=900and AB=AC. Find ∠B and ∠C
View SolutionABC is a right angled triangle in which ∠A=90∘ and AB =AC . Find ∠Band∠C.
View SolutionIf △ABC is a right triangle such that ∠C=90∘ , ∠A=45∘ and BC=7 units. Find ∠B, AB and AC .
View SolutionIn a right angled triangle ABC ,if ∠A=90∘, sinB=45, then cosC =
View SolutionIn a right angled triangle ABC ,if ∠A=90∘, sinB=45, then cosC =
View SolutionABCD is a cyclic quadrilateral in which: BCAD, ∠ADC=1100 and ∠BAC=500. Find ∠DAC ∠DBC=800 and ∠BAC=400. Find ∠BCD ∠BCD=1000and ∠ABD=700 find ∠ADB
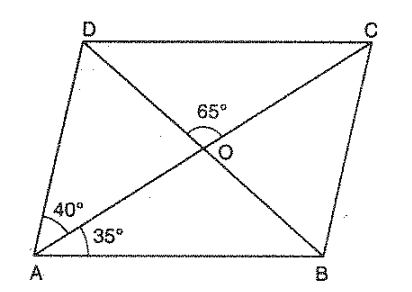
View SolutionIn Figure, ABCD is a parallelogram in which ∠DAO=400, ∠BAO=350 and ∠COD=650. Find: ∠ABO (ii) ∠ODC (iii) ∠ACB (iv) ∠CBD
View SolutionIf ABC and DEF are two triangles such that ABC ≅FDE and AB=5cm, ∠B=400 and ∠A=800. Then, which of the following is true? DF=5cm, ∠F=600 (b) DE=5cm, ∠E=600 DF=5 cm, ∠E=600 (d) DE=5cm, ∠D=400
View Solution
