ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD⊥AC (iii) CM=MA=12AB

(i)D is the mid-point of AC
In△ABC
Given : M is the mid-point of AB
& MD || BC
∴D is the mid-point of AC(Converse of mid-point theorem)
(ii) MD⊥AC
DM∣∣CB, AC is the transversal
∠MDC+∠DCB=1800(Co-interior angles)
∠MDC+900=1800
∠MDC=1800−900
∠MDC=900
∴MD⊥AC
(iii)CM=MA=12AB
=>Join MC
In △AMDand△CMD,
AD=CD (D is the mid-point of side AC)
∠ADM=∠CDM(900)
DM=DM (Common)
∴△AMD≅△CMD(By SAS congruence rule)
AM=CM (By CPCT)
AM=12AB (M is the mid-point of AB)
∴CM=AM=12AB
Topper's Solved these Questions
Similar Questions
ABC is a triangle right-angled at C. A line through the midpoint P of hypotenuse AB and parallel to BC intersects AC at M. Show that
(i) M is the midpoint of AC,
(ii) MP⊥AC,
(iii) CP=AP=12AB.View SolutionΔABC is a right triangle right angled at A such that AB=AC and bisector of ∠C intersects the side AB at D. Prove that AC+AD=BC.
View Solution
Knowledge Check
- Question 1 - Select One
If ABC is a right angled triangle at B and M, N are the mid-points of AB and BC, then 4(AN2+CM2) is equal to :
A4AC2B6AC2C5AC2D54AC2 - Question 2 - Select One
Let D be the mid-point of a straight line AB and let C be a point different from D such that AC=BC, then.
AAC⊥ABB∠BDC=90∘C∠BDC is acuteD∠BNDC>90∘ - Question 3 - Select One
In the figure, ABC is a triangle in which AB=AC. A circle through B touches AC at D and intersects AB at P. If D is the mid - point of AC, Find the value of AB :
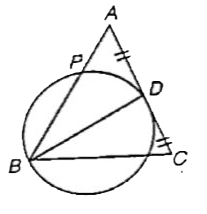 A2APB3APC4APD4√2cm
A2APB3APC4APD4√2cm
In Fig. 3, ABC is a right triangle, right angled at C and D is the mid-point of BC. Prove that AB2=4AD2−3AC2.
View SolutionABC is a triangle right angled at B. D is a point on AC such that ∠ABD=45∘ If AC=6 and AD=2 then AB=
View Solution15m,BC=8 2. ABC is a triangle, right-angled at B. If AB =15 m, BC = 8 m, find AC.
View SolutionIn right triangle ABC , right angle at C, M is the mid-point of the hypotenuse AB. C is jointed to M and produced to a point D such that DM=CM. Point D is joined to point B. Show that AMC≅BMD (ii) ∠DBC=90∘ (iii)DBC≅ACB (iv) CM=12AB
View SolutionIf D is the mid-point of the hypotenuse AC of a right triangle ABC, prove that BD=12AC
View Solution

