The line segment joining the mid-points of any two sides of a triangle in parallel to the third side and equal to half of it.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

ocr_image
Given−DandE are midpoint of AB and AC
To prove:DE//BCandDE=12BC
construct CX//ABsuch that extended DE meet at F
proof:In△ADEand△CEF
∠A=∠C→(alternate interior angles)
AED=∠CEF→(vertically opposite angles)
AE=CE→(given)
∴△ADE≅△CEF−.(angle-side-angle congruency rule )
DE=EF->"(by C.P.C.T) AD=CF->"(by C.P.C.T)"
∴BD//CF
AD=BDandAD=CF
∴BD=CF
⇒BDFC is a parallelogram because opposite sides are equal and parallel
DF//BC
=>DE//BC DF=BCandDE=EF
⇒DE=12BC
Hence proved

Updated on:21/07/2023
|
Class 9MATHSQUADRILATERALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Assertion : ABCD and PQRC are rectangles and Q is a midpoint of AC. Then DP = PC.
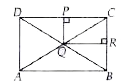
    ocr_image
    Reason : The line segment joining the midpoints of any two sides of a triangle is parallel to the third side and equal to half of it.

    AIf both assertion and reason are true and reason is the correct explanation of assertion
    BIf both assertion and reason are true but reason is not the correct explanation of assertion.
    CIf assertion is true but reason is false.
    DIf assertion is false but reason is true.

  • The line segment joining the midpoints of any two sides of a triangle is parallel to the third side and equal to half of it.
    In the given figure, the side AC of ΔABC is produced to E such that CE = FAC. If D is the midpoint of BC and ED produced meets AB at F and CP, DQ are drawn parallel to BA, then FD=
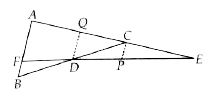    ocr_image

    A12FE
    B13EF
    C2FE
    DFE

  • Assertion : If a line divides any two sides of a triangle in the same ratio , then the line is paralle to third side .
    Reason : Line segment joining the mid -point of any two sides of a triangle is parallel to the third side .

    AIf both assertion and reason are true and reason is the correct explanation of assertion .
    BIf both assertion and reason are true but reason is not the correct explanation of assertion .
    CIf assertion is true reason is false .
    DIf assertion is false but reason is true .