ABC is a triangle right-angled at C. A line through the midpoint P of hypotenuse AB and parallel to BC intersects AC at M. Show that
$(i)$ M is the midpoint of $A C,$
$(ii) M P ⊥ A C,$
$(iii) C P = A P = \frac{1}{2} A B .$

Video Solution

Text Solution

Verified by Experts

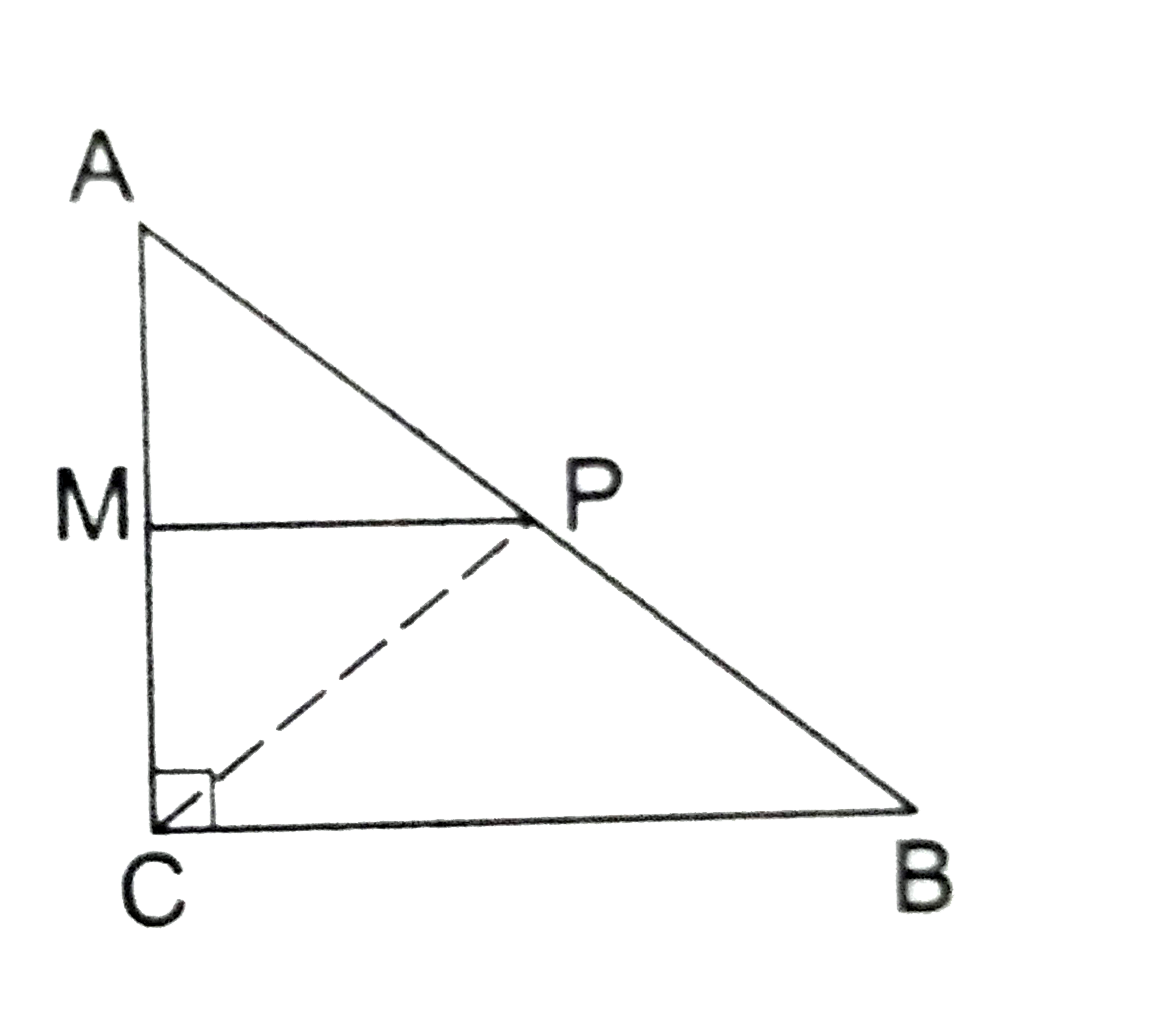
In $△ A C B$ , we have
P is the midpoint of AB and PM||BC.
$∴$ M is the midpoint of AC.
[by converse of midpoint theorem].
Now, PM||BC.
$∴ ∠ P M C + ∠ B C M = 180^{\circ} (co-interior ∠)$
$\Rightarrow ∠ P M C + 90^{\circ} = 180^{\circ} \Rightarrow ∠ P M C = 90^{\circ} .$
Thus, $M P ⊥ A C .$
Join PC.
In $△ P M A$ and PMC, we have:
$M A = M C [∵ M is the midpoint of AC]$
$∠ P M A = ∠ P M C (each equal to 90^{\circ})$
$P M = P M$ (common)
$∴ △ P M A ≅ △ P M C$ (SAS-criterion).
And so, $A P = C P$ (c.p.c.t.).
Now, P is the midpoint of AB.
$∴ C P = A P = \frac{1}{2} A B .$

Updated on:21/07/2023

ABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then, AP : AB is
A $3 : 5$
B $1 : 4$
C $4 : 1$
D $2 : 3$
ABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point Intersects AB at P. Then AP: AB is :
A $4 : 1$
B $2 : 3$
C $3 : 5$
D $1 : 4$
ABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then AP : AB is :
A $4 : 1$
B $2 : 3$
C $3 : 5$
D $1 : 4$