ABC is an isosceles right triangle, right-angled at C . Prove that: AB2=2AC2.
Step by step video & image solution for ABC is an isosceles right triangle, right-angled at C . Prove that: A B^2=2A C^2dot by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ABC is an isosceles right triangle right-angled at C . Prove that AB2=2AC2 .
View SolutionABC is a isosceles right angled triangle, right angled at C. prove that AB2=2AC2
View SolutionIn figure ABC is an isosceles triangle , right angled at C . Therefore

View SolutionABC is an isosceles right-triangle right angled at C such that AC=5 cm. Then, AB= (a) 2.5 cm (b) 5√2 cm (c) 10 cm (d) 5 cm
View SolutionABC is a right triangle right-angled at CandAC=√3BC . Prove that ∠ABC=600.
View SolutionD and E are points on the sides CA and CB respectively of a triangle ABC right angled at C. Prove that AE2+BD2=AB2+DE2 .
View SolutionD and E are points on the sides CA and CB respectively of a triangle ABC right angled at C. Prove that AE2+BD2=AB2+DE2.
View SolutionABC is a triangle, right angled at B, M is a point on BC. Prove that :
AM2+BC2=AC2+BM2
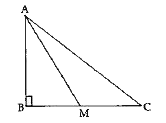
View SolutionBL and CM are medians of a triangle ABC right angled at A. Prove that 4(BL2+CM2)=5BC2
View SolutionLet the complex numbers Z1,Z2andZ3 are the vertices A, B and C respectively of an isosceles right - angled triangle ABC with right angle at C, then the value of (Z1−Z2)2(Z1−Z3)(Z3−Z2) is equal to
View Solution
