ABCD is a regular tetrahedron, A is the origin and B lies on x-axis. ABC lies in the xy-plane ∣∣∣−−→AB∣∣∣=2 Under these conditions, the number of possible tetrahedrons is :
The correct Answer is:8
To solve the problem, we need to analyze the conditions given for the regular tetrahedron ABCD. Let's break it down step by step.
Step 1: Understand the Position of Points
- Point A is the origin, so A(0,0,0).
- Point B lies on the x-axis, which means it can be represented as B(b,0,0).
- The length of vector →AB is given as |→AB|=2. Therefore, |b|=2, which gives us two possible coordinates for B: B(2,0,0) or B(−2,0,0).
Step 2: Determine the Position of Point C
- The points A, B, and C lie in the xy-plane. Hence, point C can be represented as C(0,c,0), where c can take positive or negative values.
- Since ABC is a triangle in the xy-plane, point C can be either above or below the x-axis, leading to two possible values for C: C(0,c,0) where c can be +y or −y.
Step 3: Determine the Position of Point D
- Point D is the apex of the tetrahedron and must be above or below the plane formed by triangle ABC. Therefore, point D can be represented as D(0,0,d), where d can also take positive or negative values.
- This gives us two possible values for D: D(0,0,+z) or D(0,0,−z).
Step 4: Count the Combinations
1. For B(2,0,0):
- C can be (0,+c,0) or (0,−c,0) → 2 options.
- D can be (0,0,+d) or (0,0,−d) → 2 options.
- Total combinations for B at (2,0,0) = 2×2=4.
2. For B(−2,0,0):
- C can again be (0,+c,0) or (0,−c,0) → 2 options.
- D can be (0,0,+d) or (0,0,−d) → 2 options.
- Total combinations for B at (−2,0,0) = 2×2=4.
Step 5: Calculate Total Tetrahedrons
- Total number of tetrahedrons = Combinations for B(2,0,0) + Combinations for B(−2,0,0) = 4+4=8.
Final Answer
The total number of possible tetrahedrons is 8.
---
- Point A is the origin, so A(0,0,0).
- Point B lies on the x-axis, which means it can be represented as B(b,0,0).
- The length of vector →AB is given as |→AB|=2. Therefore, |b|=2, which gives us two possible coordinates for B: B(2,0,0) or B(−2,0,0).
- The points A, B, and C lie in the xy-plane. Hence, point C can be represented as C(0,c,0), where c can take positive or negative values.
- Since ABC is a triangle in the xy-plane, point C can be either above or below the x-axis, leading to two possible values for C: C(0,c,0) where c can be +y or −y.
- Point D is the apex of the tetrahedron and must be above or below the plane formed by triangle ABC. Therefore, point D can be represented as D(0,0,d), where d can also take positive or negative values.
- This gives us two possible values for D: D(0,0,+z) or D(0,0,−z).
1. For B(2,0,0):
- C can be (0,+c,0) or (0,−c,0) → 2 options.
- D can be (0,0,+d) or (0,0,−d) → 2 options.
- Total combinations for B at (2,0,0) = 2×2=4.
- C can again be (0,+c,0) or (0,−c,0) → 2 options.
- D can be (0,0,+d) or (0,0,−d) → 2 options.
- Total combinations for B at (−2,0,0) = 2×2=4.
- Total number of tetrahedrons = Combinations for B(2,0,0) + Combinations for B(−2,0,0) = 4+4=8.
The total number of possible tetrahedrons is 8.
Topper's Solved these Questions
- BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - VECTOR & 3DIMENSIONAL GEOMETRYEXERCISE - Exercise-4 : Matching Type Problems2videos
VECTOR & 3DIMENSIONAL GEOMETRY
- BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS EXERCISE - Exercise-5 : Subjective Type Problems9videos
TRIGONOMETRIC EQUATIONS
Similar Questions
ABCD is a regular tetrahedron P & Q are the mid -points of the edges AC and AB respectively, G is the cenroid of the face BCD and θ is the angle between the vectors −−→PG and −−→DQ, then
View SolutionABCD is square in which A lies on positive y-axis and B lies on the positive x-axis. If D is the point (12, 17), then co-ordinate of C is
View SolutionIf O be the origin and OP=r and OP makes an angle θ with the positive direction of x-axis and lies in the XY plane find the coordinates of P.
View SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The value of [→a→b→c]2 isView SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The angle between any edge and a face not containing the edge isView SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The angle between any two faces isView SolutionA triangle has two of its vertices at (0,1) and (2,2) in the cartesian plane. Its third vertex lies on the x-axis. If the area of the triangle is 2 square units then the sum of the possible abscissae of the third vertex, is-
View SolutionA circular hole of radius R/2 is cut from a circular disc of radius R. The disc lies in the xy-plane and its centre coincides with the origin. If the remaining mass of the disc is M, then
a. determine the initial mass of the disc and
b. determine its moment of inertia about the z-axis.
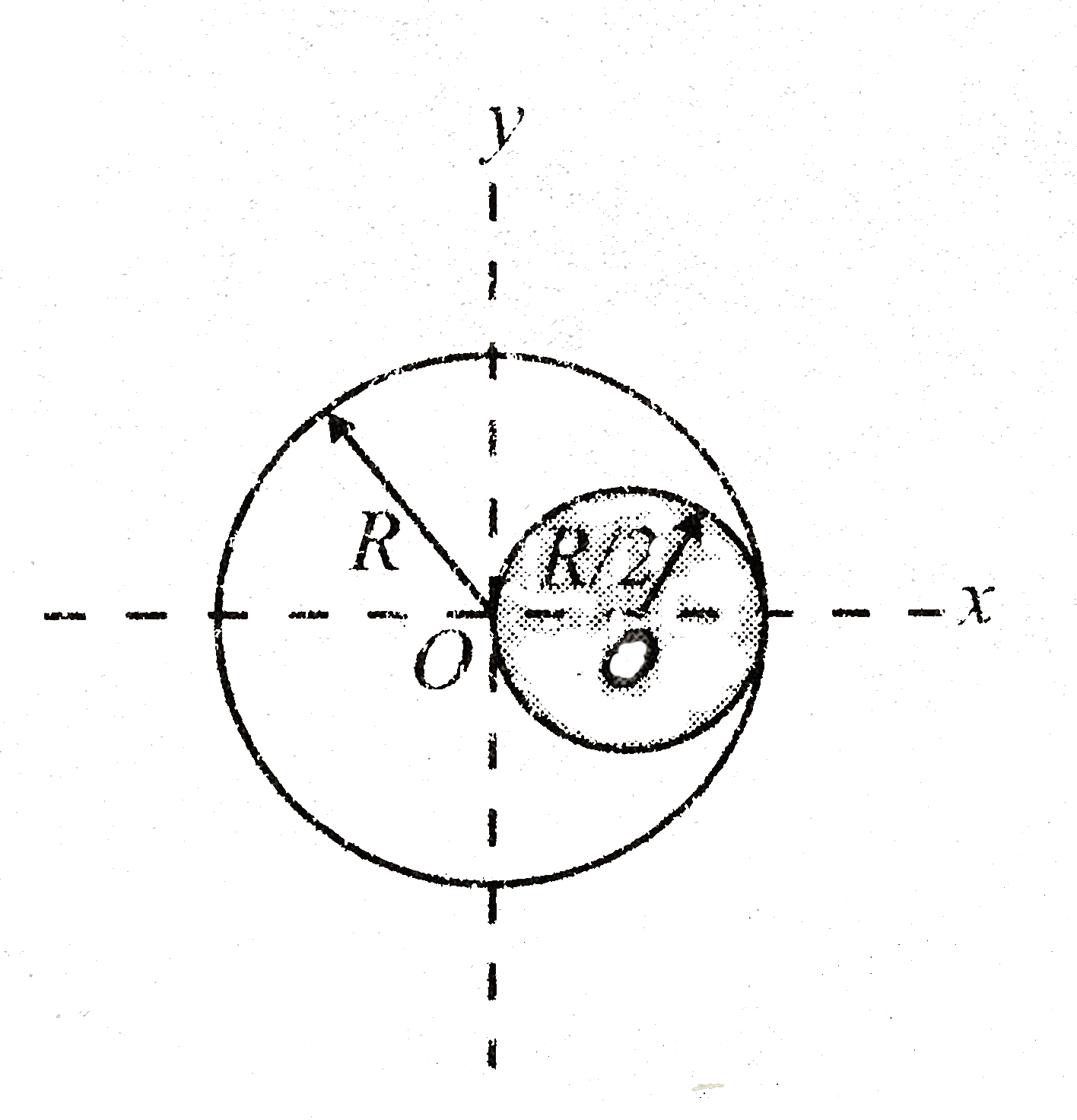 View Solution
View SolutionA circle is drawn whose centre is on the x - axis and it touches the y - axis. If no part of the circle lies outside the parabola y2=8x, then the maximum possible radius of the circle is
View SolutionOABCDE is a regular hexagon of side 2 units in the XY-plane in the first quadrant. O being the origin and OA taken along the x-axis. A point P is taken on a line parallel to the z-axis through the centre of the hexagon at a distance of 3 unit from O in the positive Z direction. Then find vector AP.
View Solution

