ABCD is a square with sides 12 cm each. Find its perimeter and area. Cut off a rectangle measuring C 8cm 2 cm by 4 cm from a corner as shown in the figure. Find the perimeter and area of the new polygon thus formed.
The correct Answer is:136 sq.cm
To solve the problem step by step, we will first find the perimeter and area of the square ABCD, and then we will calculate the perimeter and area of the new polygon after cutting off the rectangle.
Step 1: Find the Perimeter of Square ABCD
The formula for the perimeter P of a square is given by:
P=4×side
Given that each side of the square ABCD is 12 cm:
P=4×12=48 cm
Step 2: Find the Area of Square ABCD
The formula for the area A of a square is given by:
A=side2
Using the side length of 12 cm:
A=122=144 cm2
Step 3: Find the Area of the Rectangle Cut Off
The dimensions of the rectangle are given as 2 cm by 4 cm. The area Ar of the rectangle can be calculated using the formula:
Ar=length×breadth
Thus,
Ar=2×4=8 cm2
Step 4: Find the Area of the New Polygon
To find the area of the new polygon formed after cutting off the rectangle, we subtract the area of the rectangle from the area of the square:
Anew=A−Ar
Substituting the values we found:
Anew=144−8=136 cm2
Step 5: Find the Perimeter of the New Polygon
To find the perimeter of the new polygon, we need to consider the perimeter of the square and subtract the lengths of the sides of the rectangle that were removed. The rectangle has one side of 4 cm and another side of 2 cm, so we remove these lengths from the perimeter of the square:
Pnew=P−(4+2)
Substituting the values:
Pnew=48−6=42 cm
Summary of Results
- Perimeter of square ABCD: 48 cm
- Area of square ABCD: 144 cm²
- Area of the rectangle cut off: 8 cm²
- Area of the new polygon: 136 cm²
- Perimeter of the new polygon: 42 cm
The formula for the perimeter P of a square is given by:
P=4×side
Given that each side of the square ABCD is 12 cm:
P=4×12=48 cm
The formula for the area A of a square is given by:
A=side2
Using the side length of 12 cm:
A=122=144 cm2
The dimensions of the rectangle are given as 2 cm by 4 cm. The area Ar of the rectangle can be calculated using the formula:
Ar=length×breadth
Thus,
Ar=2×4=8 cm2
To find the area of the new polygon formed after cutting off the rectangle, we subtract the area of the rectangle from the area of the square:
Anew=A−Ar
Substituting the values we found:
Anew=144−8=136 cm2
To find the perimeter of the new polygon, we need to consider the perimeter of the square and subtract the lengths of the sides of the rectangle that were removed. The rectangle has one side of 4 cm and another side of 2 cm, so we remove these lengths from the perimeter of the square:
Pnew=P−(4+2)
Substituting the values:
Pnew=48−6=42 cm
- Perimeter of square ABCD: 48 cm
- Area of square ABCD: 144 cm²
- Area of the rectangle cut off: 8 cm²
- Area of the new polygon: 136 cm²
- Perimeter of the new polygon: 42 cm
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the perimeter and area of a square of side 9 cm .
A=86cm2B=81cm2C=91cm2D=71cm2
Find the perimeter and area of a square whose side measures :
1m 20 cmView SolutionIf one side of a square is 6.5 cm, find its perimeter and area.
View SolutionFind the perimeter and area of a rectangle having :
Length = 1 m 25 cm , Breadth = 8 cmView SolutionFind the perimeter and area of a square whose side measures :
14 cmView SolutionFind the perimeter and area of a rectangle having :
Length = 16 cm , Breadth = 12 cmView SolutionFind the perimeter and area of a square whose side measures :
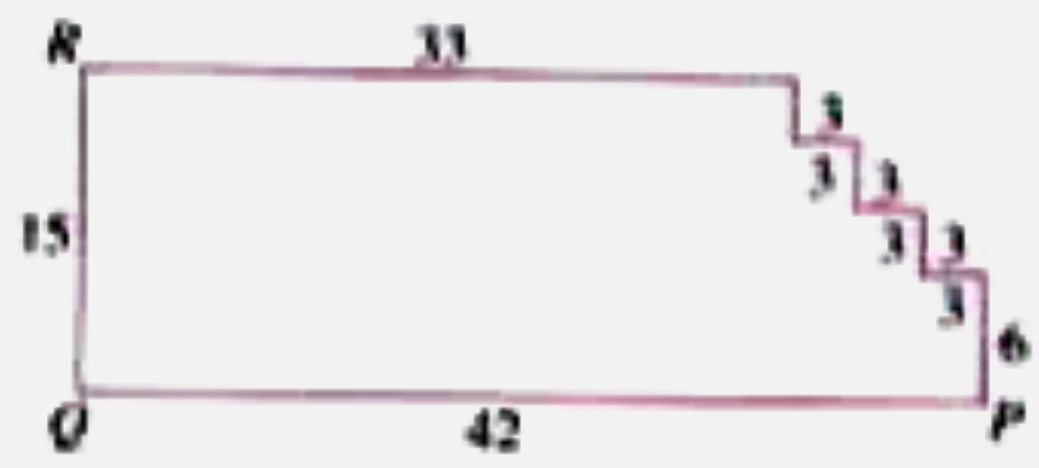
3.5 cmView SolutionPQRS is a rectangle measuring 42 cm by 15 cm. Find its perimeter and area. A portion from one end of the rectangle is cut off as shown in the perimeter and area of the new gigure thus formed.
 View Solution
View Solution
