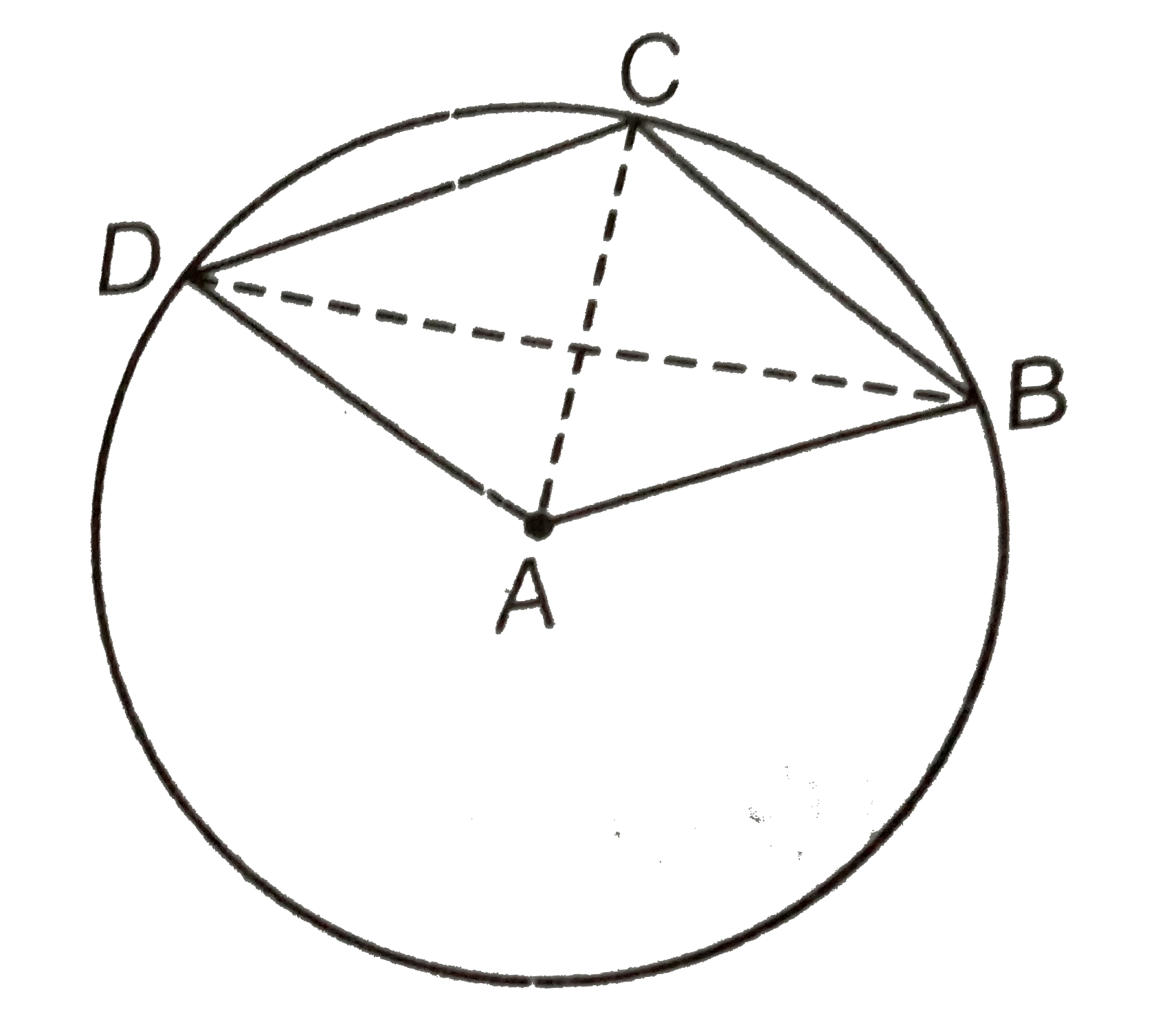
ABCD is such a quadrilateral that A is the centre of the circle passing through B,CandD. Prove that ∠CBD+∠CDB=12∠BAD.
Video Solution
Text Solution
Given In a circle, ABCD is a wuadrilateral having centre A.
To prove ∠CBD+∠CDB=12∠BAD
Construction Join AC and BD.
Proof Since, arc DC subtends ∠DAC at the centre and ∠CBD at a point B in the remaining part of the circle.
∴∠DAC=2∠CBD...(i)
In a circle, the angle subtended by an arc at the centre is twice the angle subtended by it at the remaining part of the circle.
Similarly, arc BC subtends ∠CAB at the centre and ∠CDB at a point D in the remaining part of the circle.
∴∠CAB=2∠CDB....(ii)
In a circle, the angle subtended by an arc at the centre is twice the angle subtended by it at the remaining part of the circle.
On adding Eqs. (i) and (ii), we get
∠DAC+∠CAB=2∠CBD+2∠CDB
⇒∠BAD=2(∠CBD+∠CDB)
⇒∠CDB+∠CBD=12∠BAD

|
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLARCHAPTER - CIRCLESEXERCISE - Exercise 10.4
2 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLARCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Areas Of Parallelograms And Triangles
34 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
Knowledge Check
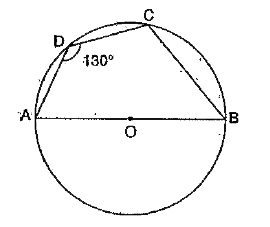
ABCD is a cyclic quadrilateral whose side AB is a diameter of the circle through A, B, C, D. If ∠ADC=130∘, find ∠BAC.
 A40∘B50∘C60∘D30∘
A40∘B50∘C60∘D30∘The area of the circle centred at (1,2) and passing through (4,6), is
A5π sq unitsB10π sq unitsC25π sq unitsDNone of theseThe area of circle centred at (1,2) and passing through (4,6) is
A5πB10πC25πDnone of these
The area of the circle centred at (1,2) and passing through (4,6) is
View SolutionA circle passes through A,B,C and D as shown in the figure. If ∠BAD=63∘, find x.
View SolutionIn a quadrilateral ABCD, CO and DO are the bisectors of ∠C and ∠D respectively. Prove that ∠COD=12(∠A+∠B).
View SolutionIn a quadrilateral ABCD, CO and DO are the bisectors of ∠C and ∠D respectively. Prove that ∠COD=12(∠A+∠B)
View SolutionThe area of circle centred at (1,2) and passing through (4,6) is
View Solution
