An army contingent of 616 members is to march behind an army band of 32 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march ?
To solve the problem of finding the maximum number of columns in which an army contingent of 616 members and an army band of 32 members can march, we need to determine the highest common factor (HCF) of the two numbers.
1. Identify the Numbers:
We have two groups:
- Army contingent: 616 members
- Army band: 32 members
2. Find the Prime Factorization:
- For 616:
- Divide by 2: 616÷2=308
- Divide by 2 again: 308÷2=154
- Divide by 2 again: 154÷2=77
- Now, 77 can be factored into 7 and 11 (both are prime).
- Therefore, the prime factorization of 616 is:
616=23×71×111
- For 32:
- Since 32 is a power of 2, we can express it as:
32=25
3. Determine the HCF:
- The HCF is found by taking the lowest power of all prime factors common to both numbers.
- The only common prime factor is 2.
- The lowest power of 2 in the factorizations is 23.
- Therefore, the HCF is:
HCF=23=8
4. Conclusion:
- The maximum number of columns in which both the army contingent and the army band can march is 8.
Final Answer:
The maximum number of columns in which they can march is 8.
We have two groups:
- Army contingent: 616 members
- Army band: 32 members
- For 616:
- Divide by 2: 616÷2=308
- Divide by 2 again: 308÷2=154
- Divide by 2 again: 154÷2=77
- Now, 77 can be factored into 7 and 11 (both are prime).
- Therefore, the prime factorization of 616 is:
616=23×71×111
- Since 32 is a power of 2, we can express it as:
32=25
- The HCF is found by taking the lowest power of all prime factors common to both numbers.
- The only common prime factor is 2.
- The lowest power of 2 in the factorizations is 23.
- Therefore, the HCF is:
HCF=23=8
- The maximum number of columns in which both the army contingent and the army band can march is 8.
The maximum number of columns in which they can march is 8.
Topper's Solved these Questions
- SAMPLE PAPER 2019BOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION-D
3 Videos
- SAMPLE PAPER 2019BOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION-D 36 (a)
1 Videos
- SAMPLE PAPER 2019BOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION-C 31 (a)
1 Videos
- CBSE BOARDS 2020BOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - CBSE BOARDS 2020EXERCISE - QUESTION
41 Videos
- X BoardsBOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - X BoardsEXERCISE - All Questions
481 Videos
Similar Questions
Any contingent of 616 members is to march behind an army band of 32 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march?
View SolutionCadets are marching in a parade. There are 5 cadets in a row. What is the rule which gives the number of cadets, given the number of rows? (Use n for the number of rows.)
View Solution
Knowledge Check
There are 45 Musicians in an orchestra , and all play two instruments . Of these musicians , 36 play the piano , and 22 play the violin . What is the maximum possible number of orchestra members who play both the piano and the violin ?
A9B13C22D23
Read the bar graph given in Figure and answer the following questions: What is the information given by the bar graph? What is the number of families having 6 members? How many member per family are there in the maximum number of families? Also tell the number of such families. What are the number of members per family for which the number of families are equal? Also, tell the number of such families?
View SolutionA group of 2n students consisting of n boys and n girls are to be arranged in a row such that adjacent members are of opposite sex. The number of ways in which this can be done is
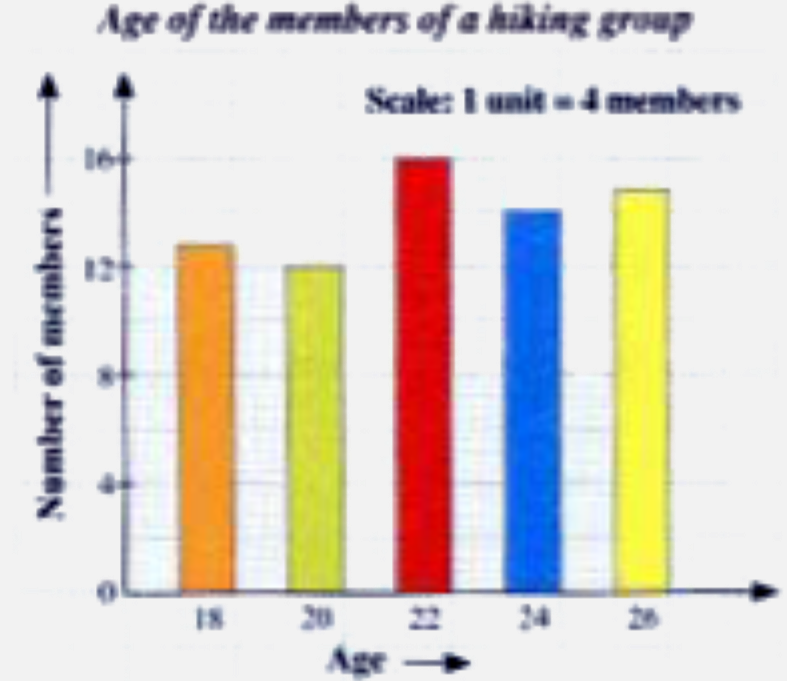
View SolutionThe given graph represents the age and number of members of a hiking group who are planning an excursion. Study the graph and answer the following question.
Which age group has the maximum members in the hiking gruoup?
View SolutionWhich of the following statements related to the modern periodic table is incorrect?
a.The p-block has 6 columns, because a maximum of 6 electrons can occupy all the orbitals in a p-shell.
b.The d-blocks has 8 columns, because a maximum of 8 electrons can occupy all the orbitals in a d-subshell.
c.Each block contains a number of columns equal to the number of electrons that can occupy that subshell.
d.The block indicates value of azimuthal quantum number (l) for the last subshell that received electrons in building up the electronic configuration.
View SolutionIn a high school, a committee has to be formed from a group of 6 boys M1, M2, M3, M4, M5, M6 and 5 girls G1, G2, G3, G4, G5 . Let α1 be the total number of ways in which the committee can be formed such that the committee has 5 members, having exactly 3 boys and 2 girls. (ii) Let α2 be the total number of ways in which the committee can be formed such that the committee has at least 2 members, and having an equal number of boys and girls. (iii) Let α3 be the total number of ways in which the committee can be formed such that the committee has 5 members, at least 2 of them being girls. (iv) Let α4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls and such that both M1 and G1 are NOT in the committee together. LIST-I LIST-II P. The value of α1 is 1. 136 Q. The value of α2 is 2. 189 R. The value of α3 is 3. 192 S. The value of α4 is 4. 200 5. 381 6. 461 The correct option is: P→4; Q→6; R→2; S→1 (b) P→1; Q→4; R→2; S→3 (c) P→4; Q→6; R→5; S→2 (d) P→4; Q→2; R→3; S→1
View SolutionFind the number of ways in which two small squares can be selected on the normal chessboard if they are not in same row or same column.
View SolutionColumn I, Column II Number of straight lines joining any two of 10 points of which four points are collinear, p. 30 Maximum number of points of intersection of 10 straight lines in the plane, q. 60 Maximum number of points of intersection of six circles in the plane, r. 40 Maximum number of points of intersection of six parabolas, s. 45
View Solution
