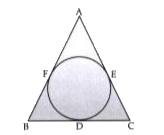
An isosceles triangle ABC, with AB = AC, circumscribes a circle, touching BC at P, AC at Q and AB at R. Prove that the contact point P bisects BC.
To prove that the contact point P bisects BC in the isosceles triangle ABC, where AB = AC and the triangle circumscribes a circle, we can follow these steps:
Step 1: Understand the Triangle and Circle
We have an isosceles triangle ABC with AB = AC. The circle touches the sides of the triangle at points P (on BC), Q (on AC), and R (on AB).
Step 2: Assign Tangent Lengths
Since the circle is tangent to the sides of the triangle, we can denote the lengths of the tangents from points B and C to the points of tangency:
- Let AR = x (tangent from A to R)
- Let BR = y (tangent from B to R)
- Let AQ = x (tangent from A to Q)
- Let QC = z (tangent from C to Q)
- Let BP = y (tangent from B to P)
- Let PC = z (tangent from C to P)
Step 3: Use the Tangent Length Property
Using the property of tangents from a point to a circle, we know:
- From point A: AR = AQ = x
- From point B: BR = BP = y
- From point C: QC = PC = z
Step 4: Write Equations Based on Tangents
From the above, we can write:
1. AB=AR+BR=x+y
2. AC=AQ+QC=x+z
Since AB = AC, we can set the equations equal to each other:
x+y=x+z
Step 5: Simplify the Equation
By simplifying the equation, we can cancel x from both sides:
y=z
Step 6: Conclude that P Bisects BC
Since y = z, we can conclude that:
- BP = y
- PC = z
Thus, we have BP = PC, which means point P bisects segment BC.
Final Conclusion
Therefore, we have proved that the contact point P bisects BC.
We have an isosceles triangle ABC with AB = AC. The circle touches the sides of the triangle at points P (on BC), Q (on AC), and R (on AB).
Since the circle is tangent to the sides of the triangle, we can denote the lengths of the tangents from points B and C to the points of tangency:
- Let AR = x (tangent from A to R)
- Let BR = y (tangent from B to R)
- Let AQ = x (tangent from A to Q)
- Let QC = z (tangent from C to Q)
- Let BP = y (tangent from B to P)
- Let PC = z (tangent from C to P)
Using the property of tangents from a point to a circle, we know:
- From point A: AR = AQ = x
- From point B: BR = BP = y
- From point C: QC = PC = z
From the above, we can write:
1. AB=AR+BR=x+y
2. AC=AQ+QC=x+z
x+y=x+z
By simplifying the equation, we can cancel x from both sides:
y=z
Since y = z, we can conclude that:
- BP = y
- PC = z
Therefore, we have proved that the contact point P bisects BC.
Topper's Solved these Questions
- C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)BOOK - OSWAL PUBLICATIONCHAPTER - C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)EXERCISE - OUTSIDE DELHI SET -III ( SECTION- C )
2 Videos
- C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)BOOK - OSWAL PUBLICATIONCHAPTER - C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)EXERCISE - OUTSIDE DELHI SET -III ( SECTION- D )
2 Videos
- C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)BOOK - OSWAL PUBLICATIONCHAPTER - C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)EXERCISE - OUTSIDE DELHI SET -III ( SECTION- A )
6 Videos
- C.B.S.E 2020 CLASS -X (DELHI)BOOK - OSWAL PUBLICATIONCHAPTER - C.B.S.E 2020 CLASS -X (DELHI)EXERCISE - DELHI SET -III ( SECTION- D )
1 Videos
- CIRCLES BOOK - OSWAL PUBLICATIONCHAPTER - CIRCLES EXERCISE - SELF ASSESSMENT
5 Videos
Similar Questions
Knowledge Check
ABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then, AP : AB is
A3:5B1:4C4:1D2:3ABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then AP : AB is :
A4:1B2:3C3:5D1:4ABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point Intersects AB at P. Then AP: AB is :
A4:1B2:3C3:5D1:4
A triangle ABC is drawn to circumscribe a circle. If AB = 13 cm, BC = 14 cm and AE =7 cm, then find AC.
View SolutionThe bisector of ∠B of an isosceles triangle ABC with AB=AC meets the circumcircle of ABC at P as shown in Figure. If AP and BC produced meet at Q,. prove that CQ=CA
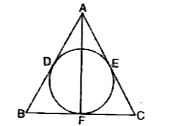
View SolutionThe incircle of an isoceles triangle ABC, with AB=AC, touches the sides AB,BC and CA at D,E and F respecrively. Prove that E bisects BC.
View SolutionIn ΔABC,AB=AC.A circle is drawn with diameter AB and it intersects BC at D. Prove that D is the midpoint of BC.
View SolutionABC is an isosceles triangle (AB = AC) circumscribed about a circle. Then, which of the following statements is correct ?
View Solution
