Any line parallel to the parallel sides of a trapezium divides the non-parallel sides proportionally.
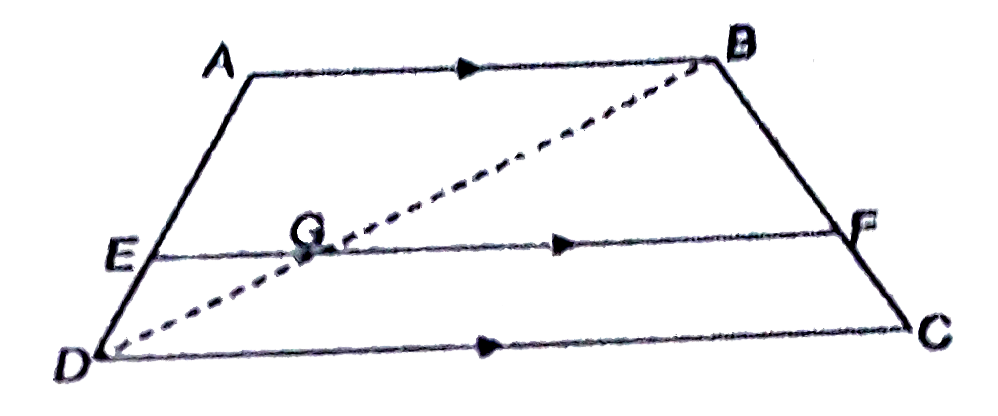
A trapezim ABCD in which AB||DC.
Also a line EF||AB||DC.
To prove , AEED=BGGD
Also in ΔBDC, since GF||DC
BFFC=BGGD ( by B.P theorem)
From (1) and (2) ,we have
AEED=BFFC Hecne proved

Topper's Solved these Questions
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar
14 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6a
23 Videos
- STATISTICS BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
5 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5 Videos
Similar Questions
Consider the following statements :
1. The diagonals of a trapezium divide each other proportionally.
2. Any line drawn parallel to the parallel sides of a trapezium divides the non-parallel sides proportionally.
Which of the above statements is/are correct?
View SolutionThe opposite sides of a trapezium are parallel.
View Solution
Knowledge Check
An isosceles trapezium whose parallel sides are in the ratio 1:4. . If the sum of the two parallel sides is 70 cm,and perpendicular distance between them is 12 cm find the area of trapezium.
A616 cmB420cmC140 cmDnone of theseA trapezium inscribes a circle that touches all its sides and the line connecting the mid-points of the non-parallel sides of the trapezium divides the trapezium in such a way that the area of one part is half the area of the other part. If the lengths of the non-parallel sides be 8 cm and 16 cm, find the length of the shortest side of the trapezium.
A4B6C9Dnone of theseThe parallel sides fo a trapezium are 30 cm and 20 cm and its non-parallel sides are both equal. If the area fo the trapezium is 300cm2, then what is the length (in cm) of eac non-parallel side?
A10B15C12D13
Prove that the segment joining the middle points of two non-parallel sides of a trapezium is parallel to the parallel sides and half of their sum.
View SolutionIf two non-parallel sides of a trapezium are equal; it is cyclic.
View SolutionIf two non-parallel sides of a trapezium are equal; it is cyclic.
View SolutionDraw a trapezium and state the pair of parallel side
View SolutionExamples: Prove that the segment joining the middle points of two non parallel sides of a trapezium is parallel to the parallel sides and half of their sum.
View Solution
